Problem #PRU-5089

Problem

Let’s prove that any ${90}^{\circ }$ angle is equal to any angle larger than ${90}^{\circ }$. On the diagram

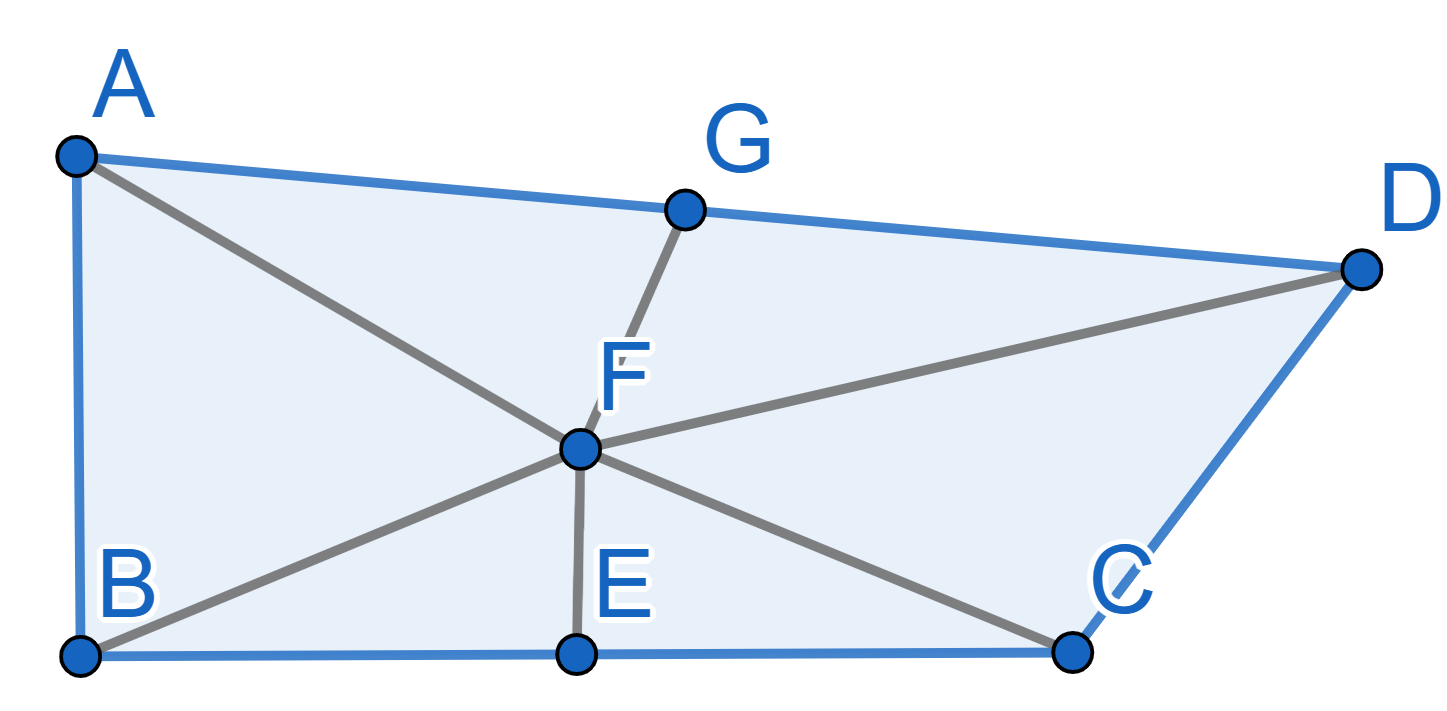
We have the angle $\mathrm{\angle }ABC={90}^{\circ }$ and angle $\mathrm{\angle }BCD>{90}^{\circ }$. We can choose a point $D$ in such a way that the segments $AB$ and $CD$ are equal. Now find middles $E$ and $G$ of the segments $BC$ and $AD$ respectively and draw lines $EF$ and $FG$ perpendicular to $BC$ and $AD$.
Since $EF$ is the middle perpendicular to $BC$ the triangles $BEF$ and $CEF$ are equal which implies the equality of segments $BF$ and $CF$ and of angles $\mathrm{\angle }EBF=\mathrm{\angle }ECF$, the same about the segments $AF=FD$. By condition we have $AB=CD$, thus the triangles $ABF$ and $CDF$ are equal, thus $\mathrm{\angle }ABF=\mathrm{\angle }DCF$. But then we have $$\mathrm{\angle }ABE=\mathrm{\angle }ABF+\mathrm{\angle }FBE=\mathrm{\angle }DCF+\mathrm{\angle }FCE=\mathrm{\angle }DCE.$$