Problems

A maths teacher draws a number of circles on a piece of paper. When she shows this piece of paper to the young mathematician, he claims he can see only five circles. The maths teacher agrees. But when she shows the same piece of paper to another young mathematician, he says that there are exactly eight circles. The teacher confirms that this answer is also correct. How is that possible and how many circles did she originally draw on that piece of paper?

What is the ratio between the red and blue area? All shapes are semicircles.

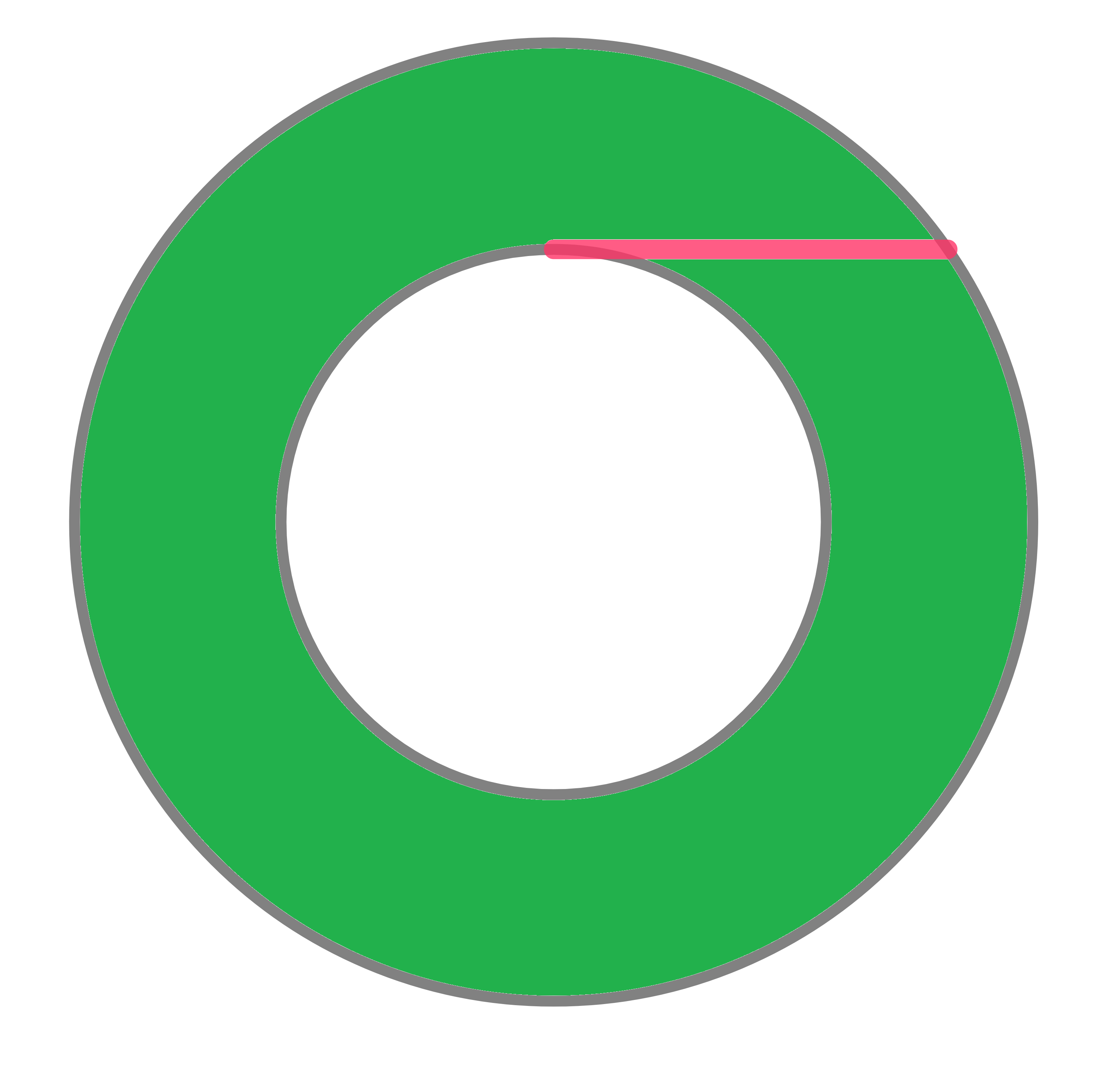
The marked pink segment (tangent to the inner circle) has length $1$. Find the area of the green annulus.

A circle is divided up by the points $A,B,C,D$ so that $⌣AB:⌣BC:⌣CD:⌣DA=2:3:5:6$. The chords $AC$ and $BD$ intersect at point $M$. Find the angle $AMB$.

A circle is divided up by the points $A$, $B$, $C$, $D$ so that $⌣AB:⌣BC:⌣CD:⌣DA=3:2:13:7$. The chords $AD$ and $BC$ are continued until their intersection at point $M$. Find the angle $AMB$.

The bisector of the outer corner at the vertex $C$ of the triangle $ABC$ intersects the circumscribed circle at the point $D$. Prove that $AD=BD$.

The vertex $A$ of the acute-angled triangle $ABC$ is connected by a segment with the center $O$ of the circumscribed circle. The height $AH$ is drawn from the vertex $A$. Prove that $\mathrm{\angle }BAH=\mathrm{\angle }OAC$.

The vertex $A$ of the acute-angled triangle $ABC$ is connected by a segment with the center $O$ of the circumscribed circle. The height $AH$ is drawn from the vertex $A$. Prove that $\mathrm{\angle }BAH=\mathrm{\angle }OAC$.

From an arbitrary point $M$ lying within a given angle with vertex $A$, the perpendiculars $MP$ and $MQ$ are dropped to the sides of the angle. From point $A$, the perpendicular $AK$ is dropped to the segment $PQ$. Prove that $\mathrm{\angle }PAK=\mathrm{\angle }MAQ$.

On a circle, the points $A,B,C,D$ are given in the indicated order. $M$ is the midpoint of the arc $AB$. We denote the intersection points of the chords $MC$ and $MD$ with the chord $AB$ by $E$ and $K$. Prove that $KECD$ is an inscribed quadrilateral.