Problems
There is a triangle with side lengths \(a\), \(b\)
and \(c\). Can you form a triangle with
side lengths \(\frac{a}{b}\), \(\frac{b}{c}\) and \(\frac{c}{a}\)? Does it depend on what \(a\), \(b\)
and \(c\) are? Give a proof if it is
always possible or never possible. Otherwise, construct examples to show
the dependence on \(a\), \(b\) and \(c\).
Recall that a triangle can be drawn with side lengths \(x\), \(y\)
and \(z\) if and only if \(x+y>z\), \(y+z>x\) and \(z+x>y\).
There is a triangle with side lengths \(a\), \(b\)
and \(c\). Does there exist a triangle
with side lengths \(|a-b|\), \(|b-c|\) and \(|c-a|\)? Does it depend on what \(a\), \(b\)
and \(c\) are?
Recall that a triangle can be formed with side lengths \(x\), \(y\)
and \(z\) if and only if all the
inequalities \(x+y>z\), \(y+z>x\) and \(z+x>y\) hold.
There is a triangle with side lenghts \(a\), \(b\) and \(c\). Does there exist a triangle with sides of lengths \(a^2+bc\), \(b^2+ca\) and \(c^2+ab\)? Does it depend on the values of \(a\), \(b\) and \(c\)?
In good conditions, bacteria in a Petri cup spread quite fast, doubling every second. If there was initially one bacterium, then in \(32\) seconds the bacteria will cover the whole surface of the cup.
Now suppose that there are initially \(4\) bacteria. At what time will the bacteria cover the surface of the cup?
A piece containing exactly \(4\) black cells is cut out from a regular \(8\) by \(8\) chessboard. You are only allowed to cut along the edges of the cells and the piece must be connected - namely you cannot have cells attached only with a vertex, they have to share a common edge.
Find the largest possible area of such a piece.
In a distant village, there are \(3\) houses and \(3\) wells. Inhabitants of each house want to have access to all \(3\) wells. Is it possible to build non-intersecting straight paths from each house to each well? All houses and well must be level (that is, none of them are higher up, like on a mountain, nor are any of them on lower ground, like in a valley).
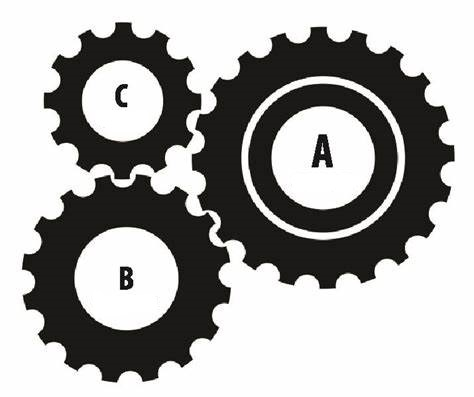
Find all \(n\) such that a closed system of \(n\) gears in a plane can rotate. We call a system closed if the first gear wheel is connected to the second and the \(n\)th, the second is connected to the first and the third, the third is connected to the second and the fourth, the fourth is connected to the third and the fifth, and so on until the \(n\)th is connected to the \(n-1\)th and the first. In the picture, we have a closed system of three gears.
There are various ways to prove mathematical statements. One of the possible methods which might come in handy in certain situations is called proof by contradiction. To prove a statement we first assume that the statement is false and then deduce something that contradicts either the condition, or the assumption itself, or just common sense. Due to the contradiction, we have to conclude that the first assumption must have been wrong, so the statement is actually true.
A closely related method is called contrapositive proof. An example should make the idea quite clear. Consider the statement “if the joke is funny, then I will be laughing". Another completely equivalent way of saying it would be “if I am not laughing, then the joke is not funny". The second statement is known as the contrapositive of the first statement.
We can often prove a statement by proving its contrapositive. Many statements are proven by deriving a contradiction. However, one can often rewrite them as either a direct proof or a contrapositive proof.
Let’s take a look at both of these techniques.
Could you meet a person inhabiting this planet who asks you “Am I a Goop?"
On this planet you meet a couple called Tom and Betty. You hear Tom
ask someone: “Are Betty and I both Goops?"
What kind is Betty?