Problems

The picture below shows the ‘hockey-stick’ identity in Pascal’s triangle.

It says that if you start one of the diagonals formed of \(1\)s at the edge, then follow the numbers diagonally in one direction (e.g. left in the picture) and then change direction on your final turn (right in the picture), then the sum of the all but the last number is equal to the last number.

Prove this is true. Written algebraically, \[\binom{r}{r}+\binom{r+1}{r}+...+\binom{n-1}{r}+\binom{n}{r}=\binom{n+1}{r+1},\] where \(0\le r\le n\).

In the \(n^{\text{th}}\) row of Pascal’s triangle, leave the left \(1\) untouched, multiply the next number along (which is \(\binom{n}{1}=n\)) by \(2\), multiply the next number along (which is \(\binom{n}{2}=\frac{n(n-1}{2}\)) by \(4\), and so on, until you multiply the right-hand \(1\) by \(2^n\). That is, multiply the \(k^{\text{th}}\) number from the left by \(2^k\).

Now what’s the sum of the numbers in the \(n^{\text{th}}\) row?

Let \(s_n\) be the product of the numbers in the \(n^{\text{th}}\) row. (e.g. \(s_3=1\cdot3\cdot3\cdot1=9\)) What’s the limit \[\lim_{n\to\infty}\frac{s_{n-1}s_{n+1}}{s_n^2}?\]

Other than \(1\), does any number appear more than eight times in Pascal’s triangle?

Some randomly chosen people are in a room. A mathematician walks in and says that the probability that there exist at least two people with the same birthday is just over \(50\%\). How many people are in the room?

Today we’ll look at various problems involving probability. [do we need more of an introduction?]

Imagine there’s a disease called ‘mathematitis’ which \(1\%\) of people have. Doctors create a new test to discover whether people have mathematitis. The doctors fine-tune the test until it’s \(99\%\) accurate - that is, if a person \(A\) has it, then \(99\%\) of the time the test will say that \(A\) has it, and \(1\%\) of the time the test will say that \(A\) doesn’t have mathematitis.

Additionally, for person \(B\) who doesn’t have the disease, \(99\%\) of the time the test will correctly identify that \(B\) doesn’t have it - and the other \(1\%\) of the time, the test will say that \(B\) does have mathematitis.

Suppose you don’t know whether you have mathematitis, so you go to the doctors to take this test, and the test says you’ve got it! What’s the probability that you do actually have the disease?

Imagine that people are equally likely to be born in each of the \(12\) months. How many people do you need in a room for the probability that some two are born in the same month to be more than \(50\%\)?

Some doctors make a new test for the disease ‘mathematitis’ which is even better. This new test is \(99.9\%\) accurate - meaning that \(99.9\%\) of the time when someone has the disease, the test will say so. And when someone doesn’t have the disease, \(99.9\%\) of the time the test will say that they don’t have it.

Paul goes to the doctor and test positive for mathematitis. What’s the chance he actually has mathematitis? Recall that \(1\%\) of the population has mathematitis.

Consider the following dice below:

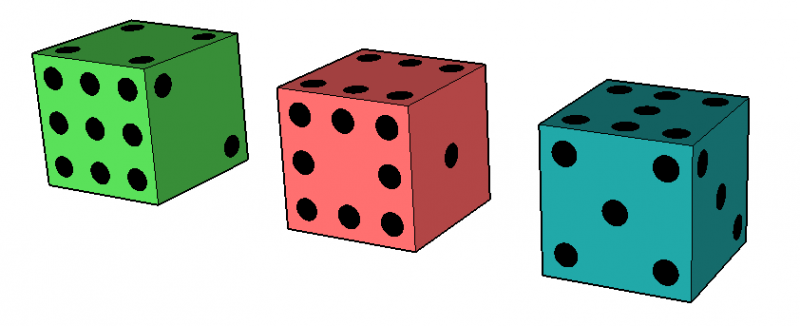
That is, the green die on the left has sides \(2,2,4,4,9,9\), the red die in the middle has sides \(1,1,6,6,8,8\) and the blue die on the right has sides \(3,3,5,5,7,7\). In each of the dice, each side is equally likely to appear.

Imagine we both roll one die, and whoever gets the higher score wins. If I choose the green die, what die should you choose?