Problems

There are \(20\) chairs in the room, which come in two colors: blue and red. Each chair is occupied by either a knight or a liar. Knights always tell the truth, while liars always lie. Initially, each of those seated claimed to be sitting on a blue chair. Then, they switched seats, after which half of the participants asserted that they were now sitting on blue chairs, while the other half claimed to be sitting on red ones. How many knights are currently occupying red chairs?

All the positive fractions smaller than \(1\) with denominators not more than \(100\) are written in a row. Isley and Ella put signs \("+"\) or \("-"\) in front of any fraction, which does not yet have a sign before it. They write signs in turns, but it is known that Isley has to make the last move and calculate the resulting sum. If the total sum turns out to be an integer number, then Ella will give her a chocolate bar. Will Isley be able to get a chocolate bar regardless of Ella’s actions?

Is it possible to cut an equilateral triangle into three equal hexagons?

A labyrinth was drawn on a \(5\times 5\) grid square with an outer wall and an exit one cell wide, as well as with inner walls running along the grid lines. In the picture, we have hidden all the inner walls from you (We give you several copies to facilitate drawing)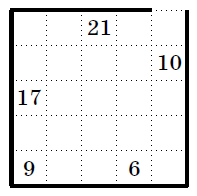 
Please draw how the walls were arranged. Keep in mind that the numbers in the cells represent the smallest number of steps needed to exit the maze, starting from that cell. A step can be taken to any adjacent cell vertically or horizontally, but not diagonally (and only if there is no wall between them, of course).

Is it possible to cut this figure, called "camel"

• a) along the grid lines;

• b) not necessarily along the grid lines;

into \(3\) parts, which you can use to build a square?
(We give you several copies to facilitate drawing)

Michael used different numbers \(\{0,1,2,3,4,5,6,7,8,9\}\) to put in the circles in the picture below, without using any one of them twice. Inside each triangle he wrote down either the sum or the product of the numbers at its vertices. Then he erased the numbers in the circles. Which numbers need to be written in circles so that the condition is satisfied?

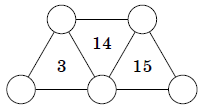
Solve the puzzle: \[\textrm{AC}\times\textrm{CC}\times\textrm{K} = 2002.\] Different letters correspond to different digits, identical letters correspond to identical digits.

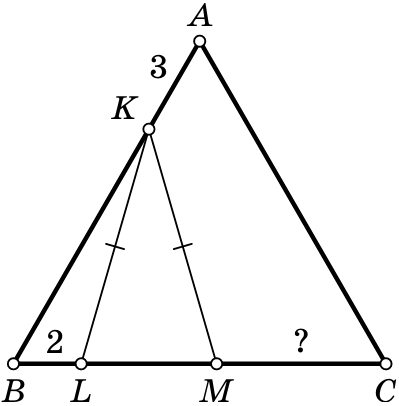
The triangle \(ABC\) is equilateral. The point \(K\) is chosen on the side \(AB\) and points \(L\) and \(M\) are on the side \(BC\) in such a way that \(L\) lies on the segment \(BM\). We have the following properties: \(KL = KM,\) \(BL = 2,\, AK = 3.\) Find the length of \(CM\).

Long ago in a galaxy far away there was a planet of liars and truth tellers, it is known that liars always tell lie, and truth tellers always respond with correct statements. All the inhabitants of the planet look identical to each other, so there is no way to distinguish between liars and truth tellers just by looking at them.
The planet is ruled by the government, where one may encounter honest governors as well as liars. The government is controlled by the High Council, where again one can meet liars and truth tellers.

Last weekend we held the verbal challenge and today we decided to demonstrate solutions of the most juicy problems.