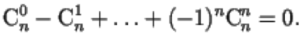Problems
a) Two students need to be chosen to participate in a mathematical Olympiad from a class of 30 students. In how many ways can this be done?
b) In how many ways can a team of three students in the same class be chosen?
How many ways can Susan choose 4 colours from 7 different ones?
On the plane, 10 points are marked so that no three of them lie on the same line. How many triangles are there with vertices at these points?
A person has 10 friends and within a few days invites some of them to visit so that his guests never repeat (on some of the days he may not invite anyone). How many days can he do this for?
Prove that out of \(n\) objects an even number of objects can be chosen in \(2^{n-1}\) ways.
Prove that every number \(a\) in Pascal’s triangle is equal to
a) the sum of the numbers of the previous right diagonal, starting from the leftmost number up until the one to the right above the number \(a\).
b) the sum of the numbers of the previous left diagonal, starting from the leftmost number to the one to left of the number which is above \(a\).
How many ways can you cut a necklace consisting of 30 different beads into 8 pieces (you can cut only between beads)?
How many necklaces can be made from five identical red beads and two identical blue beads?
a) The sports club has 30 members, of which four people are required to participate in the 1,000 metre race. How many ways can this be done?
b) How many ways can I build a team of four people to participate in the relay race 100 m + 200 m + 300 m + 400 m?