Problems

A coin is tossed six times. How many different sequences of heads and tails can you get?

Each cell of a \(3 \times 3\) square can be painted either black, or white, or grey. How many different ways are there to colour in this table?

Consider a set of numbers \(\{1,2,3,4,...n\}\) for natural \(n\). Find the number of permutations of this set, namely the number of possible sequences \((a_1,a_2,...a_n)\) where \(a_i\) are different numbers from \(1\) to \(n\).

A rectangular parallelepiped of the size \(m\times n\times k\) is divided into unit cubes. How many rectangular parallelepipeds are formed in total (including the original one)?

In the Land of Linguists live \(m\) people, who have opportunity to speak \(n\) languages. Each person knows exactly three languages, and the sets of known languages may be different for different people. It is known that \(k\) is the maximum number of people, any two of whom can talk without interpreters. It turned out that \(11n \leq k \leq m/2\). Prove that then there are at least \(mn\) pairs of people in the country who will not be able to talk without interpreters.

Eleven people were waiting in line in the rain, each holding an umbrella. They stood closely together, so that the umbrellas of the neighbouring people were touching (see the figure)

The rain stopped and all people closed their umbrellas. They then shuffled closer, keeping a distance of \(50\) cm between neighbours. By what proportion has the queue length decreased? People can be considered points, and umbrellas are circles with a radius of \(50\) cm.

Cut a square into five triangles in such a way that the area of one of these triangles is equal to the sum of the area of other four triangles.

A circular triangle is a triangle in which the sides are arcs of circles. Below is a circular triangle in which the sides are arcs of circles centered at the vertices opposite to the sides.

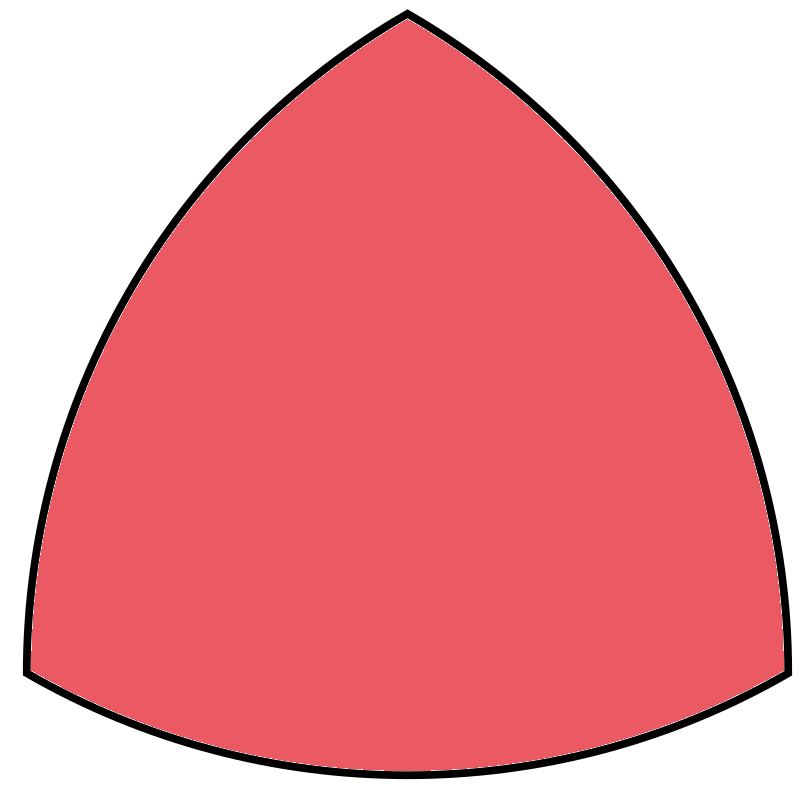
Draw how Robinson Crusoe should put pegs and ropes to tie his goat in order for the goat to graze grass in the shape of the circular triangle.

In a box there are \(20\) cards of different colours: some red, some blue and some yellow. Yellow cards outnumber red ones and there are six times as many yellow cards as blue cards. We draw some cards from the box without looking. What is the minimum number we need to draw to guarantee a red card among them?

There are two piles of rocks, \(10\) rocks in each pile. Fred and George play a game, taking the rocks away. They are allowed to take any number of rocks only from one pile per turn. The one who has nothing to take loses. If Fred starts, who has the winning strategy?