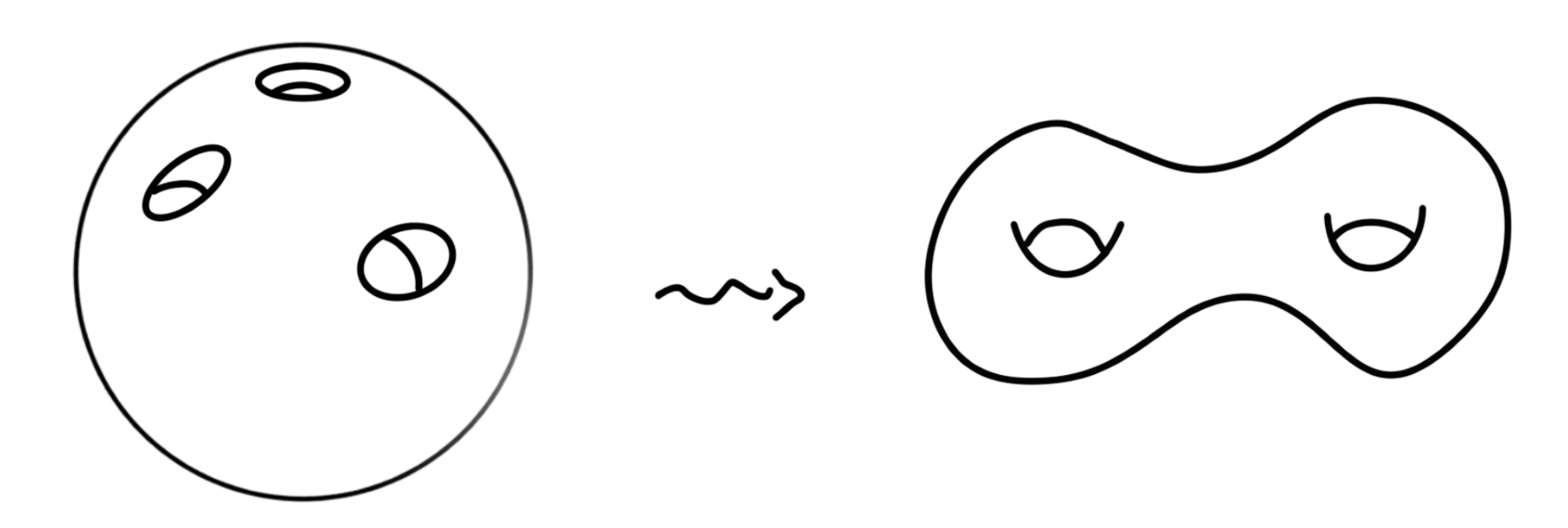Problems
Show that \(x \oplus y = 0\) if and only if \(x = y\). Remember that \(x \oplus y\) denotes the nim-sum of \(x\) and \(y\).
Show that \(\text{Nim}(x,y,z)\) is a losing position if and only if \(x \oplus y \oplus z = 0\). Remember that \(x \oplus y\) denotes the nim-sum of \(x\) and \(y\).
Is \(\text{Nim}(7,11,15)\) a winning position or a losing position? If it is a winning position, what is the optimal move?
Show that \(\text{Nim}(x_1,\dots,x_k)\) is an losing position if and only if \(x_1 \oplus \dots \oplus x_k = 0\). \(x \oplus y\) denotes the nim-sum of \(x\) and \(y\).
Suppose you have a coffee mug made of stretchy and expandable material. How do you mold it into a donut that has a hole inside?
Take a straight string of finite length. Put the right half on top of the left half to form a hoop, and then pass it (the piece now on top) through the hoop from underneath. Glue the two ends together to form the trefoil knot.
Draw a sequence of pictures to illustrate the formation of the trefoil knot.
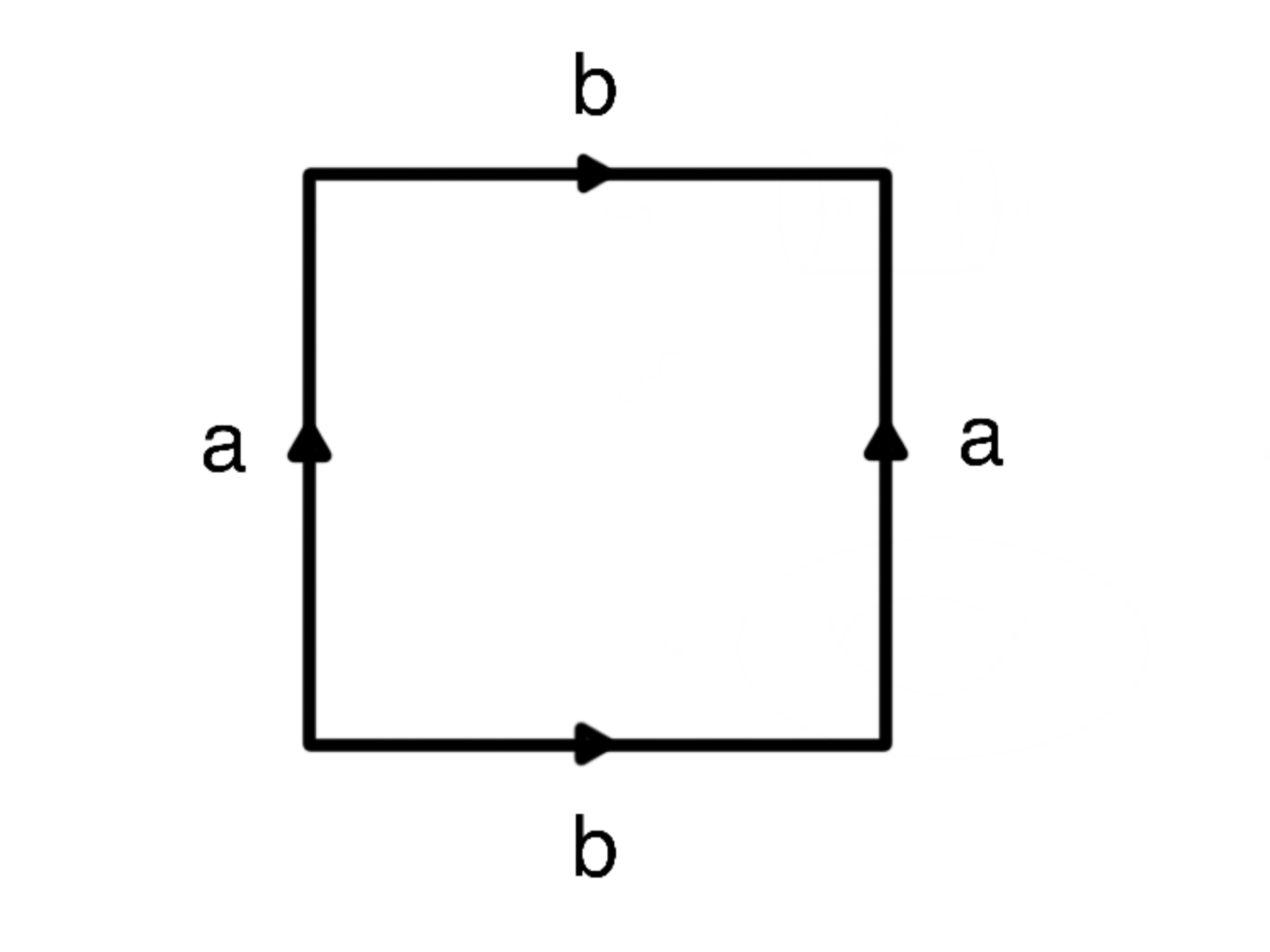
In the picture below, each side is glued in the same direction as its opposite side. Draw a sequence of pictures to show the gluing process and the final shape.
Take a straight string of finite length. Wrap the right half over and then under the left half so that it is pointing to the right again and there is a hoop. Pass the right pointing piece through the hoop from above. Glue the two ends together to form the figure-8 knot.
Draw a sequence of pictures to illustrate the formation of the figure-8 knot, seen below.
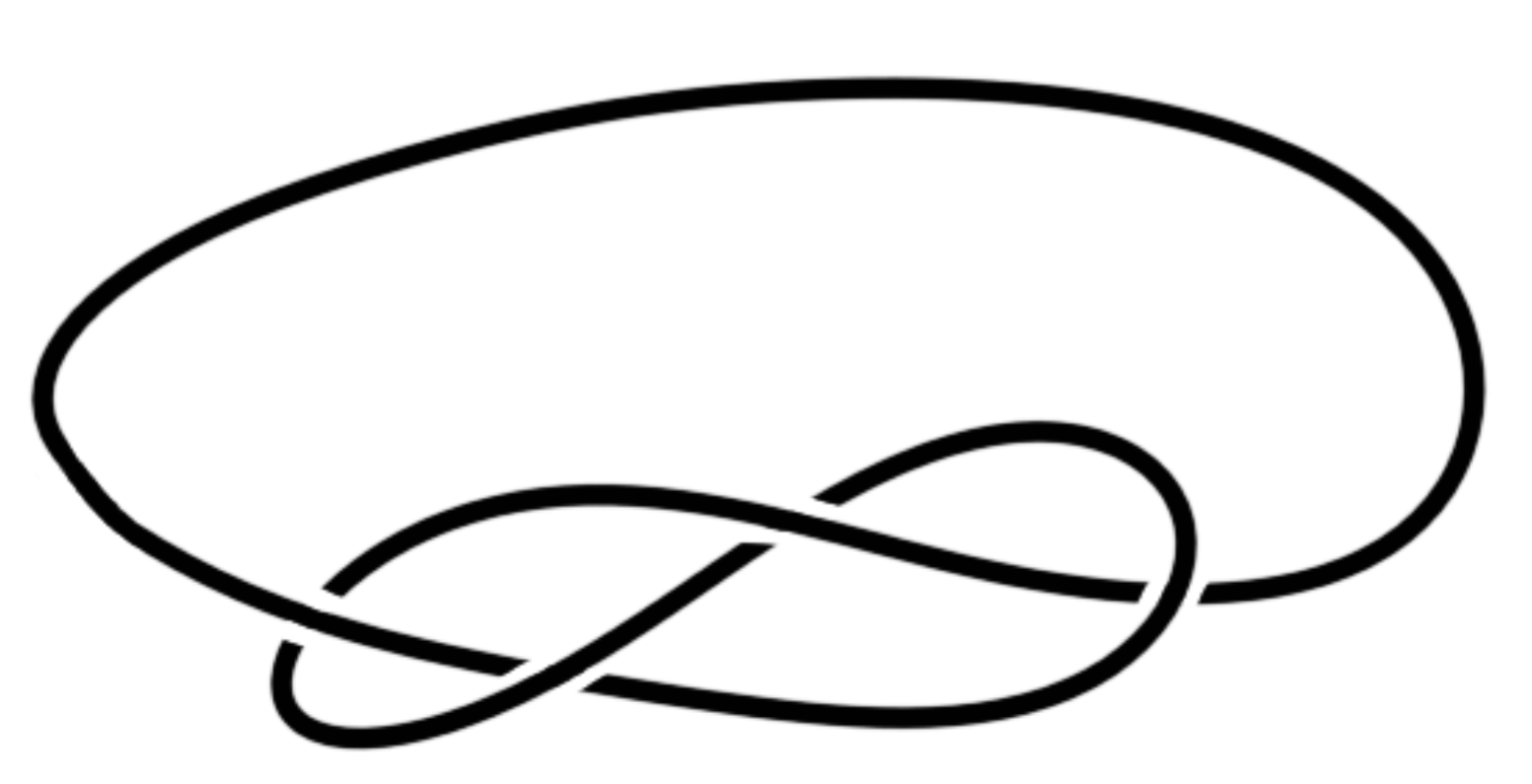
Explain why it is possible to have a picture of a figure-8 knot with 100 crossings.
Imagine the Earth is a perfectly round solid ball. Let us drill from the North Pole, London and Beijing simultaneously and meet at the centre of Earth. A ball with three openings is formed. The surface of this ball is shown on the left of the picture below. Describe how to stretch this surface so that it looks like the surface of a donut with two holes as shown on the right.