Problems
It is easy to construct one equilateral triangle from three identical matches. Can we make four equilateral triangles by adding just three more matches identical to the original ones?
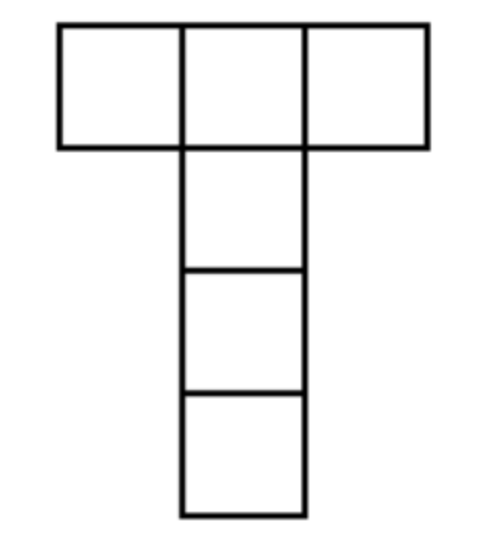
What is the smallest whole number \(n\) such that four identical T-shaped pieces (as shown below) can be placed inside an \(n\times n\) square grid without overlapping?
After having lots of practice with cutting different hexagons with a single cut Jennifer thinks she found a special one. She found a hexagon which cannot be cut into two quadrilaterals. Provide an example of such a hexagon.
My mum once told me the following story: she was walking home late at night after sitting in the pub with her friends. She was then surrounded by a group of unfriendly looking people. They demanded: “money or your life?!” She was forced to give them her purse. She valued her life more, since she was pregnant with me at that time. According to her story she gave them two purses and two coins. Moreover, she claimed that one purse contained twice as many coins as the other purse. Immediately, I thought that the mum must have made a mistake or could not recall the details because of the shock and the amount of time that passed after that moment. But then I figured out how this could be possible. Can you?
A new airline "Capitals Direct" has direct flights operating on the following routes (in both directions): Paris - London, Paris - Lisbon, Rome - London, Rome - Madrid, Berlin - Helsinki, Berlin - Amsterdam, Amsterdam - Prague. These are the only flights that the company offers. I would like to travel from Paris to Amsterdam. I cannot buy a direct ticket for sure, but can "Capitals Direct" offer me a connecting flight?
Can one arrange numbers from \(1\) to \(9\) in a row so that each pair of consecutive numbers forms a two-digit multiple of \(7\) or a multiple of \(13\)?
In the Royal Grammar School all Year \(9\) students were gathered in the Queen’s Hall for an important announcement. They have been waiting for it for a while and everyone had enough time to greet every other student with a handshake. Assuming there are \(100\) Year \(9\) students at the school, how many handshakes were made before the announcement?
In \(2149\) a regular transport connection between nine planets of the Solar System was introduced. Space capsules are flying between the following pairs of planets: Earth – Mercury, Pluto – Venus, Earth – Pluto, Pluto – Mercury, Mercury – Venus, Uranus – Neptune, Neptune – Saturn, Saturn – Jupiter, Jupiter – Mars, and Mars – Uranus. Is it possible to travel from Earth to Mars by using this type of transport with possible changes at other planets?
Is it possible to find a way of arranging numbers from \(0\) to \(9\) in a row so that each pair of consecutive numbers adds up to a multiple of \(5\), \(7\), or \(13\)?
Each pair of cities in Wonderland is connected by a flight operated by "Wonderland Airlines". How many cities are there in the country if there are \(105\) different flights? We count a flight from city \(A\) to city \(B\) as the same as city \(B\) to city \(A\) - i.e. the pair \(A\) to \(B\) and \(B\) to \(A\) counts as one flight.