We solve problems

Problems Algebra and arithmetic Number systems Binary number system Set theory and logic Theory of algotithms

Michael thinks of a number no less than \(1\) and no greater than \(1000\). Victoria is only allowed to ask questions to which Michael can answer “yes” or “no” (Michael always tells the truth). Can Victoria figure out which number Michael thought of by asking \(10\) questions?

Problem #PRU-102998

Problems Algebra and arithmetic Number theory. Divisibility Divisibility of a number. General properties Set theory and logic Joke problem

10–12 2.0

Can the equality \(K \times O \times T = U \times W \times E \times N \times H \times Y\) be true if the numbers from 1 to 9 are substituted for the letters? Different letters correspond to different numbers.

Problem #PRU-102999

Problems Algebra and arithmetic Arithmetics. Mental maths Combinatorics

10–12 2.0

Deep in a forest there is a small town of talking animals. Elephant, Crocodile, Rabbit, Monkey, Bear, Heron and Fox are friends. They each have a landline telephone and each two telephones are connected by a wire. How many wires were required?

Problem #PRU-103009

Problems Set theory and logic Algorithm Theory Algorithm Theory

10–12 2.0

There are 6 locked suitcases and 6 keys to them. At the same time, it is not known to which suitcase each key fits. What is the smallest number of attempts you need to make in order to open all the suitcases for sure? And how many attempts will it take there are not 6 but 10 keys and suitcases?

Problem #PRU-103027

Problems Set theory and logic Algorithm Theory Algorithm Theory

10–12 2.0

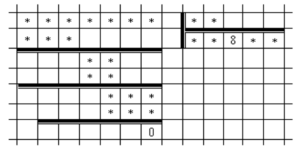
Decipher the following rebus (see the figure). Despite the fact that only two figures are known here, and all others are replaced by asterisks, the example can be restored.

Problem #PRU-103729

Problems Algebra and arithmetic Number theory. Divisibility Odd and even numbers

10–12 2.0

The parliament of a certain country has two houses with an equal number of members. In order to make a decision on an important issue all the members voted and there were no abstentions. When the chairman announced that the decision had been taken with a 23-vote advantage, the opposition leader declared that the results had been rigged. How did he know it?

Problem #PRU-103738

Problems Set theory and logic Set theory Union, intersection, and set difference

11–12 2.0

Among some number of mathematicians, every seventh is a philosopher, and among some number of philosophers every ninth is a mathematician. Who are there more of: philosophers or mathematicians?

Problem #PRU-103749

Problems Set theory and logic Order relations Methods Pigeonhole principle Pigeonhole principle (other)

10–12 2.0

Know-it-all came to visit the twin brothers Screw and Nut, knowing that one of them never speaks the truth, and asked one of them: “Are you Screw?”. “Yes,” he replied. When Know-it-all asked the second brother the same question, he received an equally clear answer and immediately determined who was who.

Who was called Screw?

Problem #PRU-103757

Problems Algebra and arithmetic Algebraic equations and systems of equations Higher order equations. Palindromic polynomial equations Equations of higher order (other) Calculus Real numbers Integer and fractional parts. Archimedean property

12–14 2.0

A resident of one foreign intelligence agency informed the centre about the forthcoming signing of a number of bilateral agreements between the fifteen former republics of the USSR. According to his report, each of them will conclude an agreement exactly with three others. Should this resident be trusted?

Problem #PRU-103780

Problems Methods Algebraic methods Partitions into pairs and groups; bijections Pigeonhole principle Pigeonhole principle (other) Proof by exhaustion

11–13 3.0

In any group of 10 children, out of a total of 60 pupils, there will be three who are in the same class. Will it always be the case that amongst the 60 pupils there will be: 1) 15 classmates? 2) 16 classmates?