Problems

Is it possible to cut this figure, called "camel"

• a) along the grid lines;

• b) not necessarily along the grid lines;

into $3$ parts, which you can use to build a square?
(We give you several copies to facilitate drawing)

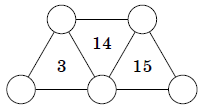
Michael used different numbers $\{0,1,2,3,4,5,6,7,8,9\}$ to put in the circles in the picture below, without using any one of them twice. Inside each triangle he wrote down either the sum or the product of the numbers at its vertices. Then he erased the numbers in the circles. Which numbers need to be written in circles so that the condition is satisfied?

Solve the puzzle: $$\text{AC}\times \text{CC}\times \text{K}=2002.$$ Different letters correspond to different digits, identical letters correspond to identical digits.

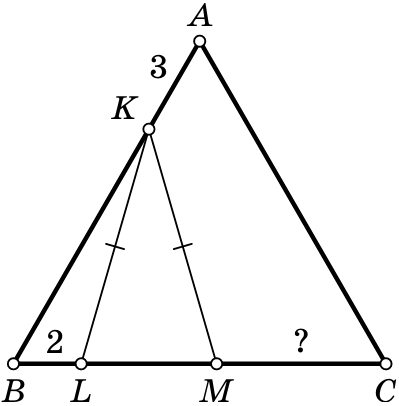
The triangle $ABC$ is equilateral. The point $K$ is chosen on the side $AB$ and points $L$ and $M$ are on the side $BC$ in such a way that $L$ lies on the segment $BM$. We have the following properties: $KL=KM,$ $BL=2,AK=3.$ Find the length of $CM$.

Long ago in a galaxy far away there was a planet of liars and truth tellers, it is known that liars always tell lie, and truth tellers always respond with correct statements. All the inhabitants of the planet look identical to each other, so there is no way to distinguish between liars and truth tellers just by looking at them.
The planet is ruled by the government, where one may encounter honest governors as well as liars. The government is controlled by the High Council, where again one can meet liars and truth tellers.

Last weekend we held the verbal challenge and today we decided to demonstrate solutions of the most juicy problems.

Today we will focus on the study of Euclidean geometry of plane figures. Around 300 BCE a Greek mathematician Euclid developed a rigorous way to study plane geometry in his work Elements based on axioms (statement assumed to be correct) and theorems (statements deduced from axioms). The axioms of Euclidean Elements are the following:

• For any two different points, there exists a line containing these two points, and this line is unique.

• A straight line segment can be prolonged indefinitely.

• A circle is defined by a point for its centre and a distance for its radius.

• All right angles are equal.

• For any line $L$ and point $P$ not on $L$, there exists a line through $P$ not meeting $L$, and this line is unique.

In examples we deduce from the axioms above the following basic principles:
1. The supplementary angles (angles "hugging" a straight line) add up to ${180}^{\circ }$.
2. The sum of all internal angles of a triangle is also ${180}^{\circ }$.
3. A line cutting two parallel lines cuts them at the same angles (these are called corresponding angles).
4. In an isosceles triangle (which has two sides of equal lengths), the two angles touching the third side are equal.

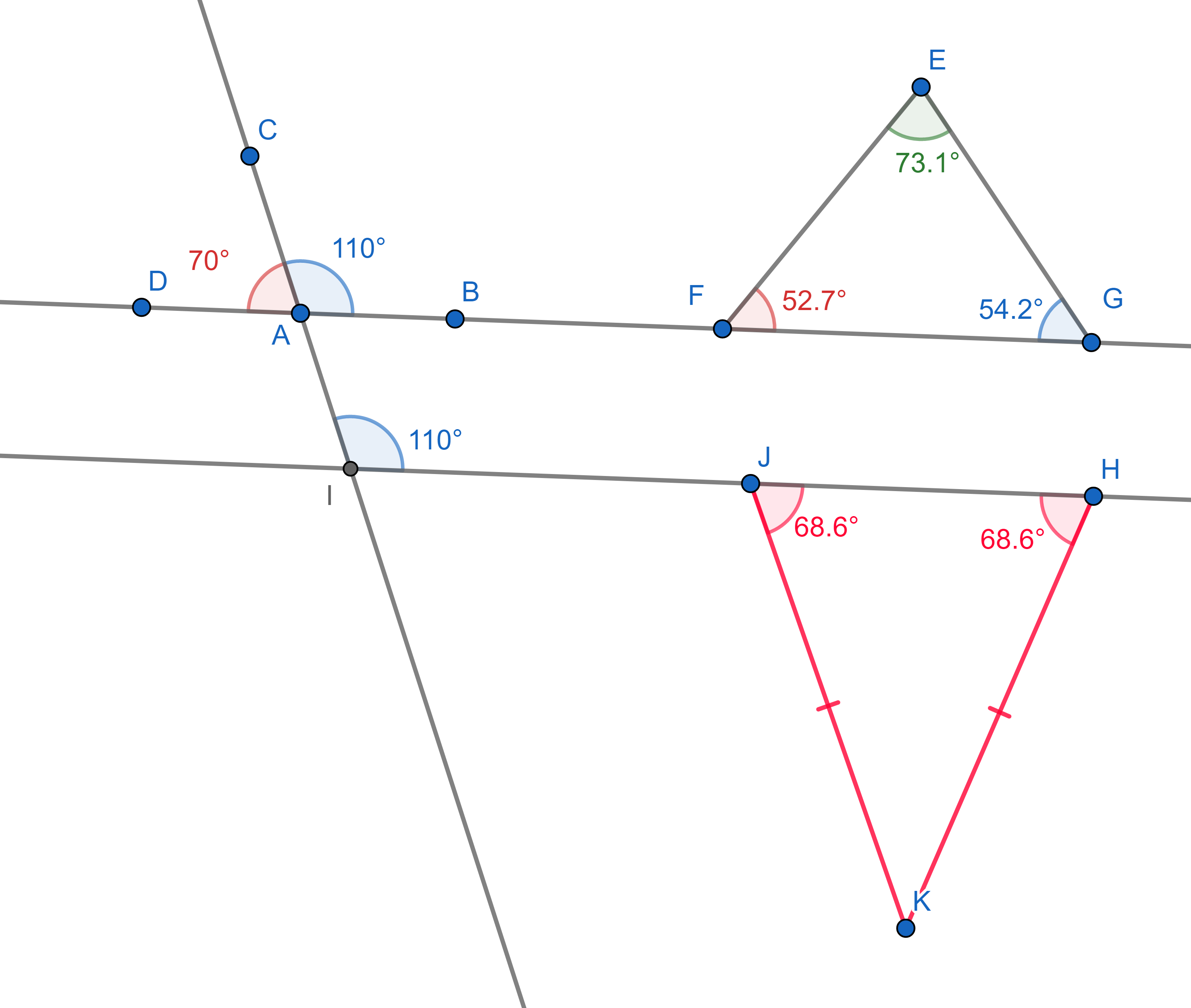
Let’s have a look at some examples of how to apply these axioms to prove geometric statements.

• We call two figures congruent if their corresponding sides and angles are equal. Let $ABD$ an $A^{\prime }{B}^{\prime }{D}^{\prime }$ be two right-angled triangles with right angle $D$. Then if $AD=A^{\prime }{D}^{\prime }$ and $AB=A^{\prime }{B}^{\prime }$ then the triangles $ABD$ and $A^{\prime }{B}^{\prime }{D}^{\prime }$ are congruent.

• It follows from the previous statement that if two lines $AB$ and $CD$ are parallel than angles $BCD$ and $CBA$ are equal.

We prove the other two assertions from the description:

• The sum of all internal angles of a triangle is also ${180}^{\circ }$.

• In an isosceles triangle (which has two sides of equal lengths), two angles touching the third side are equal.

In the triangle $ABC$ the sides are compared as following: $AC>BC>AB$. Prove that the angles are compared as follows: $\mathrm{\angle }B>\mathrm{\angle }A>\mathrm{\angle }C$.