Problems

There exist various ways to prove mathematical statements, one of the possible methods, which might come handy in certain situations is called Proof by contradiction. To prove a statement we first assume that the statement is false and then deduce something that contradicts either the condition, or the assumption itself, or just common sense. Thereby concluding that the first assumption must have been wrong, so the statement is actually true.

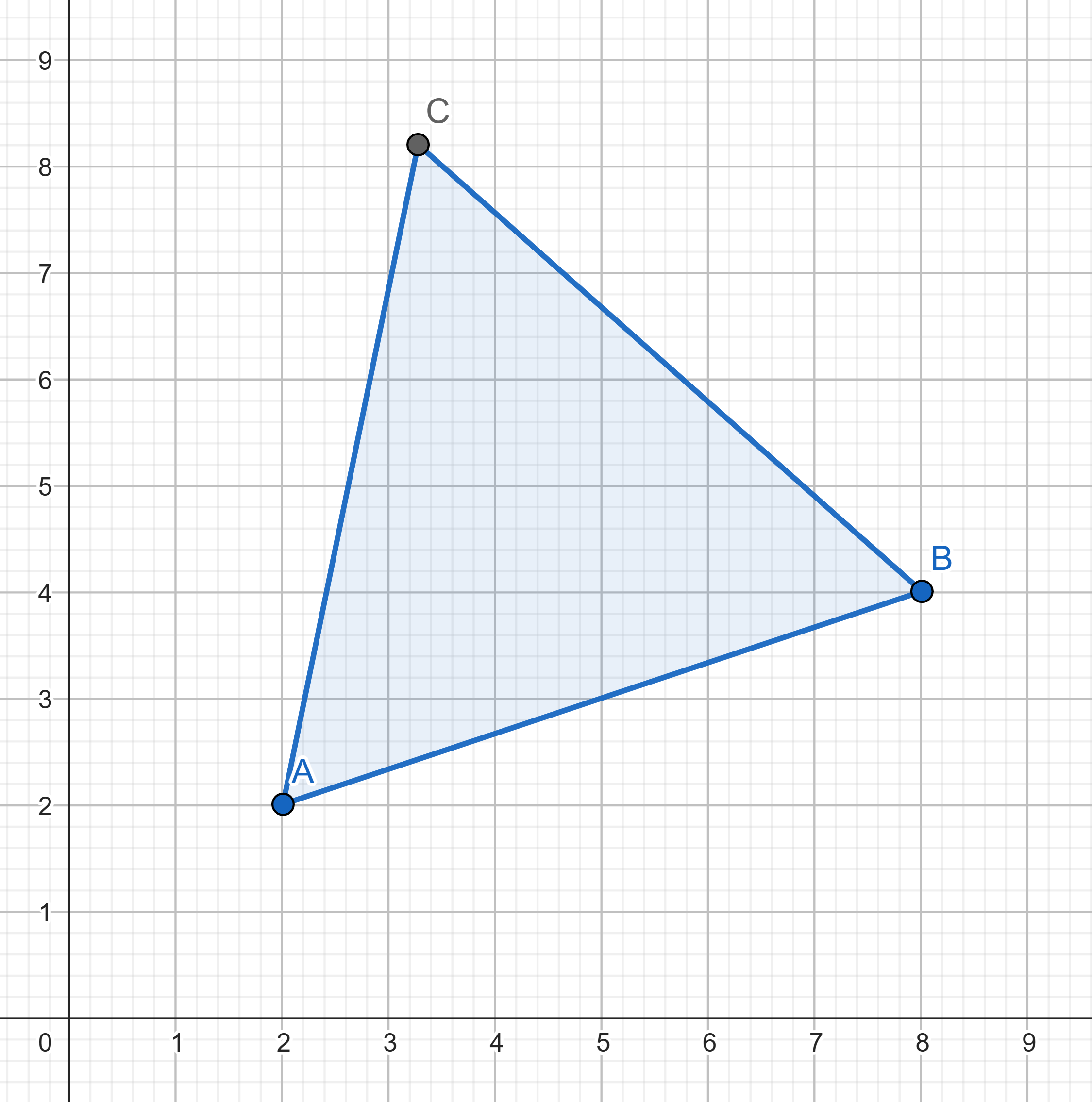
Can three points with integer coordinates be the vertices of an equilateral triangle?

Prove that there are infinitely many natural numbers \(\{1,2,3,4,...\}\).

Prove that there are infinitely many prime numbers \(\{2,3,5,7,11,13...\}\).

Is it possible to colour the cells of a \(3\times 3\) board red and yellow such that there are the same number of red cells and yellow cells?

Prove the divisibility rule for \(25\): a number is divisible by \(25\) if and only if the number made by the last two digits of the original number is divisible by \(25\);
Can you come up with a divisibility rule for \(125\)?

Which of the following numbers are divisible by \(11\) and which are not? \[121,\, 143,\, 286, 235, \, 473,\, 798, \, 693,\, 576, \,748\] Can you write down and prove a divisibility rule which helps to determine if a three digit number is divisible by \(11\)?

Sometimes one can guess certain multiples of a number just by looking at it, the idea of this sheet is to learn to recognise quickly using tricks when a natural number is divisible by another number.

Today we will study the method of finding the amount of combinations, or consecutive actions, or ways to select items from a bag which is called the Product rule. The main idea of this combinatorial is the following: if you are asked to perform an action that can be done in, say \(5\) ways and another action afterwards that can be done in \(4\) ways, then the total number of possibilities to perform two consecutive actions would be equal to \(5\times 4\). The reason for this is the opportunity to choose \(4\) possible second actions for each of the \(5\) choices of the first action already made before.

In how many ways can eight rooks be arranged on the chessboard in such a way that none of them can take any other. The color of the rooks does not matter, it’s everyone against everyone.