Problems
The numbers \(a,b,c\) are positive. Show that: \[\frac{ab}{c} + \frac{bc}{a} + \frac{ac}{b} \ge a +b+c.\]
The number \(n\) is natural. Show that: \[\frac1{\sqrt{1}} +\frac1{\sqrt{2}}+ \frac1{\sqrt{3}} + \dots +\frac1{\sqrt{n}} < 3 \sqrt{n+1} -3.\]
If \(n\) is a positive integer, we denote by \(s(n)\) the sum of the divisors of \(n\). For example, the divisors of \(n=6\) are \(1,2,3,6\), so \(s(6)=1+2+3+6=12\). Prove that, for all \(n\geq1\), \[s(1)+s(2)+\cdots+s(n)\leq n^2.\] Denote by \(t(n)\) is instead the sum of the squares of the divisors of \(n\) (e.g., \(t(6)=1^2+2^2+3^2+6^2=50\)), can you find a similar inequality for \(t(n)\)?
Consider the following sum: \[\frac1{1 \times 2} + \frac1{2 \times 3} + \frac1{3 \times 4} + \dots\] Show that no matter how many terms it has, the sum will never be larger than \(1\).
Cut a \(7\times 7\) square into \(9\) rectangles, out of which you can construct any rectangle whose sidelengths are less than \(7\). Show how to construct the rectangles.
There are \(16\) cities in the kingdom. We would like to build roads between these cities so that one can get from any city to any other without passing through more than one city on the way. To save cost, we would like to have no more than four roads coming out of each city. Prove that such a system of roads is unfortunately impossible to build.
There are \(16\) cities in the kingdom. Show that it is possible to build a system of roads in such a way that one can get from any city to any other without passing through more than one city on the way, and with at most five roads coming out of each city.
Today’s topic is inequalities, expressions like \(a\geq b\), or \(a>b\). There are certain rules for operating inequalities: one can subtract the same number from both sides of the inequality, namely if \(a\geq b\), then \(a-b \geq 0\). If \(a \geq b\) and \(b\geq c\), then \(a\geq c\). If a number \(c\geq 0\), then from \(a\geq b\) it follows that \(ac \geq bc\). However, in case of multiplication by a negative number \(c\leq 0\), the inequality sign reverses: from \(a\geq b\) it follows that \(ac \leq bc\). One should also remember that the square of any real number is non-negative.
Recall that a line is tangent to a circle if they have only one point
of intersection, a circle is called inscribed in a polygon if it is
tangent to every side as a segment of that polygon.
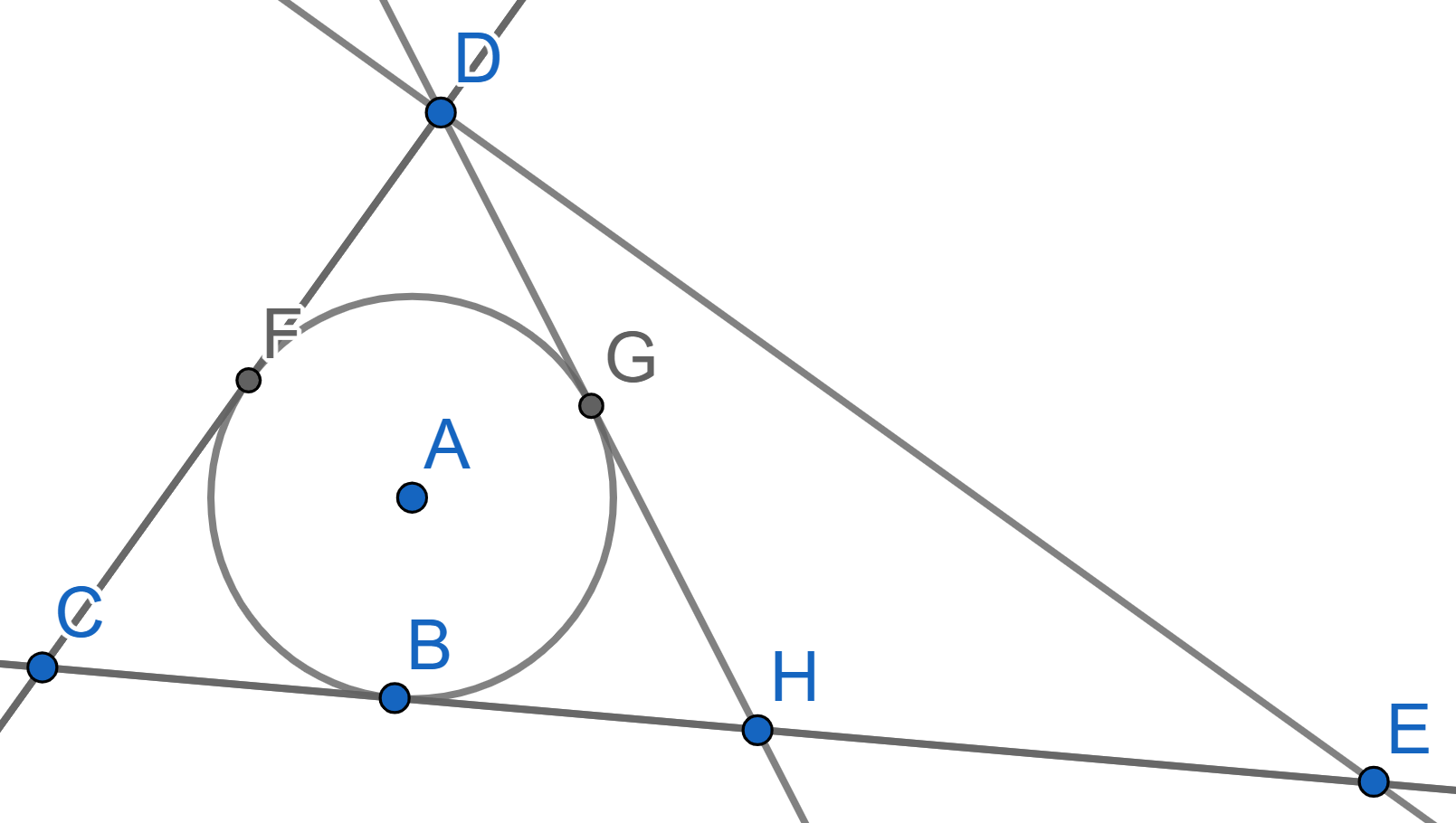
In the triangle \(CDE\) the angle \(\angle CDE = 90^{\circ}\) and the line
\(DH\) is the median. A circle with
center \(A\) is inscribed in the
triangle \(CDH\) and is tangent to the
segment \(DH\) in its middle, let’s
denote it as \(G\), so \(GH=DG\). Find the angles of the triangle
\(CDE\).
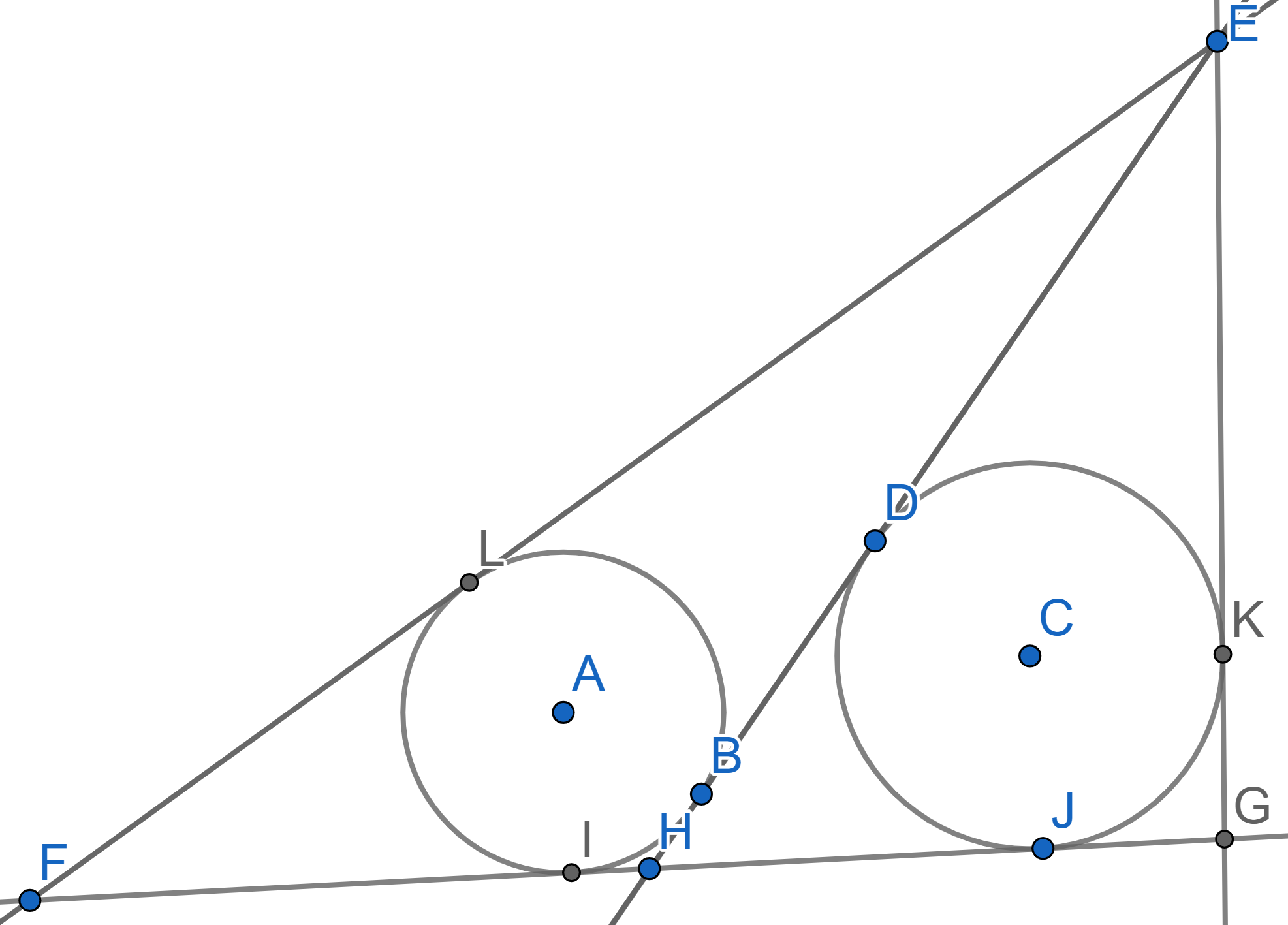
Recall that a line is tangent to a circle if they have only one point
of intersection, a circle is called inscribed in a polygon if it is
tangent to every side as a segment of that polygon.
In the triangle \(EFG\) the line \(EH\) is the median. Two circles with
centres \(A\) and \(C\) are inscribed into triangles \(EFH\) and \(EGH\) respectively, they are tangent to the
median \(EH\) at the points \(B\) and \(D\). Find the length of \(BD\) if \(EF-EG=2\).