Problems
A wide variety of questions in mathematics starts with the question ’Is it possible...?’. In such problems you would either present an example, in case the described situation is possible, or rigorously prove that the situation is impossible, with the help of counterexample or by any other means. Sometimes the border between what seems should be possible and impossible is not immediately obvious, therefore you have to be cautious and verify that your example (or counterexample) satisfies the conditions stated in the problem. When you are asked the question whether something is possible or not and you suspect it is actually possible, it is always useful to ask more questions to gather additional information to narrow the possible answers. You can ask for example "How is it possible"? Or "\(\bf Which\) properties should the correct construction satisfy"?
Jane wrote another number on the board. This time it was a two-digit number and again it did not include digit 5. Jane then decided to include it, but the number was written too close to the edge, so she decided to t the 5 in between the two digits. She noticed that the resulting number is 11 times larger than the original. What is the sum of digits of the new number?
a) Find the biggest 6-digit integer number such that each digit, except for the two on the left, is equal to the sum of its two left neighbours.
b) Find the biggest integer number such that each digit, except for the rst two, is equal to the sum of its two left neighbours. (Compared to part (a), we removed the 6-digit number restriction.)
Matt built a simple wooden hut to protect himself from the rain. From the side the hut looks like a right triangle with the right angle at the top. The longer part of the roof has 20 ft and the shorter one has 15 ft. What is the height of the hut in feet?
Tile a \(5\times6\) rectangle in an irreducible way by laying \(1\times2\) rectangles.
Does there exist an irreducible tiling with \(1\times2\) rectangles of a \(4\times 6\) rectangle?
Irreducibly tile a floor with \(1\times2\) tiles in a room that is a \(5\times8\) rectangle.
Tile the whole plane with the following shapes:
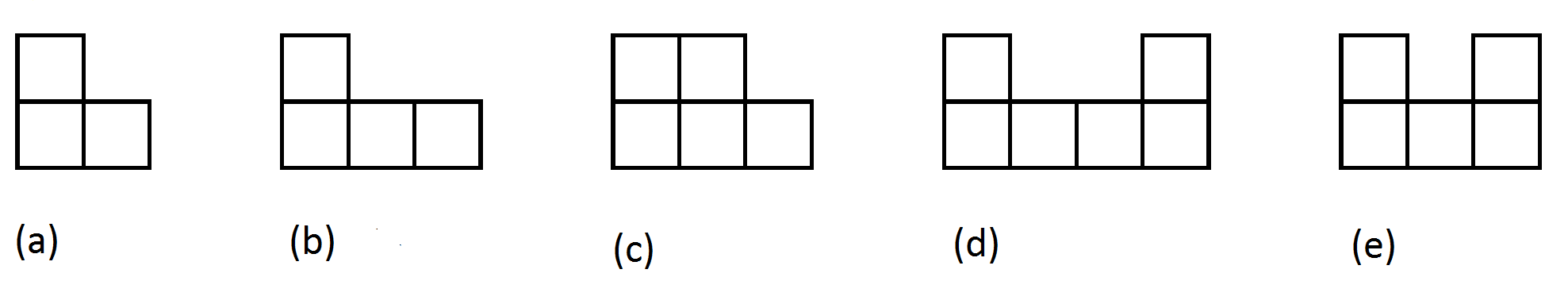
David Smith cut out 12 nets. He claimed that it was possible to make a cube out of each net. Roger Penrosae looked at the patterns, and after some considerable thought decided that he was able to make cubes from all the nets except one. Can you figure out which net cannot make a cube?
It is known that it is possible to cover the plane with any cube’s net. Show how you can cover the plane with nets below:
