Problems
We will assume that the birth of a girl and a boy is equally probable. It is known that in some family there are two children.
a) What is the probability that one of them is a boy and one a girl?
b) Additionally, it is known that one of the children is a boy. What is the probability that there is one boy and one girl in the family now?
c) Additionally, it is known that the boy was born on a Monday. What is the probability that there is one boy and one girl in the family now?
The figure shows a payment order to pay an electricity bill to some power supply company for March 2013.
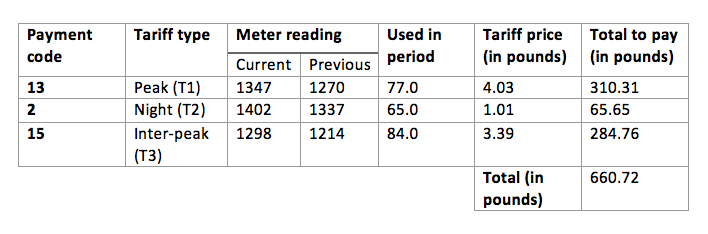
Every month, the client sends the company the testimony of a three-tariff meter installed her the apartment. From the indications for the current month, the corresponding indications for the previous month are subtracted, and the actual monthly expenditure is obtained for each of the three tariff zones (peak, night, inter-peak). Then the expense for each zone is multiplied by the price of one kilowatt-hour in this zone. Adding the received amounts, the client receives the total amount of payment for a month. In this example, the customer will pay £660.72.
The company maintains a record of electricity consumption and payment, using the data received from the customer. The problem is that the company sometimes confuses the six numbers obtained, rearranging them in an arbitrary order, however, it ensures that the current reading remains greater than the previous one. As a result, the calculation of the company may be flawed. If the company believes that the client must pay more than she has paid, the company requires additional payment.
Using the data from the receipt shown, find:
a) the maximum possible amount of surcharge for March 2013, which the company will require from the client;
b) the mathematical expectation of the difference between the amount that the company calculates and the amount paid by the client.
Every day, Patrick the dog chews one slipper from the available stock in the house. Strictly with a probability of 0.5 Patrick wants to chew the left slipper, and with a probability of 0.5 – the right one. If the desired slippers are not present, Patrick becomes upset. How many pairs of the same slippers need to be bought, so that with a probability of not less than 0.8 Patrick does not get upset for an entire week (7 days)?
Find the probability that heads will fall an even number of times, in an experiment in which:
a) a symmetrical coin is thrown \(n\) times;
b) a coin is thrown \(n\) times, for which the probability of getting heads in one throw is \(p(0 < p < 1)\).
In Anchuria, presidential elections are being prepared, in which President Miraflores wants to win. Exactly half of the voters support Miraflores, and the other half support Dick Maloney. Miraflores is also a voter. According to the law, he has the right to divide all of the voters into two constituencies at his own discretion. In each of the districts, the voting is conducted as follows: each voter marks the name of their candidate on the ballot; all ballots are placed in the ballot box. Then one random ballot is chosen from the ballot box, and the one whose name is marked on it will win in this district. The candidate wins the election only if he wins in both districts. If the winner does not appear, the next round of voting is appointed according to the same rules. How should Miraflores divide the electorate in order to maximize the probability of his victory on the first round?
In a terrible thunderstorm, along a rope ladder, \(n\) dwarfs ascend in a chain. If suddenly there is a thunderbolt, then from fear, every gnome, regardless of others, can fall with probability \(p\) (\(0 < p < 1\)). If the dwarf falls, then he also hits all of the dwarfs that are below him. Find:
a) The probability that exactly \(k\) dwarfs will fall.
b) The mathematical expectation of the number of fallen dwarfs.
We throw a symmetrical coin \(n\) times. Suppose that heads came up \(m\) times. The number \(m/n\) is called the frequency of the fall of heads. The number \(m/n - 0.5\) is called the frequency deviation from the probability, and the number \(|m/n - 0.5|\) is called the absolute deviation. Note that the deviation and the absolute deviation are random variables. For example, if a coin was thrown 5 times and heads came up two times, the deviation is equal to \(2/5 - 0.5 = -0.1\), and the absolute deviation is 0.1.
The experiment consists of two parts: first the coin is thrown 10 times, and then – 100 times. In which of these cases is the mathematical expectation of the absolute deviation of the frequency of getting heads is greater than the probability?
In the magical land of Anchuria there are only \(K\) laws and \(N\) ministers. The probability that a randomly chosen minister knows a randomly chosen law is \(p\). One day, the ministers gathered for a meeting, to write the Constitution. If at least one minister knows the law, then this law will be taken into account in the Constitution, otherwise this law will not be taken into account in the Constitution. Find:
a) The probability that exactly \(M\) laws will be taken into account into the Constitution.
b) The mathematical expectation of the number of registered laws.
The probability of the birth of twins in Cambria is \(p\), and no triplets are born in Cambria.
a) Evaluate the probability that a random Cambrian that one meets on the street is one of a pair of twins?
b) There are three children in a random Cambrian family. What is the probability that among them there is a pair of twins?
c) In Cambrian schools, twins must be enrolled in the same class. In total, there are \(N\) first-graders in Cambria.
What is the expectation of the number of pairs of twins among them?
There is a deck of playing cards on the table (for example, in a row). On top of each card we put a card from another deck. Some cards may have coincided. Find:
a) the mathematical expectation of the number of cards that coincide;
b) the variance of the number of cards that coincide.