Problems
In a certain country, there are \(n\) cities. Some of them are connected by
roads, all of which go in both directions. It is possible to get from
any city to any other city using only roads. However, for any pair of
cities, there is always only one way to get from one of them to the
other and there are no alternative routes.
Show that there are exactly \(n-1\)
roads in this country.
If \(x\) is any positive real number and \(n \ge 2\) is a natural number, show that \[(1+x)^n > 1+nx\]
Anna and Bob play a game with the following rules: they both receive
a positive integer number. They do not know each other’s numbers, but
they do know that their numbers come one after another – they do not
know which one is larger. (If Anna gets \(n\), Bob gets either \(n-1\) or \(n+1\)). Anna then asks Bob – “do you know
what number I have?” If Bob does know, he has to say Anna’s number and
he wins the game. If he does not, he has to say that he does not. Then,
he asks Anna if she knows his number. If Anna does not know, she asks
Bob. This continues until one of them finds out what is the other’s
number. Assuming that both Anna and Bob know mathematics sufficiently
well to be able to solve this problem, find out who wins the game and
how.
For simplicity let’s assume Bob always gets the odd number and Anna
always gets the even number - two consecutive numbers have opposite
parity!
A real number \(y\) is such that \(y+\frac1{y}\) happens to be an integer. Show that for any natural \(n\), it is also true that \(y^n + \frac1{y^n}\) is an integer.
DRAFT
We need to ensure that there isn’t overlap with the first areas problem
sheet.
We can introduce the areas of a new shape, e.g. a trapezium more
formally. Maybe an ellipse?
We’ll explore how to tile the plane using rectangles, more general quadrilaterals, pentagons and more unconventional shapes. In this exercise sheet, we define a plane tiling as a covering of the entire plane, without any gaps or overlaps, using identical geometric shapes that can be rotated or reflected to one another. Usually, it is sufficient to cover a small portion of the plane with a particular pattern that we see can be extended to cover the entire plane.
In the majority of today’s problems, one only needs to create a pattern for a small section of the plane, which can then be extended to cover the entire plane through repetition. Such tilings are referred to as periodic. Finding a tiling of the plane using identical shapes that is not periodic, meaning the pattern never repeats itself, has been a long-standing open problem. However, this problem was solved in an article published in March 2023 by David Smith, Joseph Samuel Myers, Craig S. Kaplan, and Chaim Goodman-Strauss. The idea of their solution is the following diagram from the article:
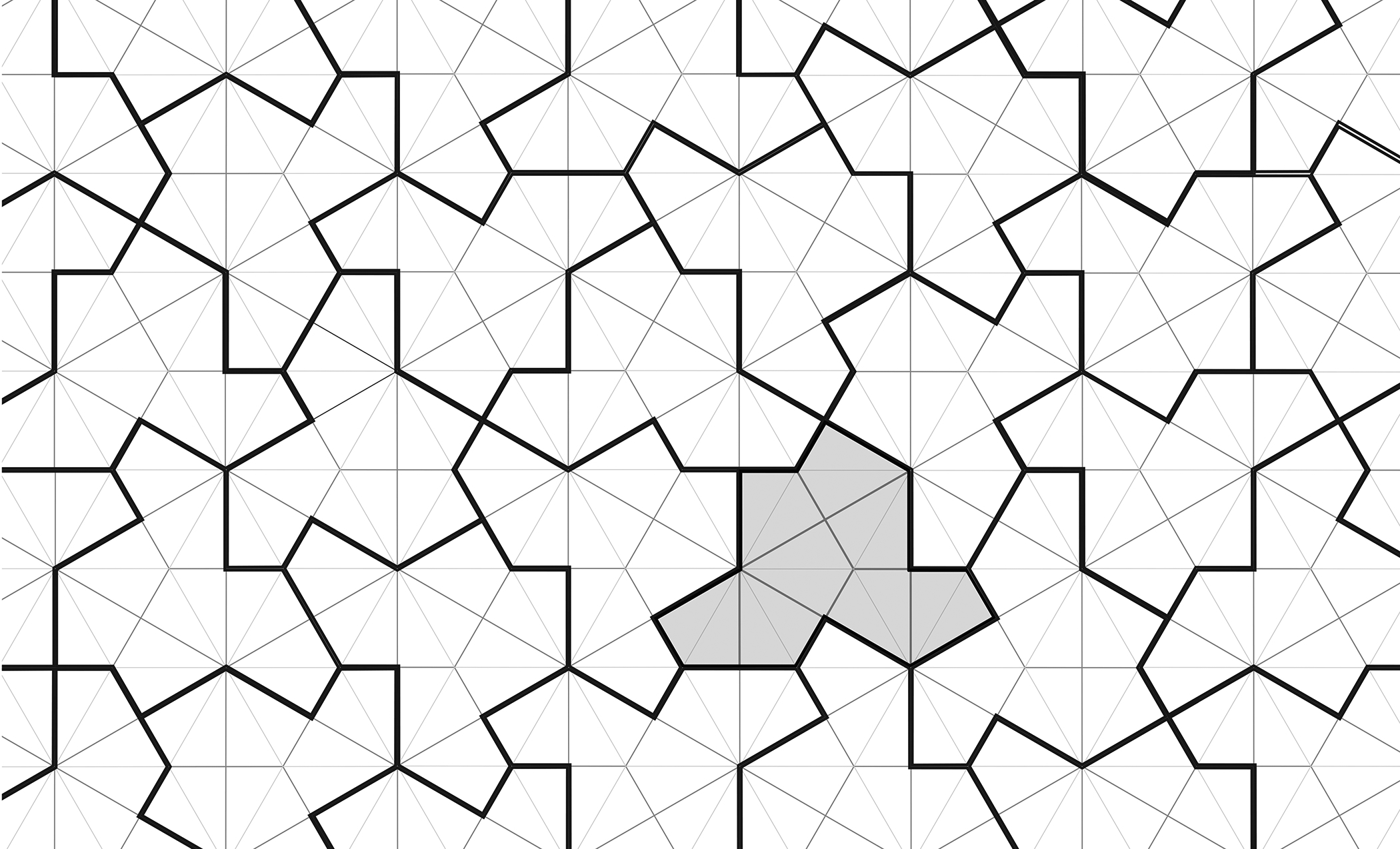
The hard part is to prove that this tiling is aperiodic.
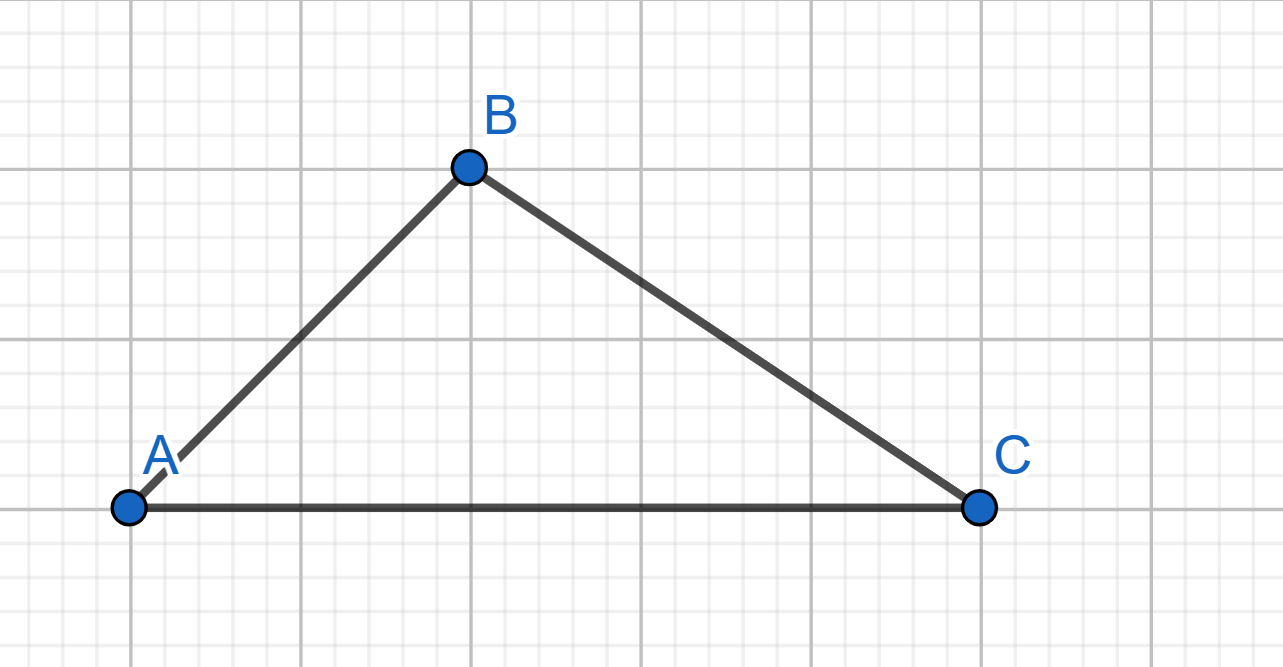
Show how to cover the plane with triangles of the following shape.
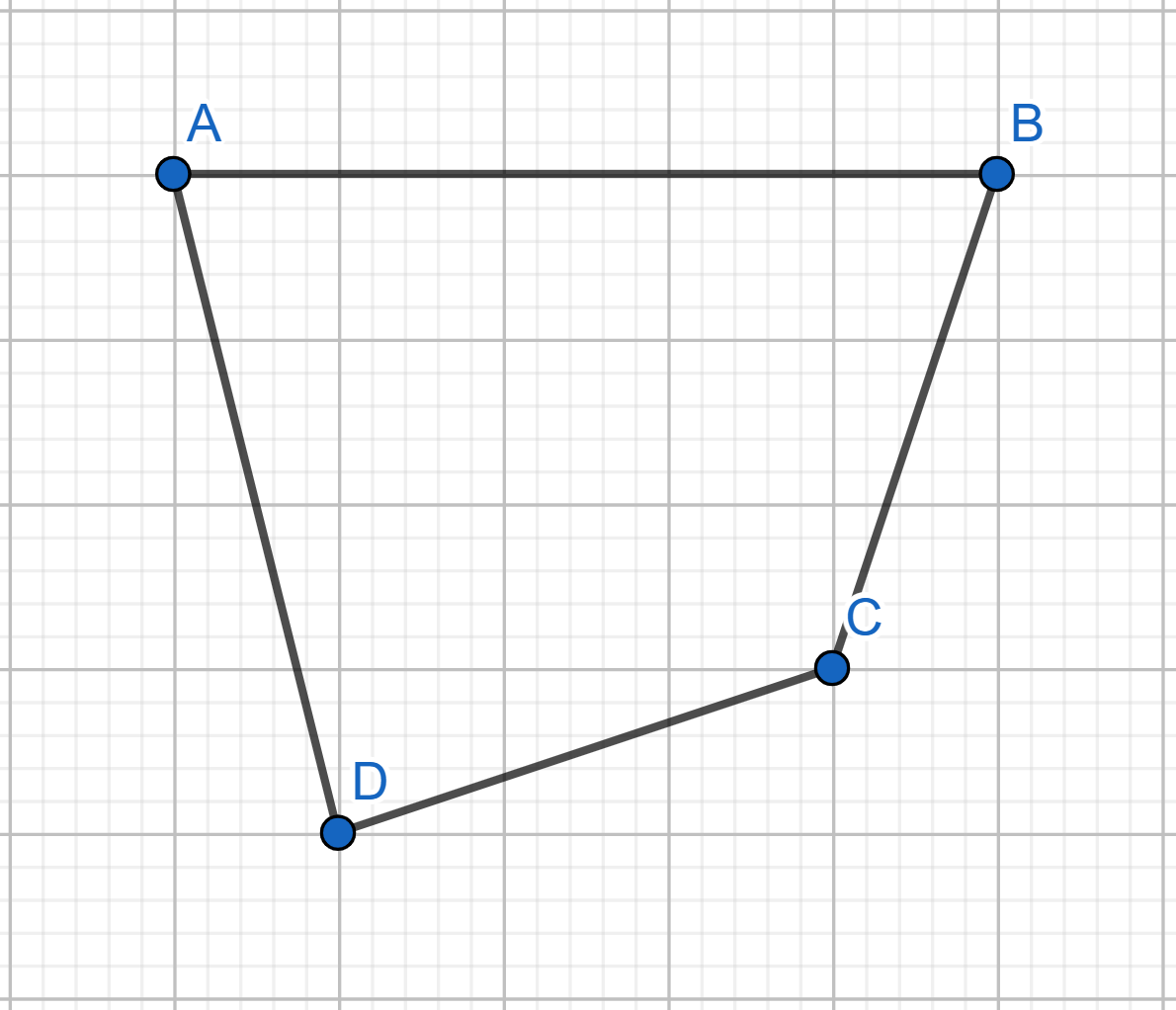
Show how to cover the plane with convex quadrilaterals.
Draw how to tile the whole plane with figures, made from squares \(1\times 1\), \(2\times 2\), \(3\times 3\), and \(4\times 4\), where squares are used the same amount of times in the design of the figure.
Draw the plane tiling with:
squares;
rectangles \(1\times 3\);
regular triangles;
regular hexagons.