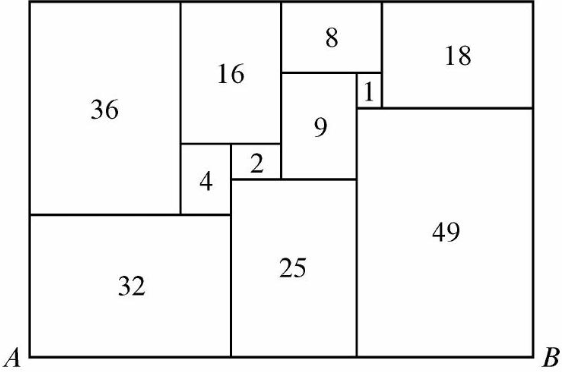
Problems
Today we will discover some ideas related to non-isosceles triangles. This restriction comes from the fact that in isosceles triangles, a median and a height coincide.
With a pile of four cards, does reversing the order of the pile by counting the cards out one by one leaves no card in its original position?
You have in your hands a royal flush! That is, Ace, King, Queen, Jack and \(10\) of spades. How many shuffles of your hand swap the Ace and Jack?
You have a row of coins and you can perform these three operations as many times as you like:
Remove three adjacent heads
Remove two adjacent tails
If there’s a tail followed by a heads, then turn the tail over and put a new tail after both heads
You apply these operations until you can’t make any more moves. Show that you will always get the same configuration at the end, no matter the order.
Let \(a\) be a positive integer, and let \(p\) be a prime number. Prove that \(a^p - a\) is a multiple of \(p\).
We ‘typically’ use the formula \(\frac{1}{2}bh\) for the area of a triangle, where \(b\) is the length of the base, and \(h\) is the perpendicular height. Here’s another one, called Heron’s formula.
Call the sides of the triangle \(a\), \(b\) and \(c\). The perimeter is \(a+b+c\). We call half of this the semiperimeter, \(s=\frac{a+b+c}{2}\). Then the area of this triangle is \[\sqrt{s(s-a)(s-b)(s-c)}.\] Prove this formula is correct.
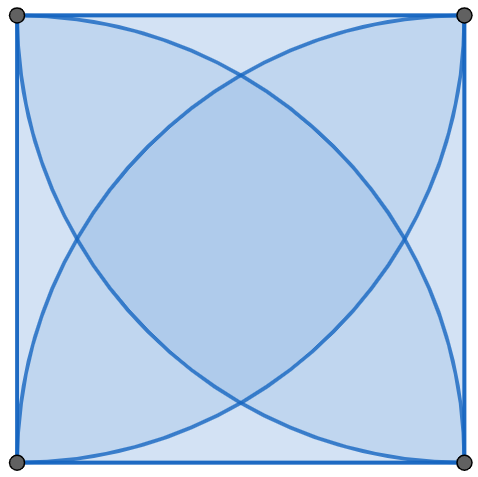
We have a square of side length 1. At each vertex of the square, we draw a circle of radius 1. What is the area bounded by all four circles?
Today we will be finding the areas of some geometric figures. Here is a brief reminder of how to calculate the area of common shapes.
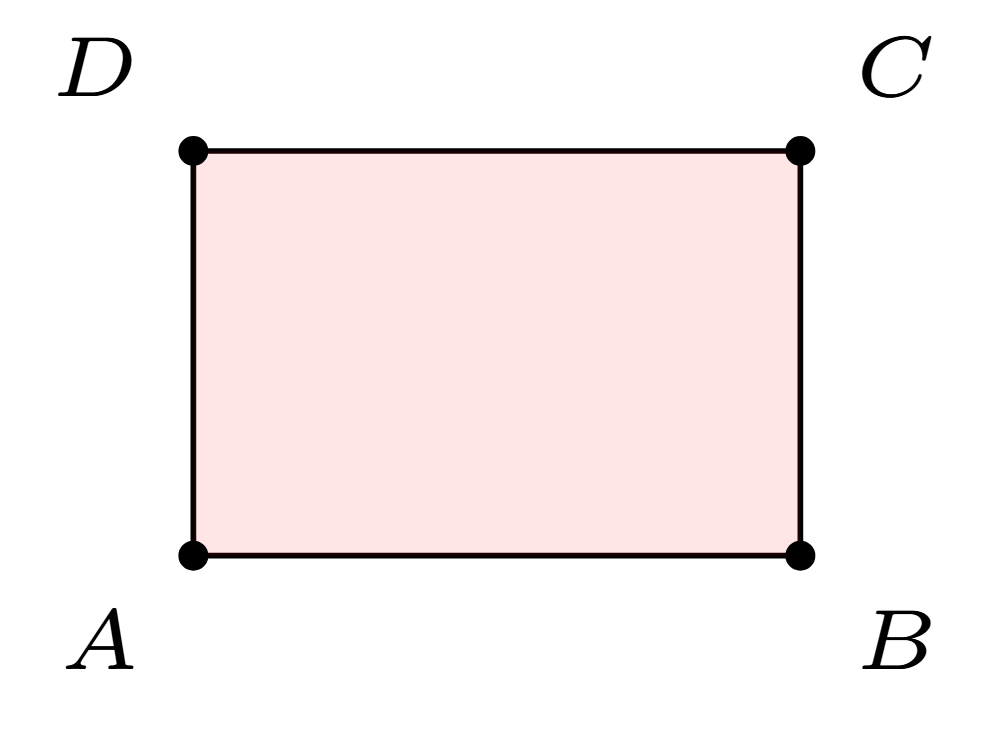
In the picture below, the area of the rectangle is \(|AB|\times |AD|\).
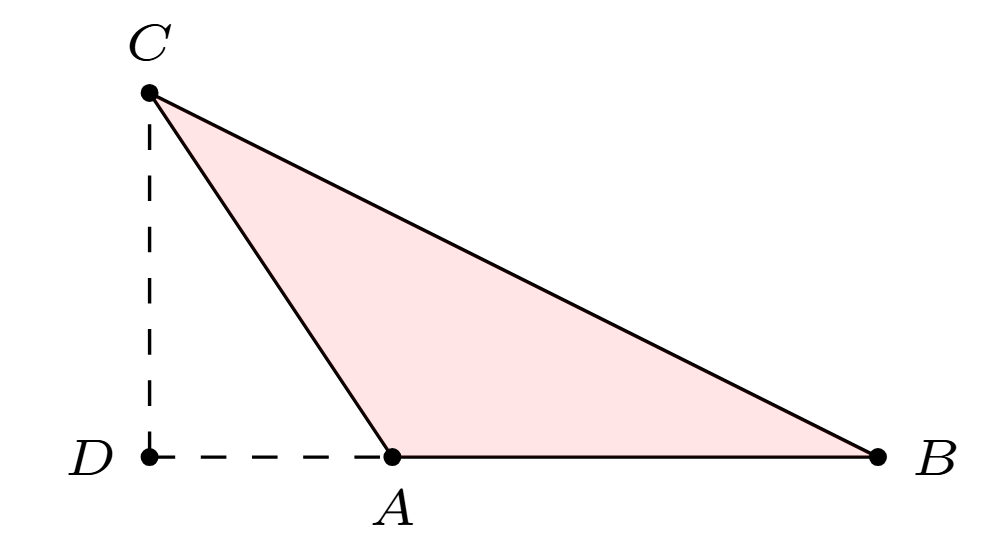
The area of a triangle is given by \(\frac{1}{2}bh\), where \(b\) is the length of a chosen base and \(h\) is the height. In this case, the segment \(AB\) is the base and \(CD\) is the altitude corresponding to the base \(AB\).
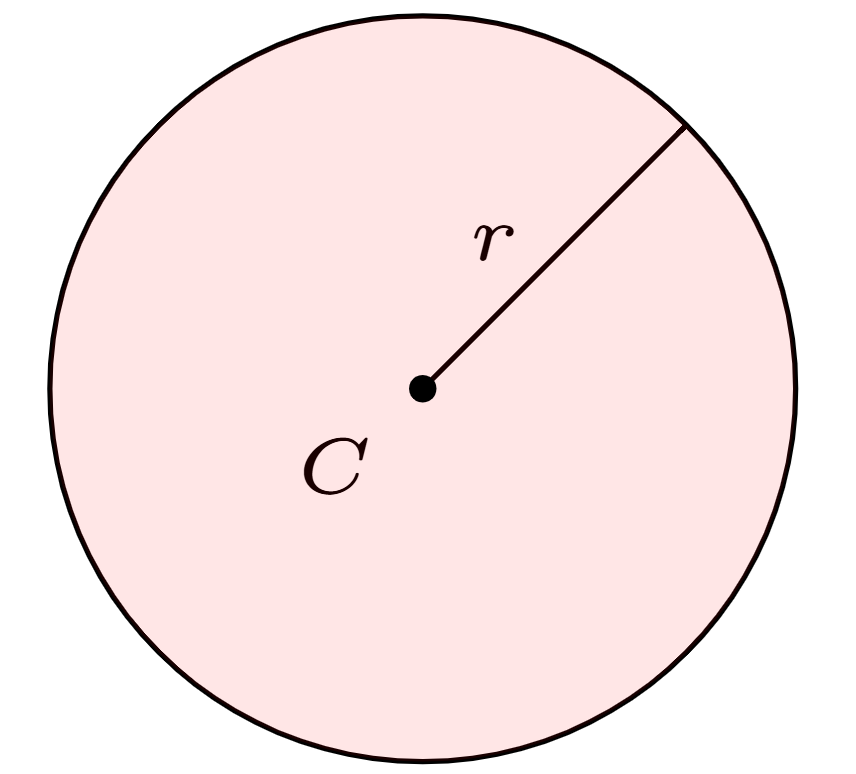
The area of a circle with radius \(r\) is \(\pi r^2\). The number \(\pi\) is approximately 3.14159 to five decimal points.
A simple polygon is a polygon that does not intersect itself and has no holes. Suppose we have a simple polygon \(S\) whose vertices consists of only integer coordinates.
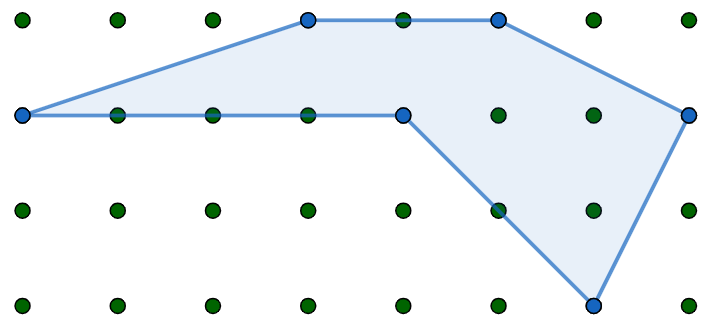
The area turns out to be remarkably easy to calculate. Count up the number of points with integer coordinate inside the polygon and on the boundary; call them \(i\) and \(b\) respectively. The area is then \[A(S) = i+\frac{b}{2}-1.\]
In the picture above, \(i=3\) and \(b=11\), so \(A(S) = \frac{15}{2}\). Prove that this formula for the area \(A(S)\) is correct.