Problems

Let’s prove that any \(90^{\circ}\) angle is equal to any angle larger than \(90^{\circ}\). On the diagram

We have the angle \(\angle ABC = 90^{\circ}\) and angle \(\angle BCD> 90^{\circ}\). We can choose a point \(D\) in such a way that the segments \(AB\) and \(CD\) are equal. Now find middles \(E\) and \(G\) of the segments \(BC\) and \(AD\) respectively and draw lines \(EF\) and \(FG\) perpendicular to \(BC\) and \(AD\).
Since \(EF\) is the middle perpendicular to \(BC\) the triangles \(BEF\) and \(CEF\) are equal which implies the equality of segments \(BF\) and \(CF\) and of angles \(\angle EBF = \angle ECF\), the same about the segments \(AF=FD\). By condition we have \(AB=CD\), thus the triangles \(ABF\) and \(CDF\) are equal, thus \(\angle ABF = \angle DCF\). But then we have \[\angle ABE = \angle ABF + \angle FBE = \angle DCF + \angle FCE = \angle DCE.\]

Nick has written in some order all the numbers \(1,2,...33\) at the vertices of a regular \(33\)-gon. His little sister Hannah assigned to each side of the \(33\)-gon the number equal to the sum of the numbers at the ends of that side. It turns out that Hannah obtained \(33\) consecutive numbers in certain order. Can you find an arrangement of numbers as written by Nick which lead to this situation?

Jess and Tess are playing a game colouring points on a blank plane. Jess is moving first, she picks a non-colored point on a plane and colours it red. Then Tess makes a move, she picks \(2022\) colourless points on the plane and colours them all green. Jess then moves again, and they take turns. Jess wins if she manages to create a red equilateral triangle on the plane, Tess is trying to prevent that from happening. Will Jess always eventually win?

Can you cover a \(10 \times 10\) board using only \(T\)-shaped tetrominos?

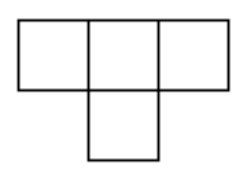
Can you cover a \(10 \times 10\) square with \(1 \times 4\) rectangles?

Two opposite corners were removed from an \(8 \times 8\) chessboard. Is it possible to cover this chessboard with \(1 \times 2\) rectangular blocks?

One unit square of a \(10 \times 10\) square board was removed. Is it possible to cover the rest of it with \(3\)-square \(L\)-shaped blocks?

A \(7 \times 7\) square was tiled using \(1 \times 3\) rectangular blocks in such a way that one of the squares has not been covered. Find all the squares that could be left without being covered.

Can you cover a \(13 \times 13\) square using \(2 \times 2\) and \(3 \times 3\) squares?

Is it possible to cover a \(10 \times 10\) board with the \(L\)-tetraminos without overlapping? The pieces can be flipped and turned.