Problems

If \(n\) is a positive integer, we denote by \(s(n)\) the sum of the divisors of \(n\). For example, the divisors of \(n=6\) are \(1,2,3,6\), so \(s(6)=1+2+3+6=12\). Prove that, for all \(n\geq1\), \[s(1)+s(2)+\cdots+s(n)\leq n^2.\] Denote by \(t(n)\) is instead the sum of the squares of the divisors of \(n\) (e.g., \(t(6)=1^2+2^2+3^2+6^2=50\)), can you find a similar inequality for \(t(n)\)?

Consider the following sum: \[\frac1{1 \times 2} + \frac1{2 \times 3} + \frac1{3 \times 4} + \dots\] Show that no matter how many terms it has, the sum will never be larger than \(1\).

Cut a \(7\times 7\) square into \(9\) rectangles, out of which you can construct any rectangle whose sidelengths are less than \(7\). Show how to construct the rectangles.

There are \(16\) cities in the kingdom. Prove that it is not possible to build a system of roads in such a way that one can get from any city to any other without passing through more than one city on the way, and with at most four roads coming out of each city.

There are \(16\) cities in the kingdom. Prove that it is possible to build a system of roads in such a way that one can get from any city to any other without passing through more than one city on the way, and with at most five roads coming out of each city.

Recall that a line is tangent to a circle if they have only one point of intersection, a circle is called inscribed in a polygon if it is tangent to every side as a segment of that polygon.
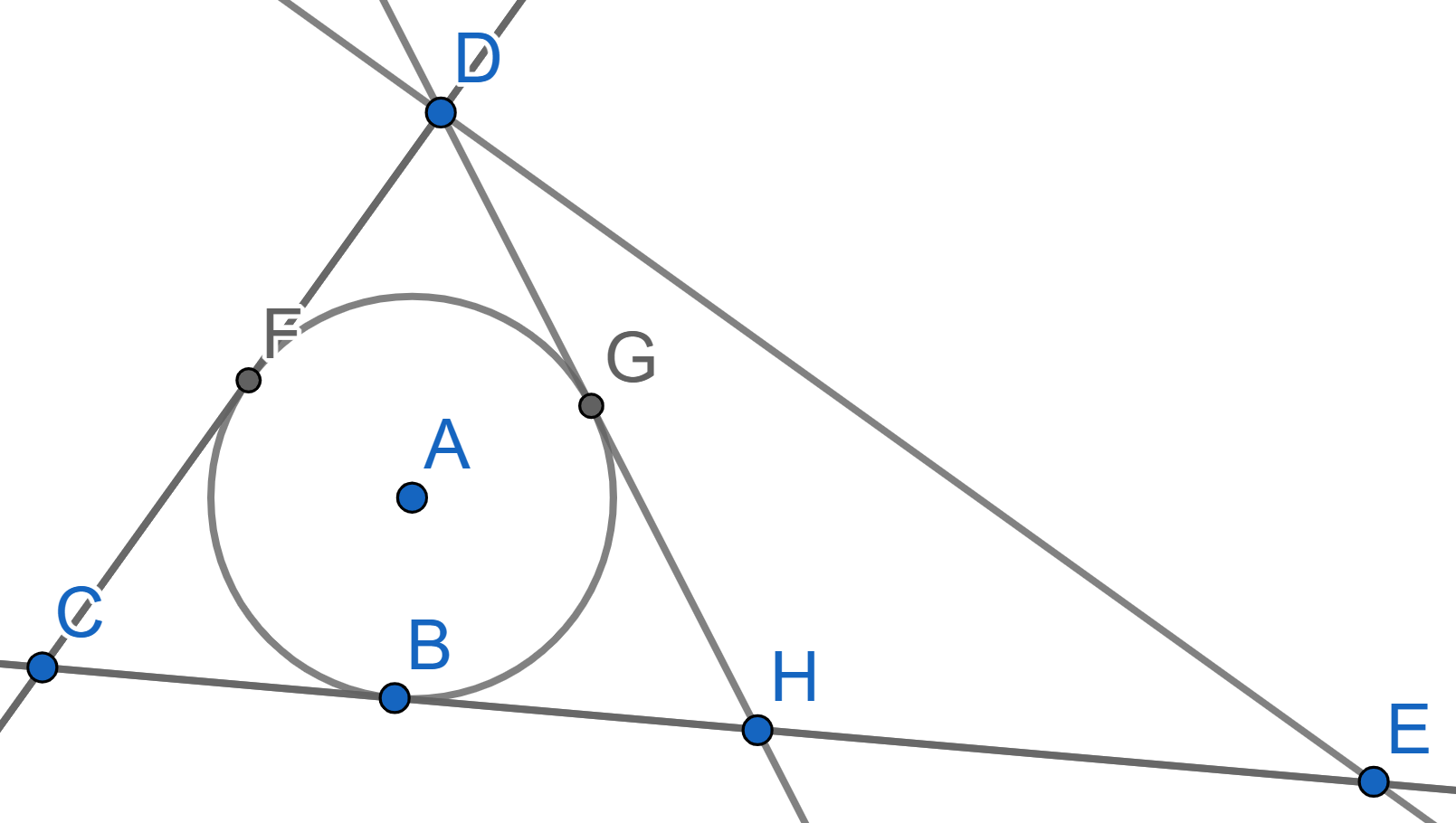
In the triangle \(CDE\) the angle \(\angle CDE = 90^{\circ}\) and the line \(DH\) is the median. A circle with center \(A\) is inscribed in the triangle \(CDH\) and is tangent to the segment \(DH\) in its middle, let’s denote it as \(G\), so \(GH=DG\). Find the angles of the triangle \(CDE\).

A circle with center \(A\) is inscribed into a square \(CDFE\). A line \(GH\) intersects the sides \(CD\) and \(CE\) of the square and is tangent to the circle at the point \(I\). Find the perimeter of the triangle \(CHG\) (the sum of lengths of all the sides) if the side of the square is \(10\)cm.

Recall that a line is tangent to a circle if they have only one point of intersection, a circle is called inscribed in a polygon if it is tangent to every side as a segment of that polygon.
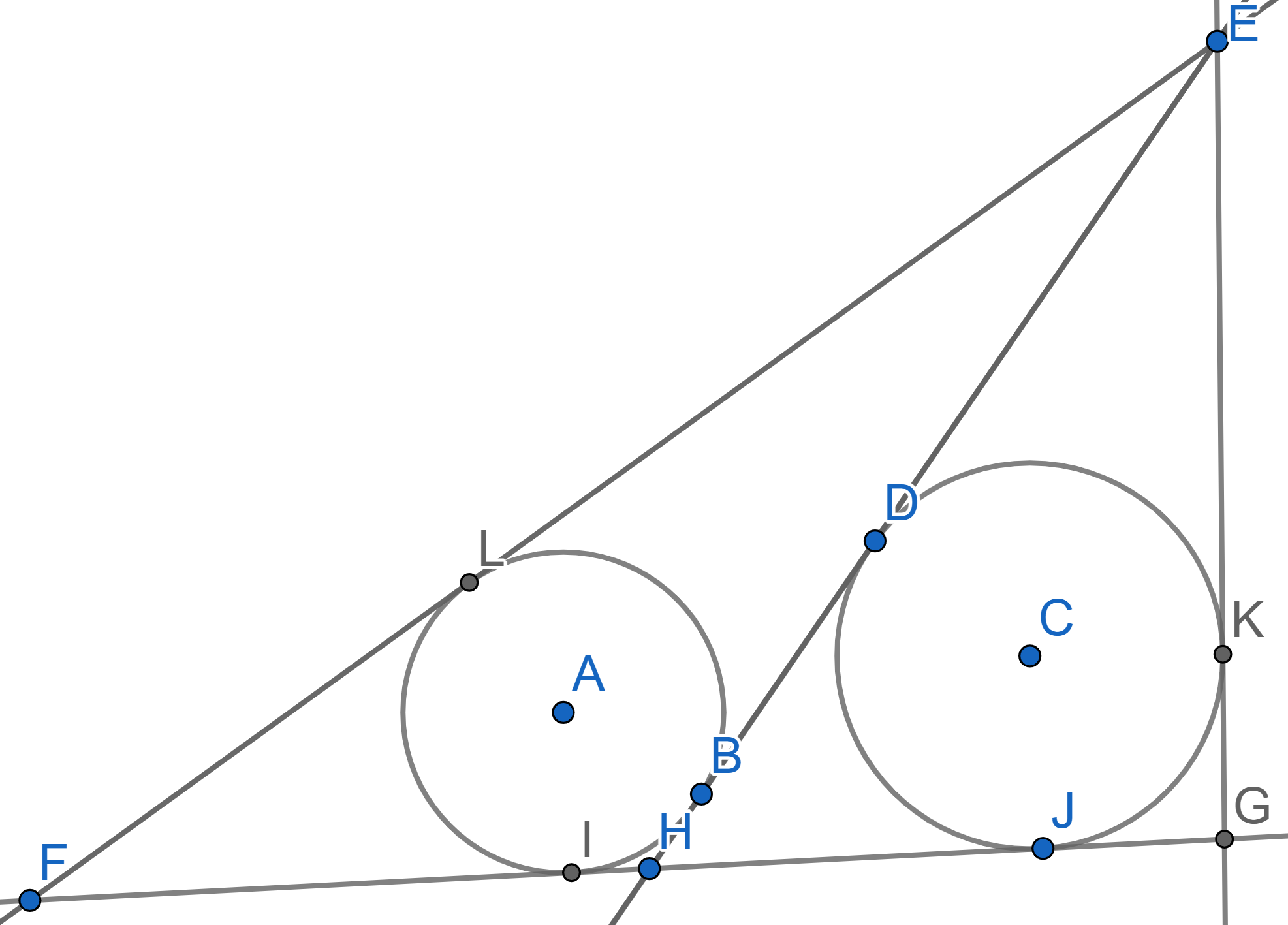
In the triangle \(EFG\) the line \(EH\) is the median. Two circles with centres \(A\) and \(C\) are inscribed into triangles \(EFH\) and \(EGH\) respectively, they are tangent to the median \(EH\) at the points \(B\) and \(D\). Find the length of \(BD\) if \(EF-EG=2\).

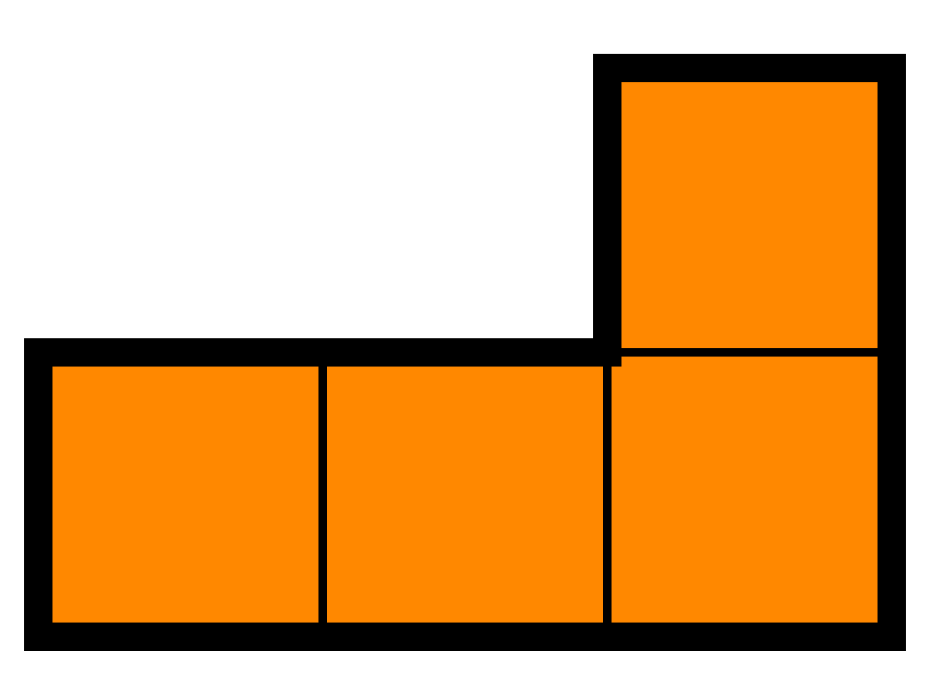
Is it possible to cover a \(6 \times 6\) board with the \(L\)-tetraminos without overlapping? The pieces can be flipped and turned.

Is it possible to cover a \((4n+2) \times (4n+2)\) board with the \(L\)-tetraminos without overlapping for any \(n\)? The pieces can be flipped and turned.