Problems
Show that \(\text{Nim}(x,y,z)\) is a losing position if and only if \(x \oplus y \oplus z = 0\). Remember that \(x \oplus y\) denotes the nim-sum of \(x\) and \(y\).
Is \(\text{Nim}(7,11,15)\) a winning position or a losing position? If it is a winning position, what is the optimal move?
Show that \(\text{Nim}(x_1,\dots,x_k)\) is an losing position if and only if \(x_1 \oplus \dots \oplus x_k = 0\). \(x \oplus y\) denotes the nim-sum of \(x\) and \(y\).
Imagine the Earth is a perfectly round solid ball. Let us drill from the North Pole, London and Beijing simultaneously and meet at the centre of Earth. A ball with three openings is formed. The surface of this ball is shown on the left of the picture below. Describe how to stretch this surface so that it looks like the surface of a donut with two holes as shown on the right.
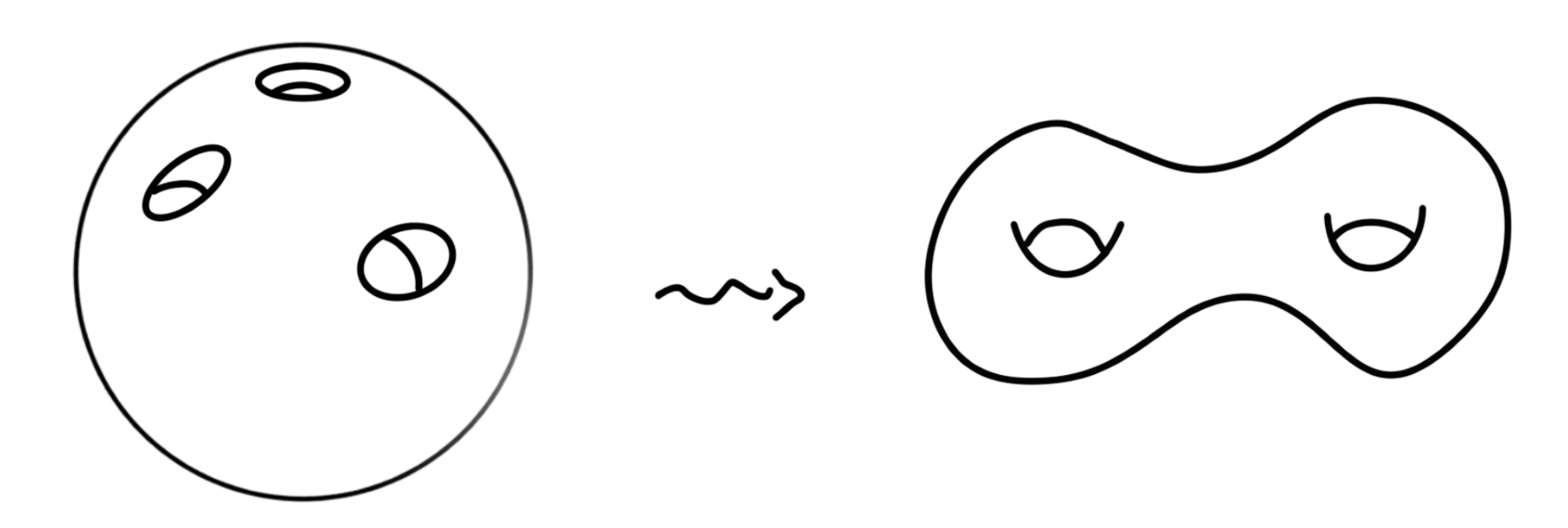
Show that there are no rational numbers \(a,b\) such that \(a^2 + b^2 = 3\).
There are infinitely many couples at a party. Each pair is separated to form two queues of people, where each person is standing next to their partner. Suppose the queue on the left has the property that every nonempty collection of people has a person (from the collection) standing in front of everyone else from that collection. A jester comes into the room and joins the right queue at the back after the two queues are formed.
Each person in the right queue would like to shake hand with a person in the left queue. However, no two of them would like to shake hand with the same person in the left queue. If \(p\) is standing behind \(q\) in the right queue, \(p\) will only shake hand with someone standing behind \(q\)’s handshake partner. Show that it is impossible to shake hands without leaving out someone from the left queue.
Suppose \(x,y\) are real numbers such that \(x < y + \varepsilon\) for every \(\varepsilon > 0\). Show that \(x \leq y\).
How many ways can the numbers \(1,1,1,1,1,2,3,\dots,9\) be listed in such a way that none of the \(1\)’s are adjacent? The number 1 appears five times and each of \(2\) to \(9\) appear exactly once.
John’s local grocery store sells 7 kinds of vegetable, 7 kinds of meat, 7 kinds of grains and 7 kinds of cheese. John would like to plan the entire week’s dinners so that exactly one ingredient of each type is used per meal and no ingredients repeat during the week. How many ways can John plan the dinners?
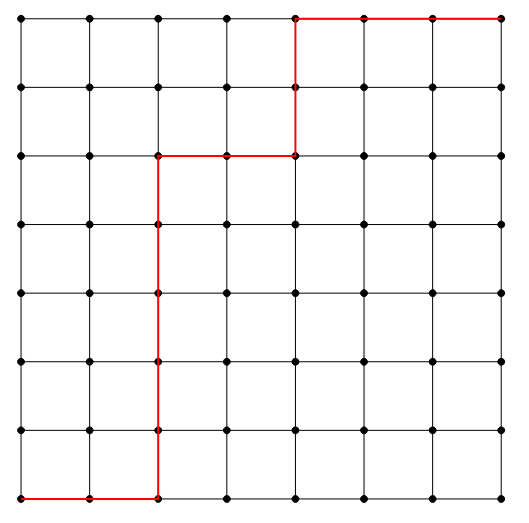
Suppose there is an \(7 \times 7\) grid. We would like to travel from the bottom left corner to the top right corner in exactly 14 steps. A step is from one point on the grid to another point via a segment of length 1. How many paths are there? The picture below shows one possible path on the grid.