Problems
Find a formula for \(R(2,k)\), where \(k\) is a natural number.
Show that \(R(4,3)\ge9\). That is, there exists a way of colouring the edges of \(K_8\) with no red \(K_4\), nor any blue \(K_3\).
Show that \(R(4,4)\ge18\) - that is, there’s a way of colouring the edges of \(K_{17}\) such that there’s no monochromatic \(K_4\).
Show that \(R(4,3)\le9\). That is, no matter how you colour the edge of \(K_9\), there must be a red \(K_4\) or a blue \(K_3\).
Show that \(R(4,4)\le18\) - that is, no matter how you colour the edges of \(K_{18}\), there must be a monochromatic \(K_4\).
By considering \(k-1\) copies of \(K_{k-1}\), show that \(R(k,k)\ge(k-1)^2\).
Let \(s>2\) and \(t>2\) be integers. Show that \(R(s,t)\le R(s-1,t)+R(s,t-1)\).
Using \(R(s,t)\le R(s-1,t)+R(s,t-1)\), prove that \(R(k,k)\le 4^k\).
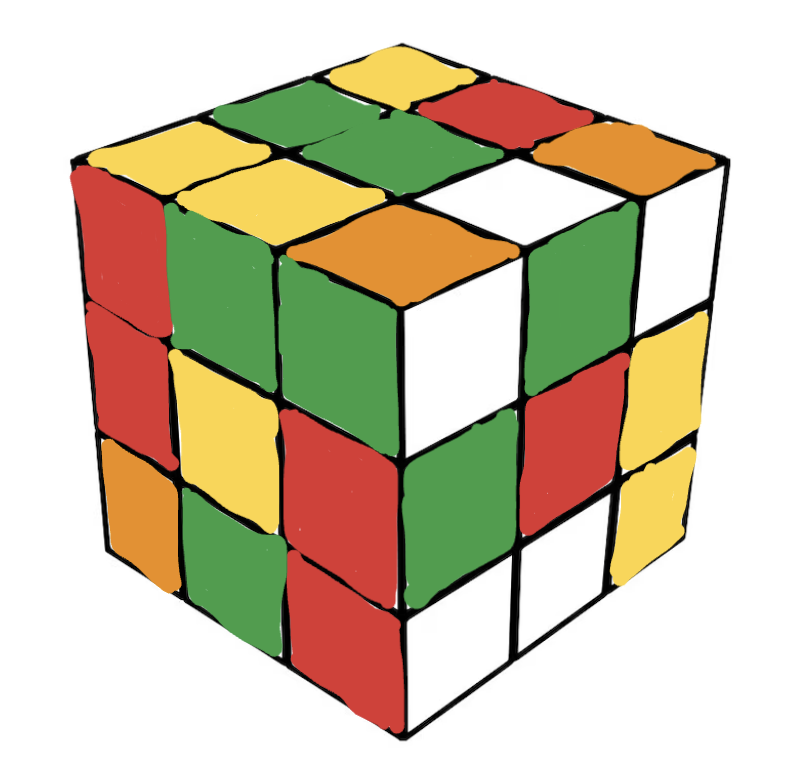
Explain why you can’t rotate the sides on a normal Rubik’s cube to get to the following picture (with no removing stickers, painting, or other cheating allowed).