Problems
Draw the plane tiling with:
squares;
rectangles \(1\times 3\);
regular triangles;
regular hexagons.
Draw the plane tiling using trapeziums of the following shape:

Here the sides \(AB\) and \(CD\) are parallel.
For any triangle, prove you can tile the plane with that triangle.
Prove that one cannot tile the whole plane with regular pentagons.
Draw the plane tiling using convex hexagons with parallel and equal opposite sides.
Draw how to tile the whole plane with figures, consisting of squares \(1\times 1\), \(2\times 2\), \(3\times 3\), \(4\times 4\), \(5\times 5\), and \(6\times 6\), where each square appears an equal number of times in the design of the figure. Can you think of two essentially different ways to do this?
Find a non-regular octagon which you can use to tile the whole plane and show how to do that.
Observe that \(14\) isn’t a square
number but \(144=12^2\) and \(1444=38^2\) are both square numbers. Let
\(k_1^2=\overline{a_n...a_1a_0}\) the
decimal representation of a square number.
Is it possible that \(\overline{a_n...a_1a_0a_0}\) and \(\overline{a_n...a_1a_0a_0a_0}\) are also
both square numbers?
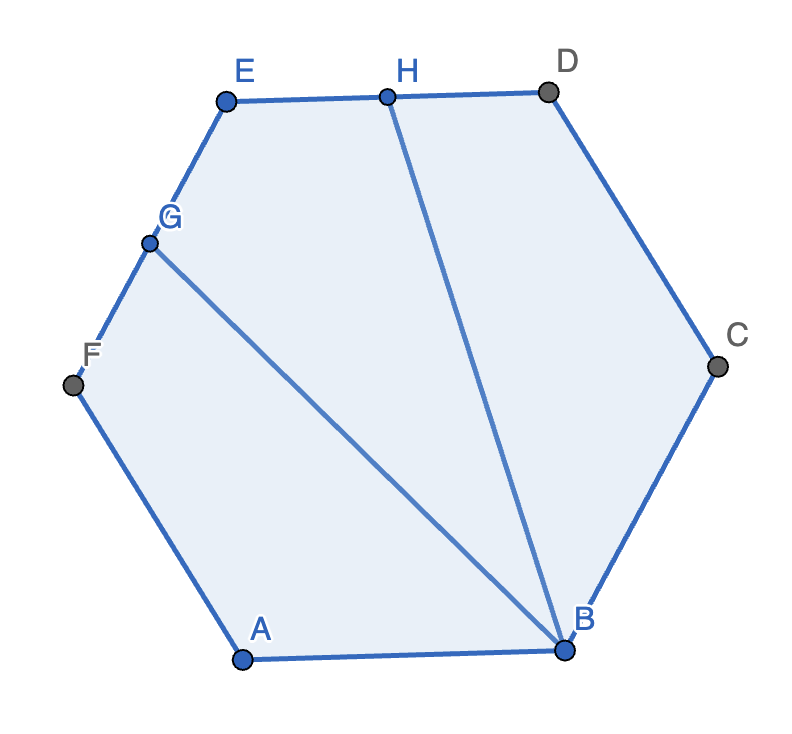
Let \(ABCDEF\) be a regular hexagon. Points \(G\) and \(H\) lie on \(EF\) and \(DE\) respectively such that \(|EG|=|EH|\). Furthermore, the area of quadrilateral \(ABGF\) is equal to the area of quadrilateral \(BGEH\), which are both equal to the area of \(BCDH\). What’s the ratio \(\frac{|EG|}{|EF|}\)?
I’m thinking of a positive number less than \(100\). This number has remainder \(1\) when divided by \(3\), it has remainder \(2\) when divided by \(4\), and finally, it leaves remainder \(3\) when divided by \(5\). What number am I thinking of?