We solve problems

Problemas Geometría Geometría plana Cuadrilátero Cuadriláteros circunscritos

Prove that a convex quadrilateral \(ICEF\) can have a circle inscribed into it if and only if \(IC+EH = CE+IF\).

Problemas Geometría Geometría plana Transformaciones planas Traslación paralela

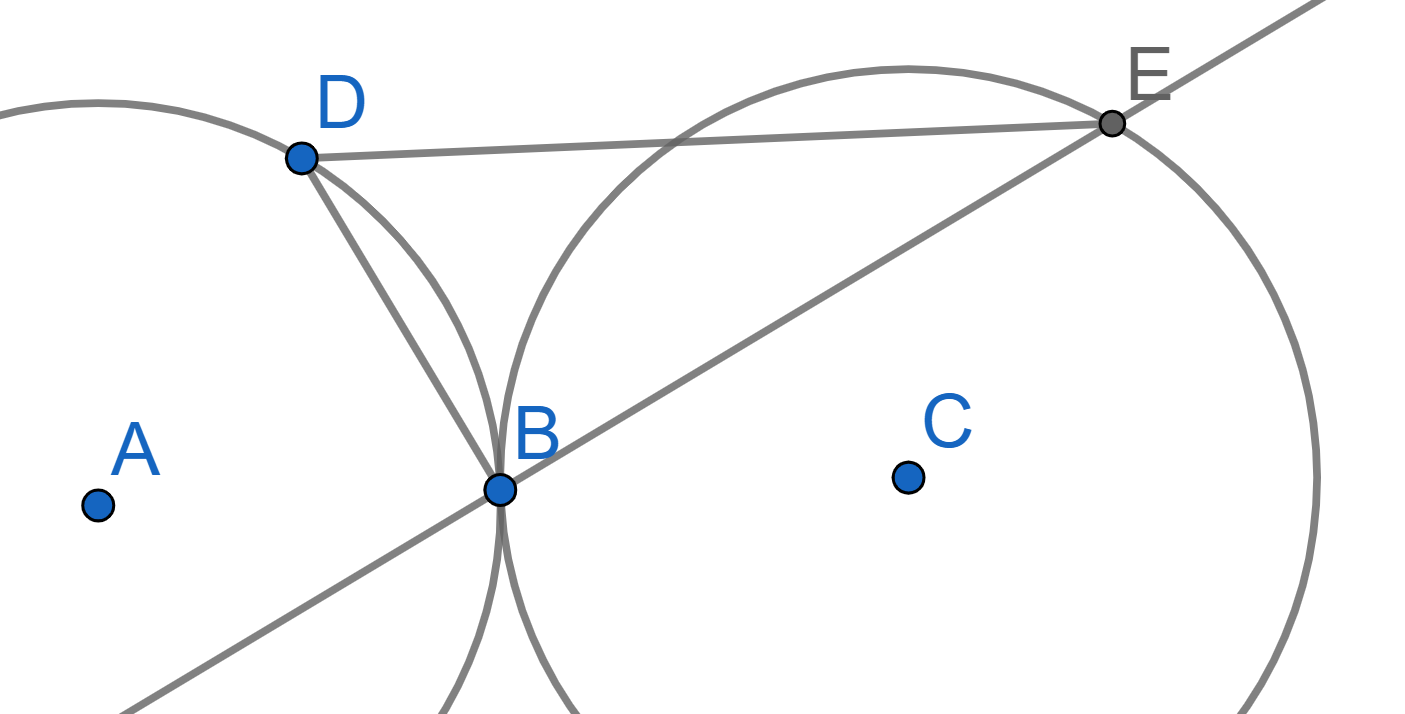
Two circles of radius \(R\) touch at point \(B\). On one of them, point \(D\) is chosen and on the other point \(E\) is chosen. These points have a property of \(\angle DBE = 90^{\circ}\). Prove that \(DE = 2R\).

Problem #PRU-58082

Problemas Geometría Geometría plana Desigualdades geométricas Desigualdades en un triángulo Un segmento dentro del triángulo es menor que el lado más largo, Problemas Geometría Geometría plana Triángulos Tipos de triángulos Triángulos rectángulos Teorema de Pitágoras y su recíproco

12–14 4.0

A \(3\times 4\) rectangle contains 6 points. Prove that amongst them there will be two points, such that the distance between them is no greater than \(\sqrt5\).

Problem #PRU-58084

Problemas Geometría Geometría plana Círculos Círculo, sector, segmento, etc. Métodos Principio del casillero Principio del casillero (ángulos y longitudes) Principio del casillero (número finito de puntos, líneas, etc.)

12–13 4.0

There are 25 points on a plane, and among any three of them there can be found two points with a distance between them of less than 1. Prove that there is a circle of radius 1 containing at least 13 of these points.

Problem #PRU-58085

Problemas Matemáticas discretas Combinatoria Divisiones, particiones, cubiertas y mosaicos Cubiertas Geometría Geometría plana Polígonos Polígonos inscritos y circunscritos Métodos Principio del casillero Principio del casillero (número finito de puntos, líneas, etc.)

13–15 4.0

A unit square contains 51 points. Prove that it is always possible to cover three of them with a circle of radius \(\frac{1}{7}\).

Problem #PRU-58089

Problemas Geometría Geometría plana Figuras convexas y no convexas Polígonos convexos Métodos Principio del palomar Principio del palomar (número finito de puntos, líneas, etc.)

12–15 4.0

What is the minimum number of points necessary to mark inside a convex \(n\)-gon, so that at least one marked point always lies inside any triangle whose vertices are the vertices of the polygon?

Problem #PRU-58094

Problemas Geometría Geometría plana Círculos Ángulo central. Longitud de arco y circunferencia Conexión entre la longitud de arco, el ángulo y el acorde Métodos Principio del casillero Principio del casillero (ángulos y longitudes)

13–15 4.0

Several chords are drawn through a unit circle. Prove that if each diameter intersects with no more than \(k\) chords, then the total length of all the chords is less than \(\pi k\).

Problem #PRU-58101

Problemas Geometría Geometría plana Área Área de un círculo, sector, segmento Cuadrilátero Paralelogramo Casos especiales Rectángulos y cuadrados. Criterios y propiedades

14–15 4.0

A square of side 15 contains 20 non-overlapping unit squares. Prove that it is possible to place a circle of radius 1 inside the large square, so that it does not overlap with any of the unit squares.

Problem #PRU-60299

Problems Teoría de Números Divisibilidad Divisibilidad de un número. Propiedades generales Métodos Métodos algebraicos Particiones en pares y grupos; biyecciones Principio del casillero Principio del casillero (otro)

13–15 4.0

From the set of numbers 1 to \(2n\), \(n + 1\) numbers are chosen. Prove that among the chosen numbers there are two, one of which is divisible by another.

Problem #PRU-60354

Problemas Métodos Principio del casillero Principio del casillero (otro)

13–15 4.0

There are \(2k+1\) cards numbered with the numbers \(1\) to \(2k+1\). What is the largest number of cards that can be chosen so that no number on a chosen card is equal to the sum of two numbers from two other chosen cards?