Problems

Draw how Robinson Crusoe should put pegs and ropes to tie his goat in order for the goat to graze grass in the shape of a given triangle.

Draw a picture how Robinson used to tie the goat and the wolf in order for the goat to graze the grass in the shape of half a circle.

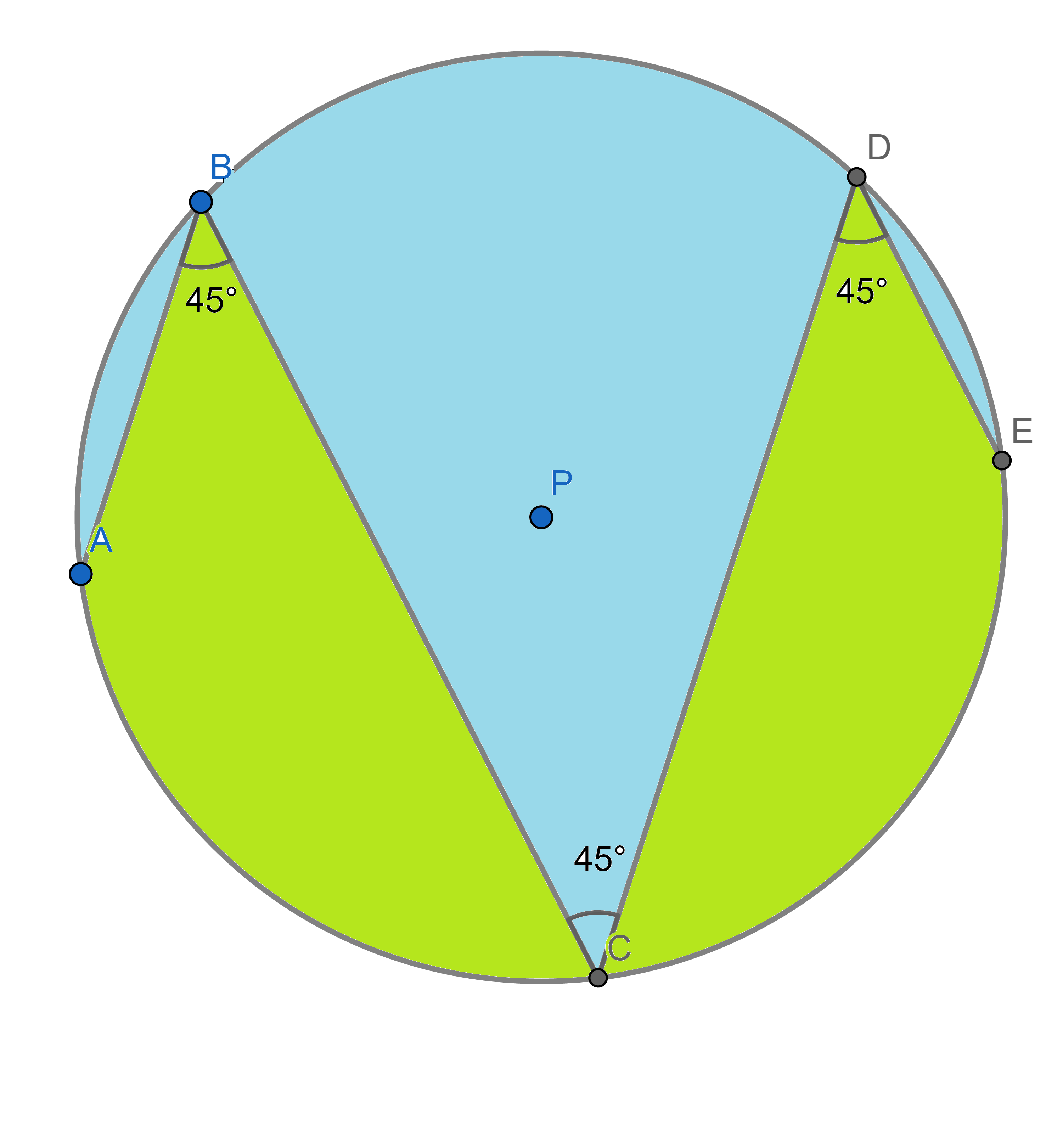
The marked angles are all \(45^{\circ}\). Show that the total green and blue areas are the same.

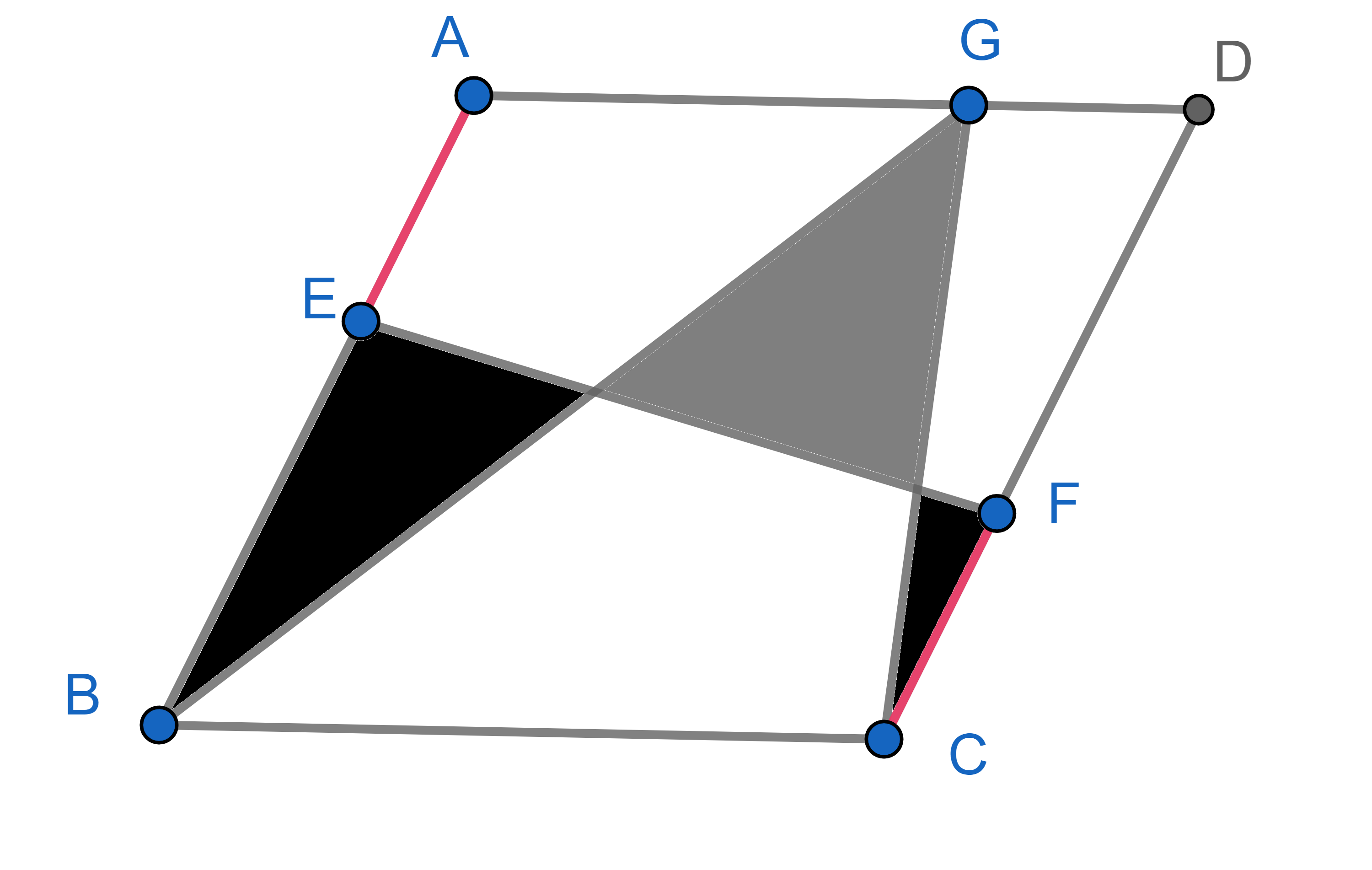
In a parallelogram \(ABCD\), point \(E\) belongs to the side \(AB\), point \(F\) belongs to the side \(CD\) and point \(G\) belongs to the side \(AD\). We know that the marked red segments \(AE\) and \(CF\) have equal lengths. Prove that the total grey area is equal to the total black area.

Jamie’s drawer is pretty big. It has infinitely many crayons. In fact, for every colour of crayon, there are infinitely many crayons of that colour.
In every group of \(9\) randomly chosen crayons from Jamie’s drawer, some \(3\) will have the same colour. Jamie chooses \(25\) crayons at random. Prove that some \(7\) of them will have the same colour.

Suppose \(n \ge 2\) cricket teams play in a tournament. No two teams play each other more than once, and no team plays itself. Prove that some two teams have to play the same number of games.

Find all the prime numbers \(p\) such that there exist natural numbers \(x\) and \(y\) for which \(p^x = y^3 + 1\).

Find all natural numbers \(n\) for which there exist integers \(a,b,c\) such that \(a+b+c = 0\) and the number \(a^n + b^n + c^n\) is prime.

On a \(10\times 10\) board, a bacterium sits in one of the cells. In one move, the bacterium shifts to a cell adjacent to the side (i.e. not diagonal) and divides into two bacteria (both remain in the same new cell). Then, again, one of the bacteria sitting on the board shifts to a new adjacent cell, either horizontally or vertically, and divides into two, and so on. Is it possible for there to be an equal number of bacteria in all cells after several such moves?

There is a scout group where some of the members know each other. Amongst any four members there is at least one of them who knows the other three. Prove that there is at least one member who knows the entirety of the scout group.