Problems
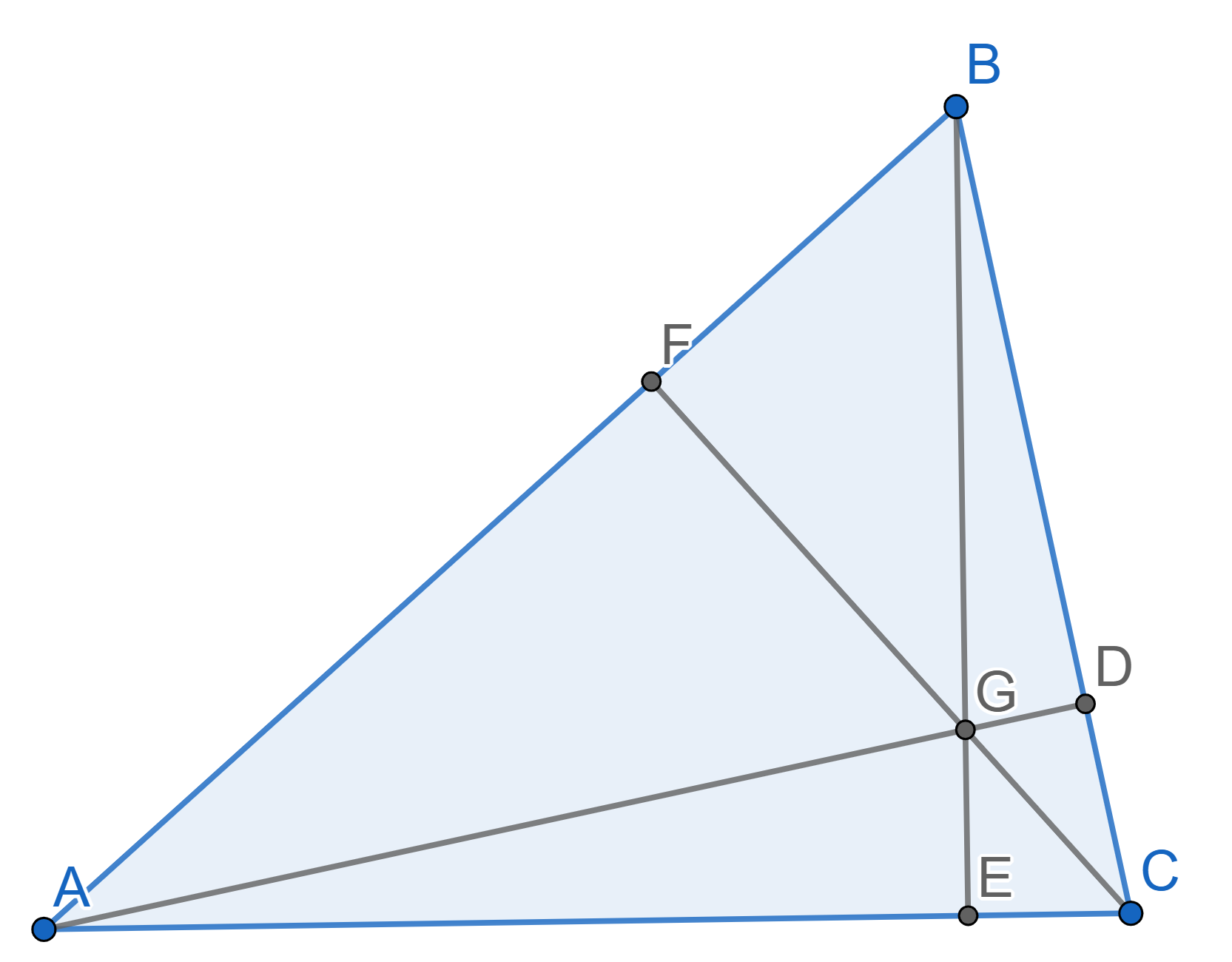
Let \(ABC\) be a non-isosceles
triangle. The point \(G\) is the point
of intersection of the medians \(AE\),
\(BF\), \(CD\). The point \(H\) is the point of intersection of all
heights. The point \(I\) is the center
of the circumscribed circle of \(ABC\),
or the point of intersection of all perpendicular bisectors to the
segments \(AB\), \(BC\), \(AC\).
Prove that points \(I,G,H\) lie on one
line and that the ratio \(IG:GH =
1:2\). The line that all of \(I\), \(G\)
and \(H\) lie on is called the
Euler line of triangle \(ABC\).

Let \(ABC\) be a triangle. Prove that the heights \(AD\), \(BE\), \(CF\) intersect in one point.
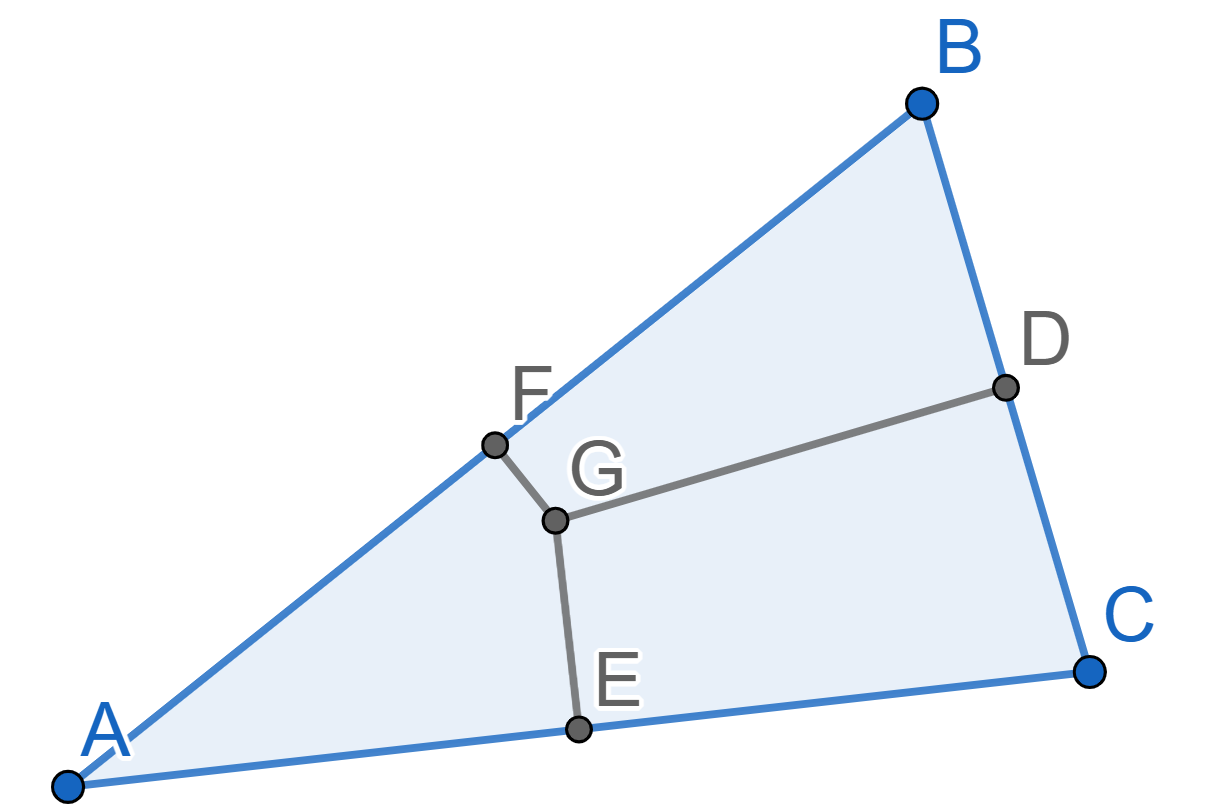
Let \(ABC\) be a triangle. Prove that the medians \(AD\), \(BE\), \(CF\) intersect in one point.
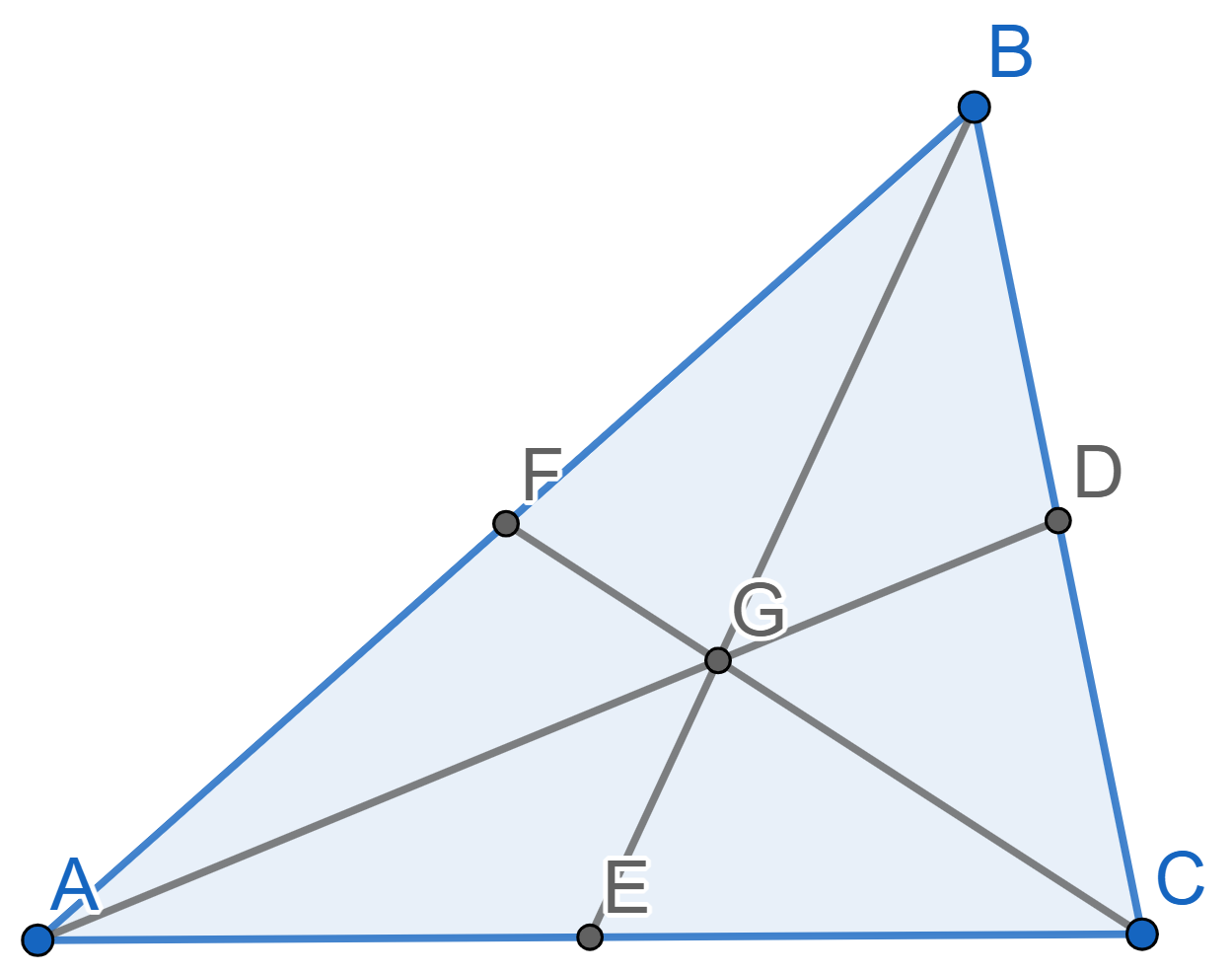
Let \(ABC\) be a triangle with medians \(AD\), \(BE\), \(CF\). Prove that the triangles \(ABC\) and \(DEF\) are similar. What is their similarity coefficient?
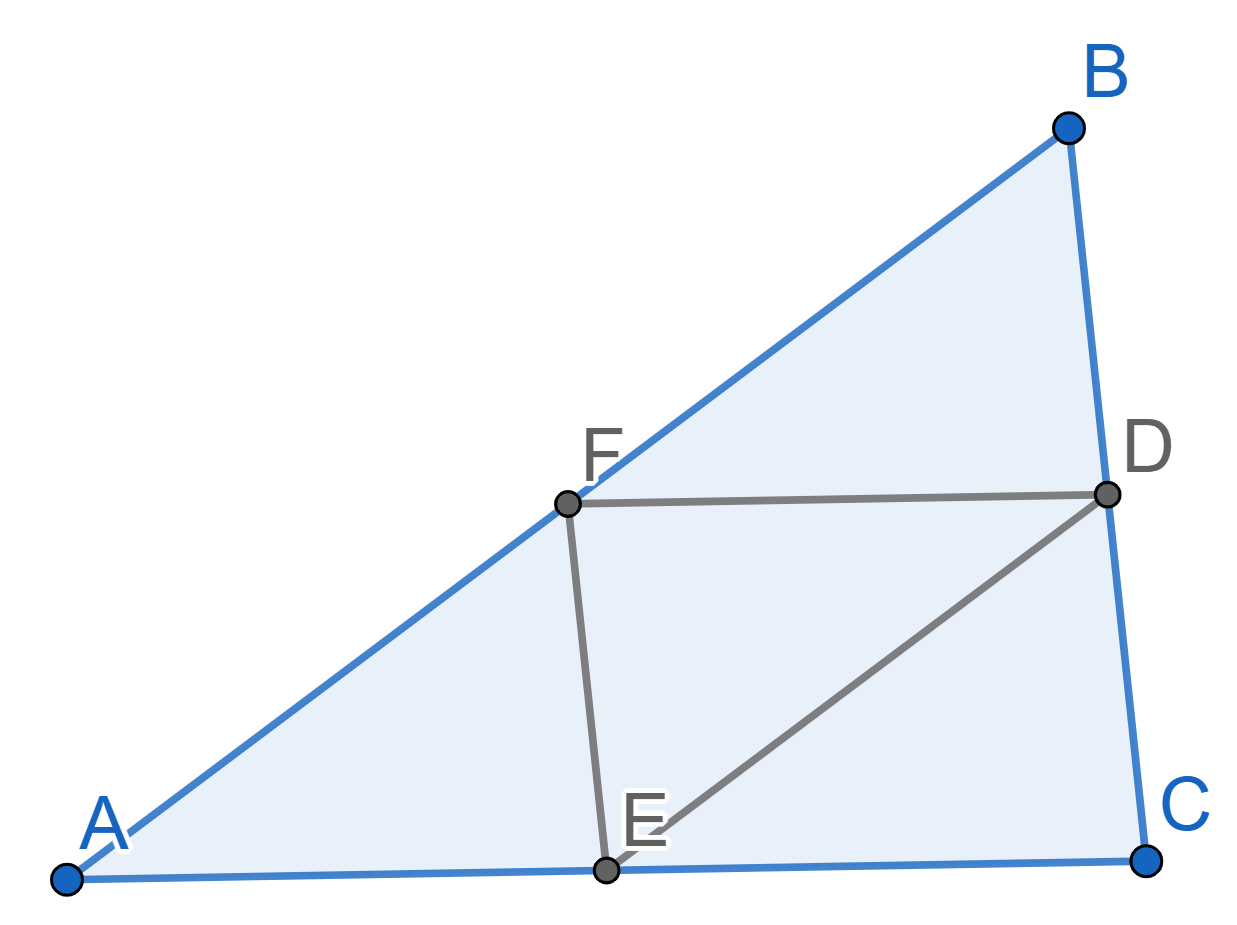
Let \(ABC\) be a triangle with midpoints \(D\) on the side \(BC\), \(E\) on the side \(AC\), and \(F\) on the side \(AB\). Prove that the perpendicular bisectors to the sides \(AB\), \(BC\), \(AC\) intersect at one point.
Let \(ABC\) be a triangle with midpoints \(D\) on the side \(BC\), \(E\) on the side \(AC\), and \(F\) on the side \(AB\). Let \(M\) be the point of intersection of all medians of the triangle \(ABC\), let \(H\) be the point of intersection of the heights \(AJ\), \(BI\) and \(CK\). Prove that the points \(D\), \(J\), \(I\), \(E\), \(F\) and \(K\) all lie on one circle.
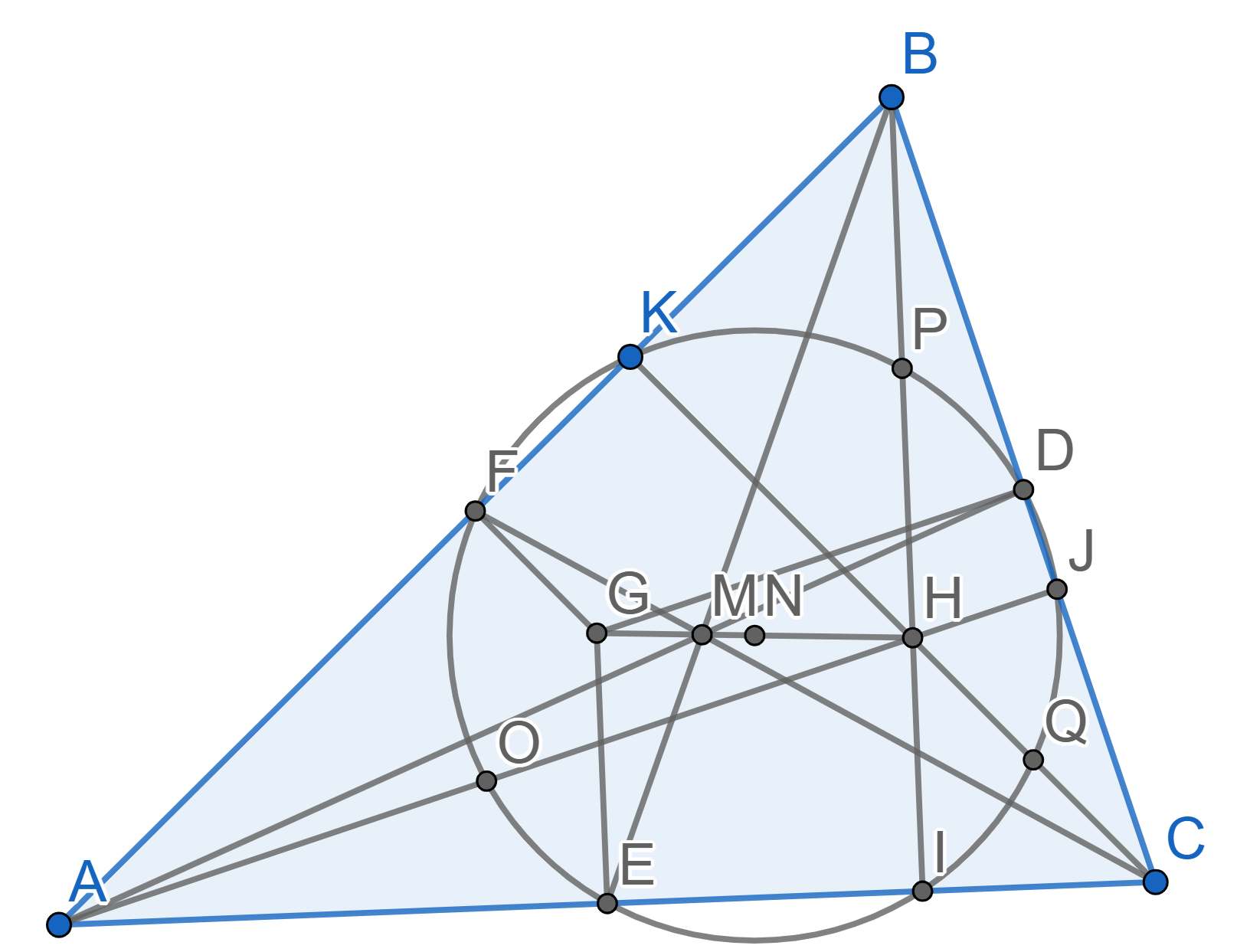
Let \(ABC\) be a triangle with midpoints \(D\) on the side \(BC\), \(E\) on the side \(AC\) and \(F\) on the side \(AB\). Let \(M\) be the point of intersection of all medians of the triangle \(ABC\) and let \(H\) be the point of intersection of the heights \(AJ\), \(BI\) and \(CK\). Consider the Euler circle of the triangle \(ABC\), which is the one that contains the points \(D,J,I,E,F,K\). This circle intersects the segments \(AH\), \(BH\) and \(CH\) at the points \(O\), \(P\) and \(Q\) respectively. Prove that \(O\), \(P\) and \(Q\) are the midpoints of the segments \(AH\), \(BH\) and \(CH\).
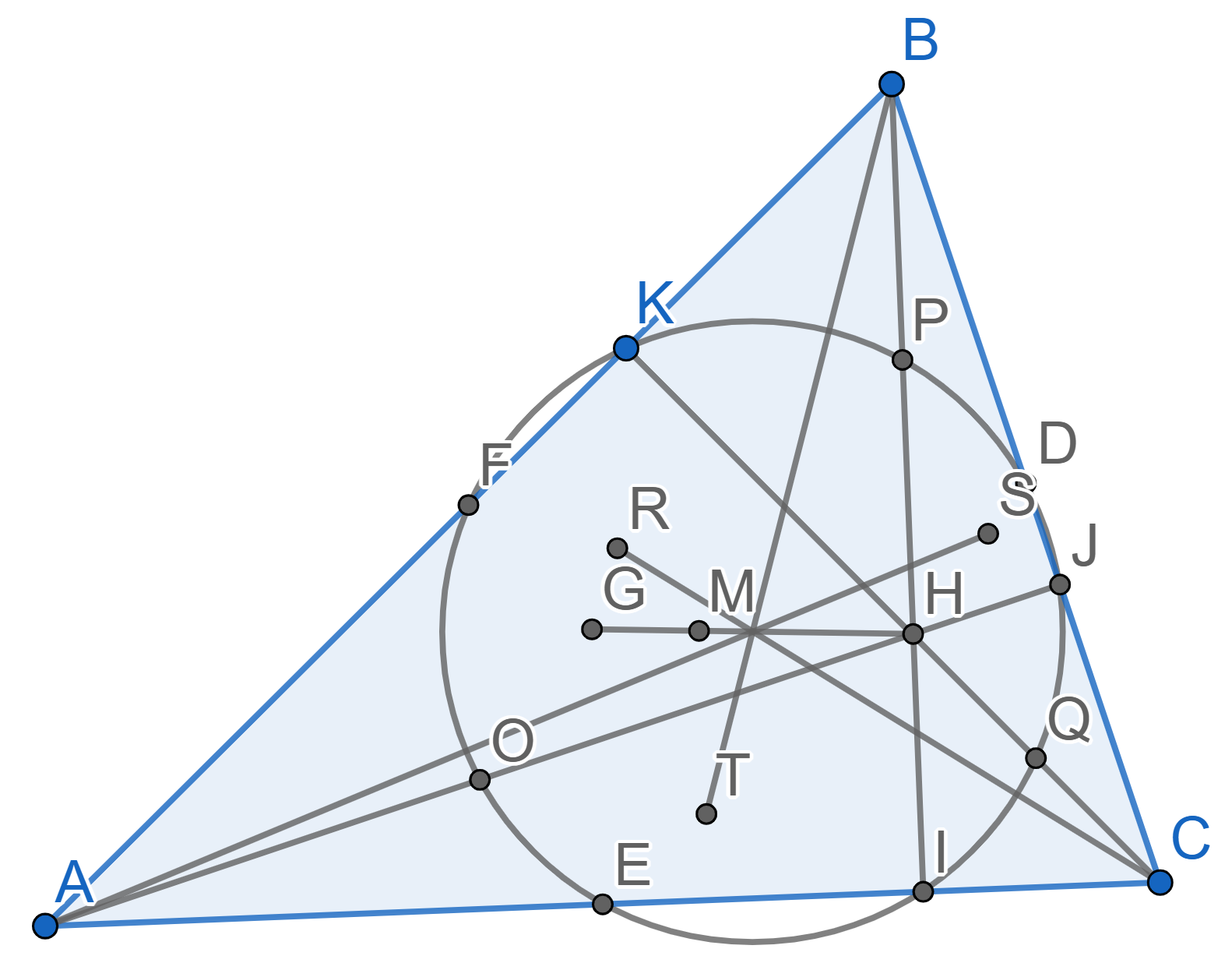
Consider the point \(H\) of intersection of the heights of the triangle \(ABC\). Prove that Euler lines of the triangles \(ABC\), \(ABH\), \(BCH\) and \(ACH\) intersect at one point. On the diagram below the points \(R,S,T\) are the points of intersection of medians in triangles \(ABH\), \(BCH\), and \(ACH\) respectively.
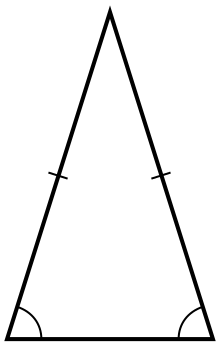
What are the symmetries of an isosceles triangle (which is not equilateral)?