Problems
How can you move the top card to any position in an even size deck using only Faro shuffles?
You have a deck of \(n\) distinct cards. Deal out \(k\) cards from the top one by one and put the rest of the deck on top of the \(k\) cards. What is the minimum number of times you need to repeat the action to return every card back to its position?
Let \(ABC\) be a triangle with midpoints \(D\) on the side \(BC\), \(E\) on the side \(AC\), and \(F\) on the side \(AB\). Prove that the perpendicular bisectors to the sides \(AB\), \(BC\), \(AC\) intersect at one point.
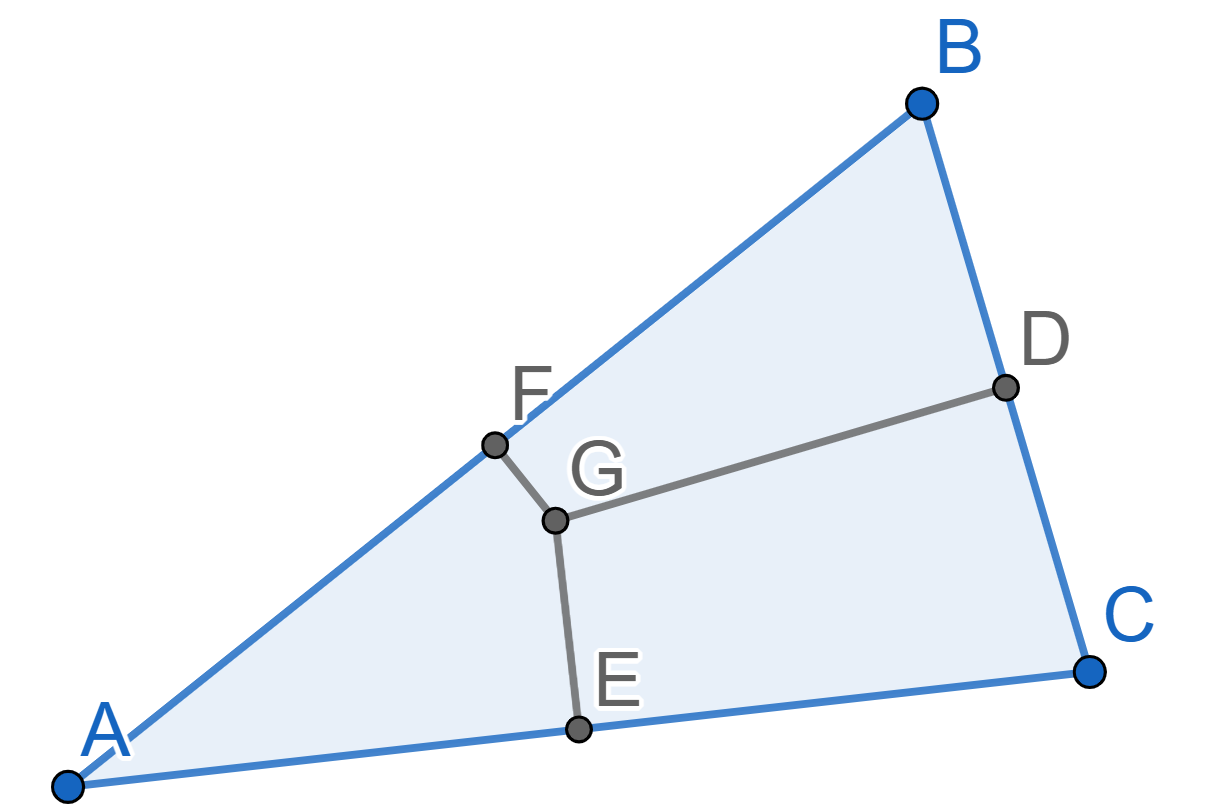
Let \(ABC\) be a triangle with midpoints \(D\) on the side \(BC\), \(E\) on the side \(AC\), and \(F\) on the side \(AB\). Let \(M\) be the point of intersection of all medians of the triangle \(ABC\), let \(H\) be the point of intersection of the heights \(AJ\), \(BI\) and \(CK\). Prove that the points \(D,J,I,E,F,K\) lie on one circle. What can you say about the center of that circle?
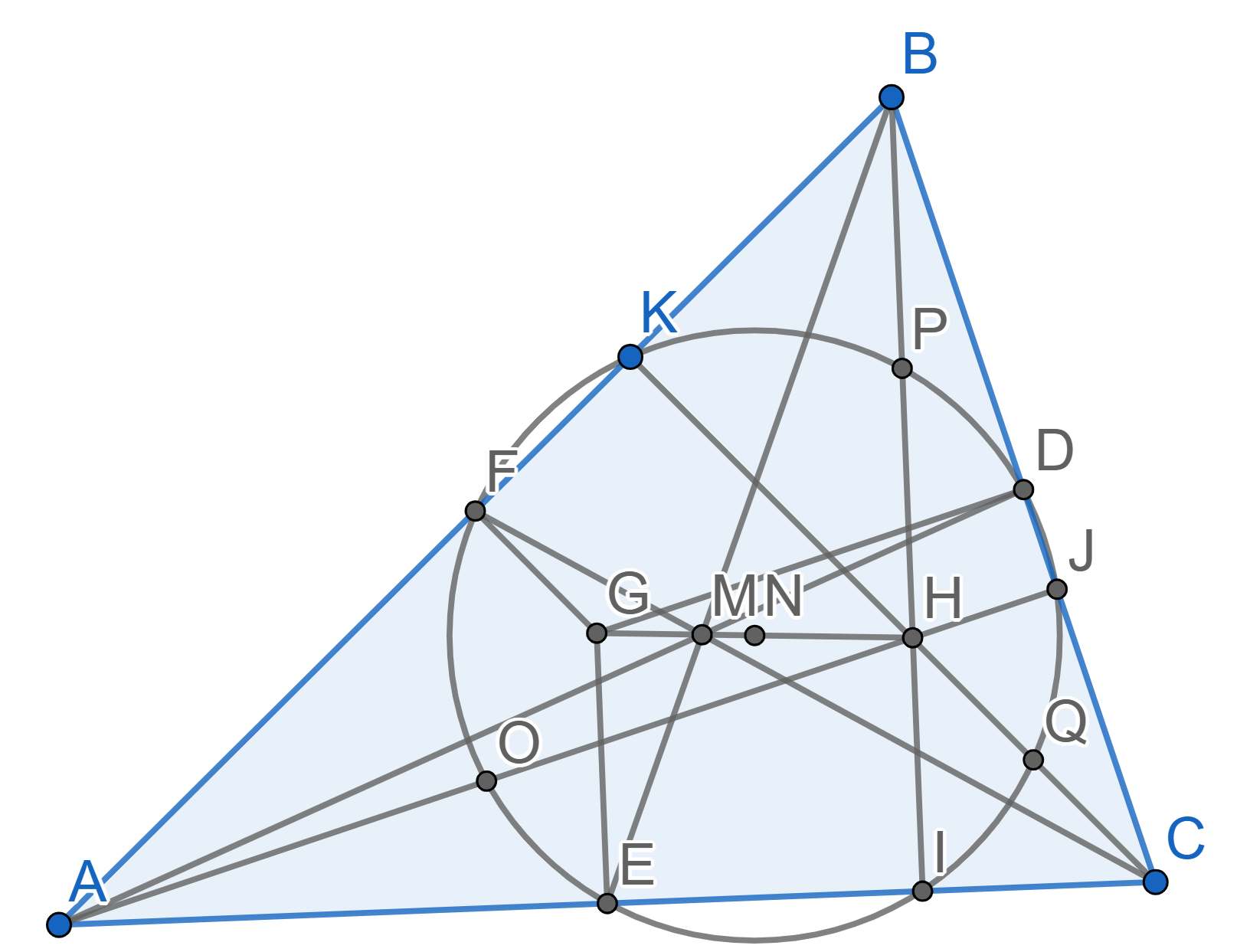
Let \(ABC\) be a triangle with midpoints \(D\) on the side \(BC\), \(E\) on the side \(AC\), and \(F\) on the side \(AB\). Let \(M\) be the point of intersection of all medians of the triangle \(ABC\), let \(H\) be the point of intersection of the heights \(AJ\), \(BI\) and \(CK\). Consider the Euler circle of the triangle \(ABC\), the one that contains the points \(D,J,I,E,F,K\). This circle intersects the segments \(AH\), \(BH\), \(CH\) at points \(O\), \(P\), \(Q\) respectively. Prove that \(O\), \(P\), \(Q\) are the midpoints of the segments \(AH\), \(BH\), \(CH\).
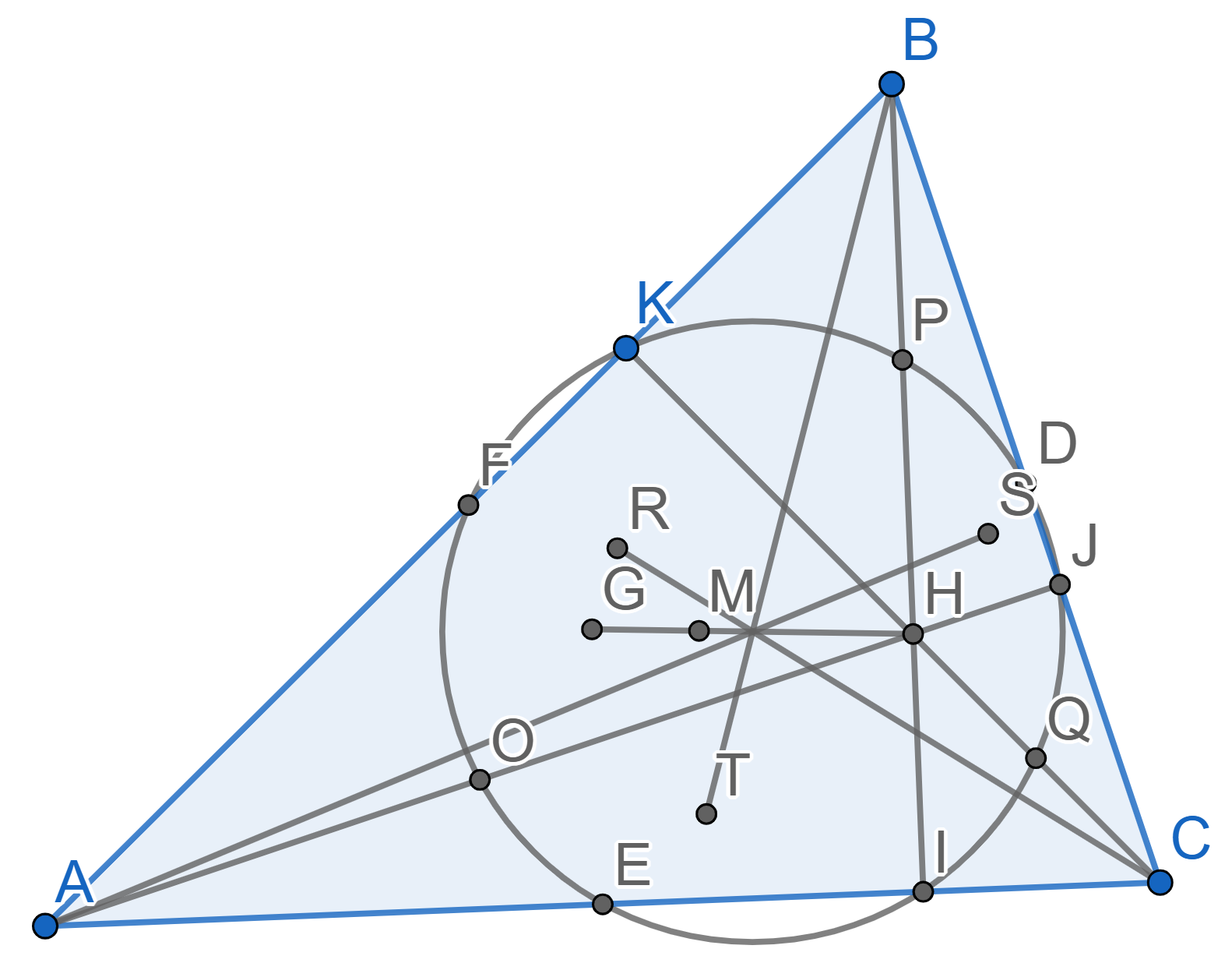
Consider the point \(H\) of intersection of the heights of the triangle \(ABC\). Prove that Euler lines of the triangles \(ABC\), \(ABH\), \(BCH\), \(ACH\) intersect at one point. On the diagram below the points \(R,S,T\) are the points of intersection of medians in triangles \(ABH\), \(BCH\), and \(ACH\) correspondingly.
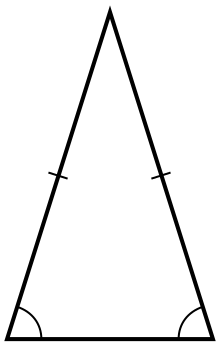
What are the symmetries of an isosceles triangle (which is not equilateral)?
What are the symmetries of the reduce-reuse-recycle symbol?
What are the symmetries of an equilateral triangle?
