Problems
The upper side of a piece of square paper is white, and the lower one is red. In the square, a point F is randomly chosen. Then the square is bent so that one randomly selected vertex overlaps the point F. Find the mathematical expectation of the number of sides of the red polygon that appears.
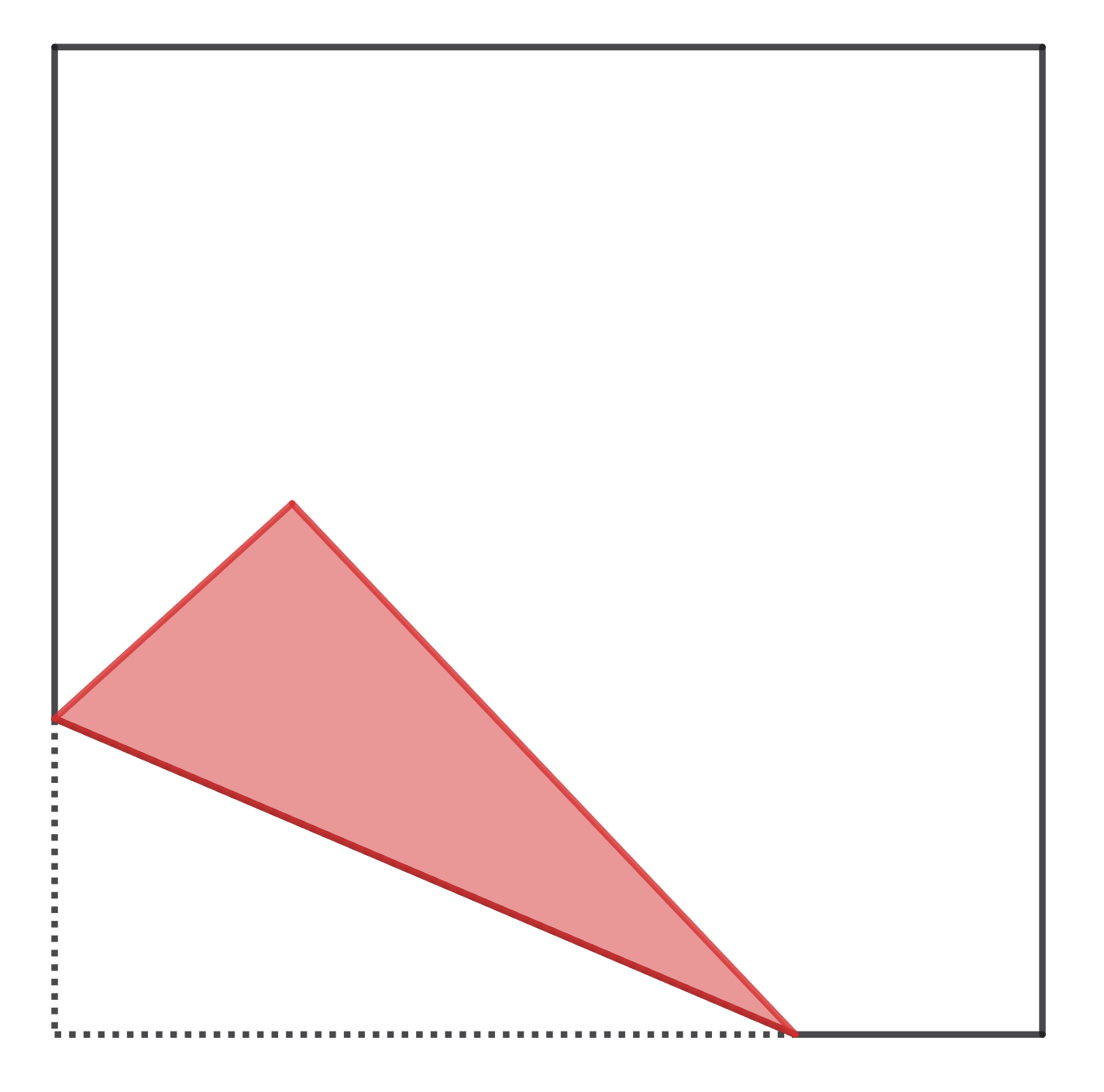
Ben is going to bend a square sheet of paper \(ABCD\). Ben calls the fold beautiful, if the side \(AB\) crosses the side \(CD\) and the four resulting rectangular triangles are equal. Before that, Jack selects a random point on the sheet \(F\). Find the probability that Ben will be able to make a beautiful fold through the point \(F\).
40% of adherents of some political party are women. 70% of the adherents of this party are townspeople. At the same time, 60% of the townspeople who support the party are men. Are the events “the adherent of the party is a townsperson” and “the adherent of party is a woman” independent?
The tower in the castle of King Arthur is crowned with a roof, which is a triangular pyramid, in which all flat angles at the top are straight. Three roof slopes are painted in different colours. The red roof slope is inclined to the horizontal at an angle \(\alpha\), and the blue one at an angle \(\beta\). Find the probability that a raindrop that fell vertically on the roof in a random place fell on the green area.
Author: A.V. Shapovalov
We call a triangle rational if all of its angles are measured by a rational number of degrees. We call a point inside the triangle rational if, when we join it by segments with vertices, we get three rational triangles. Prove that within any acute-angled rational triangle there are at least three distinct rational points.
Author: Shapovalov A.V.
Let \(A\) and \(B\) be two rectangles. From rectangles equal to \(A\), a rectangle similar to \(B\) was created.
Prove that from rectangles equal to \(B\), you can create a rectangle similar to \(A\).
a) There is an unlimited set of cards with the words “abc”, “bca”, “cab” written. Of these, the word written is determined according to this rule. For the initial word, any card can be selected, and then on each turn to the existing word, you can either add on a card to the left or to the right, or cut the word anywhere (between the letters) and put a card there. Is it possible to make a palindrome with this method?
b) There is an unlimited set of red cards with the words “abc”, “bca”, “cab” and blue cards with the words “cba”, “acb”, “bac”. Using them, according to the same rules, a palindrome was made. Is it true that the same number of red and blue cards were used?
Author: A. Glazyrin
In the coordinate space, all planes with the equations \(x \pm y \pm z = n\) (for all integers \(n\)) were carried out. They divided the space into tetrahedra and octahedra. Suppose that the point \((x_0, y_0, z_0)\) with rational coordinates does not lie in any plane. Prove that there is a positive integer \(k\) such that the point \((kx_0, ky_0, kz_0)\) lies strictly inside some octahedron from the partition.
In the triangle \(ABC\), the angle \(A\) is equal to \(40^{\circ}\). The triangle is randomly thrown onto a table. Find the probability that the vertex \(A\) lies east of the other two vertices.
At a factory known to us, we cut out metal disks with a diameter of 1 m. It is known that a disk with a diameter of exactly 1 m weighs exactly 100 kg. During manufacturing, a measurement error occurs, and therefore the standard deviation of the radius is 10 mm. Engineer Gavin believes that a stack of 100 disks on average will weigh 10,000 kg. By how much is the engineer Gavin wrong?