There are 25 children in a class. At random, two are chosen. The probability that both children will be boys is \(3/25\). How many girls are in the class?
Kate and Gina agreed to meet at the underground in the first hour of the afternoon. Kate comes to the meeting place between noon and one o’clock in the afternoon, waits for 10 minutes and then leaves. Gina does the same.
a) What is the probability that they will meet?
b) How will the probability of a meeting change if Gina decides to come earlier than half past twelve, and Kate still decides to come between noon and one o’clock?
c) How will the probability of a meeting change if Gina decides to come at an arbitrary time between 12:00 and 12:50, and Kate still comes between 12:00 and 13:00?
The length of the hypotenuse of a right-angled triangle is 3.
a) The Scattered Scientist calculated the dispersion of the lengths of the sides of this triangle and found that it equals 2. Was he wrong in the calculations?
b) What is the smallest standard deviation of the sides that a rectangular triangle can have? What are the lengths of its sides, adjacent to the right angle?
James furiously cuts a rectangular sheet of paper with scissors. Every second he cuts a random piece by an unsystematic rectilinear cut into two parts.
a) Find the mathematical expectation of the number of sides of a polygon (made from a piece of paper) that James randomly picks up after an hour of such work.
b) Solve the same problem if at first the piece of paper had the form of an arbitrary polygon.
The upper side of a piece of square paper is white, and the lower one is red. In the square, a point F is randomly chosen. Then the square is bent so that one randomly selected vertex overlaps the point F. Find the mathematical expectation of the number of sides of the red polygon that appears.
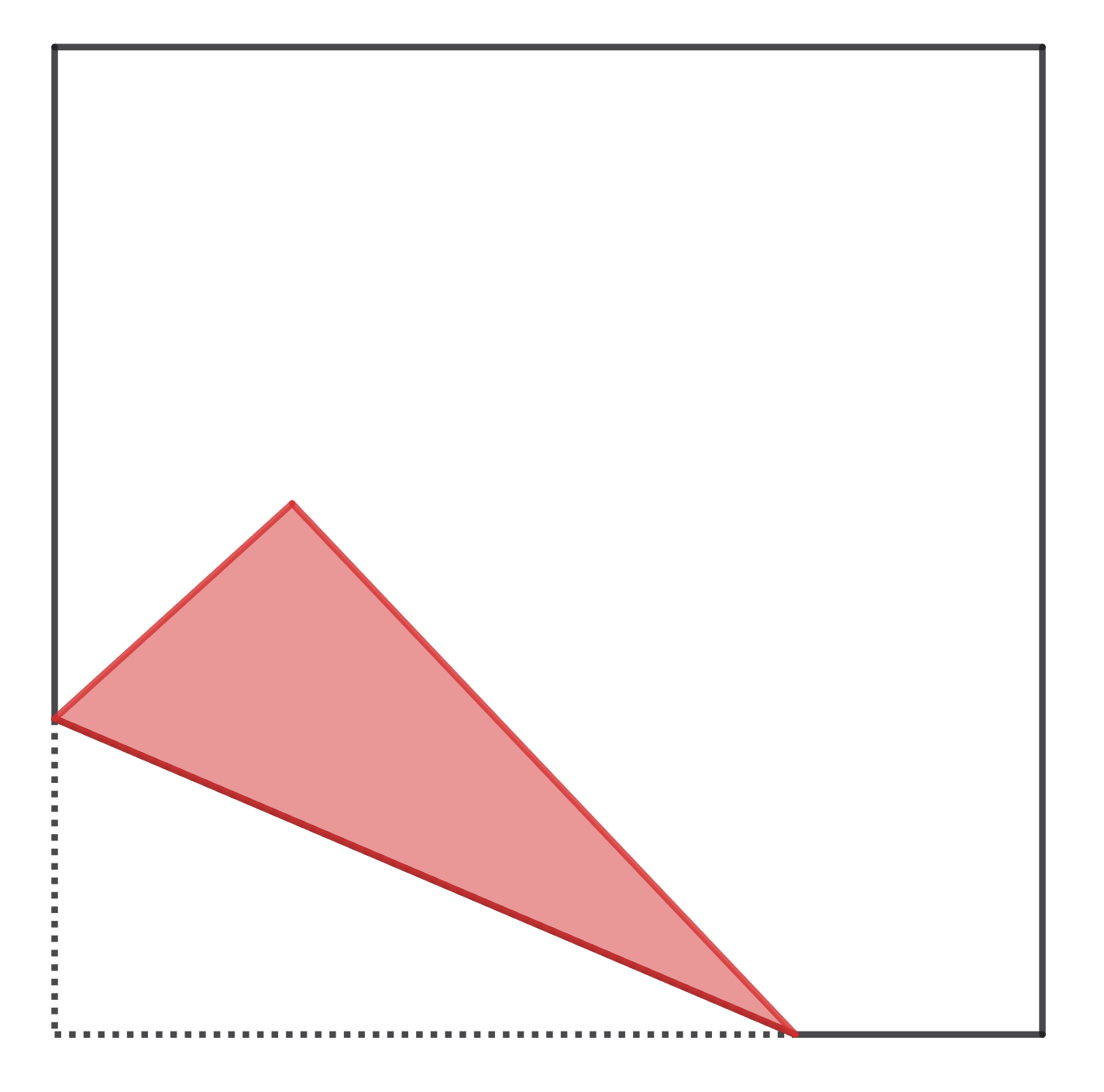
Ben is going to bend a square sheet of paper \(ABCD\). Ben calls the fold beautiful, if the side \(AB\) crosses the side \(CD\) and the four resulting rectangular triangles are equal. Before that, Jack selects a random point on the sheet \(F\). Find the probability that Ben will be able to make a beautiful fold through the point \(F\).
Author: A.V. Shapovalov
We call a triangle rational if all of its angles are measured by a rational number of degrees. We call a point inside the triangle rational if, when we join it by segments with vertices, we get three rational triangles. Prove that within any acute-angled rational triangle there are at least three distinct rational points.
a) There is an unlimited set of cards with the words “abc”, “bca”, “cab” written. Of these, the word written is determined according to this rule. For the initial word, any card can be selected, and then on each turn to the existing word, you can either add on a card to the left or to the right, or cut the word anywhere (between the letters) and put a card there. Is it possible to make a palindrome with this method?
b) There is an unlimited set of red cards with the words “abc”, “bca”, “cab” and blue cards with the words “cba”, “acb”, “bac”. Using them, according to the same rules, a palindrome was made. Is it true that the same number of red and blue cards were used?
In the triangle \(ABC\), the angle \(A\) is equal to \(40^{\circ}\). The triangle is randomly thrown onto a table. Find the probability that the vertex \(A\) lies east of the other two vertices.
At a factory known to us, we cut out metal disks with a diameter of 1 m. It is known that a disk with a diameter of exactly 1 m weighs exactly 100 kg. During manufacturing, a measurement error occurs, and therefore the standard deviation of the radius is 10 mm. Engineer Gavin believes that a stack of 100 disks on average will weigh 10,000 kg. By how much is the engineer Gavin wrong?