Problems
A series of squares are connected by touching vertices. Some triangles were drawn outside and inside of the chain. Show that the total green area is the same as the total red area.
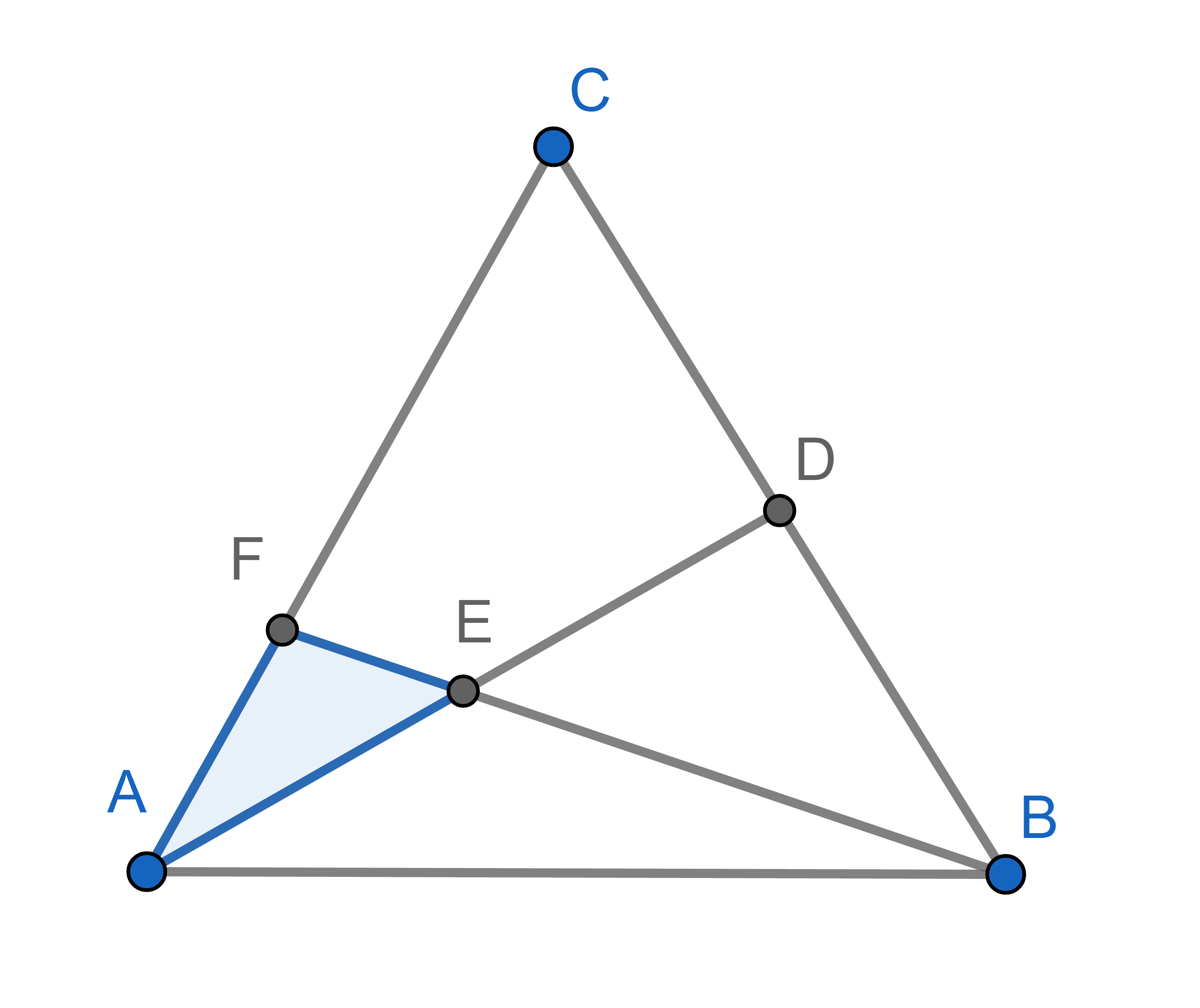
In a triangle \(\triangle ABC\), \(D\) is the midpoint of \(BC\), and \(E\) is the midpoint of \(AD\). \(F\) is the intersection of the side \(AC\) with \(BE\). What is the area of the triangle \(\triangle AEF\) as a proportion of the area of the triangle \(\triangle ABC\)?
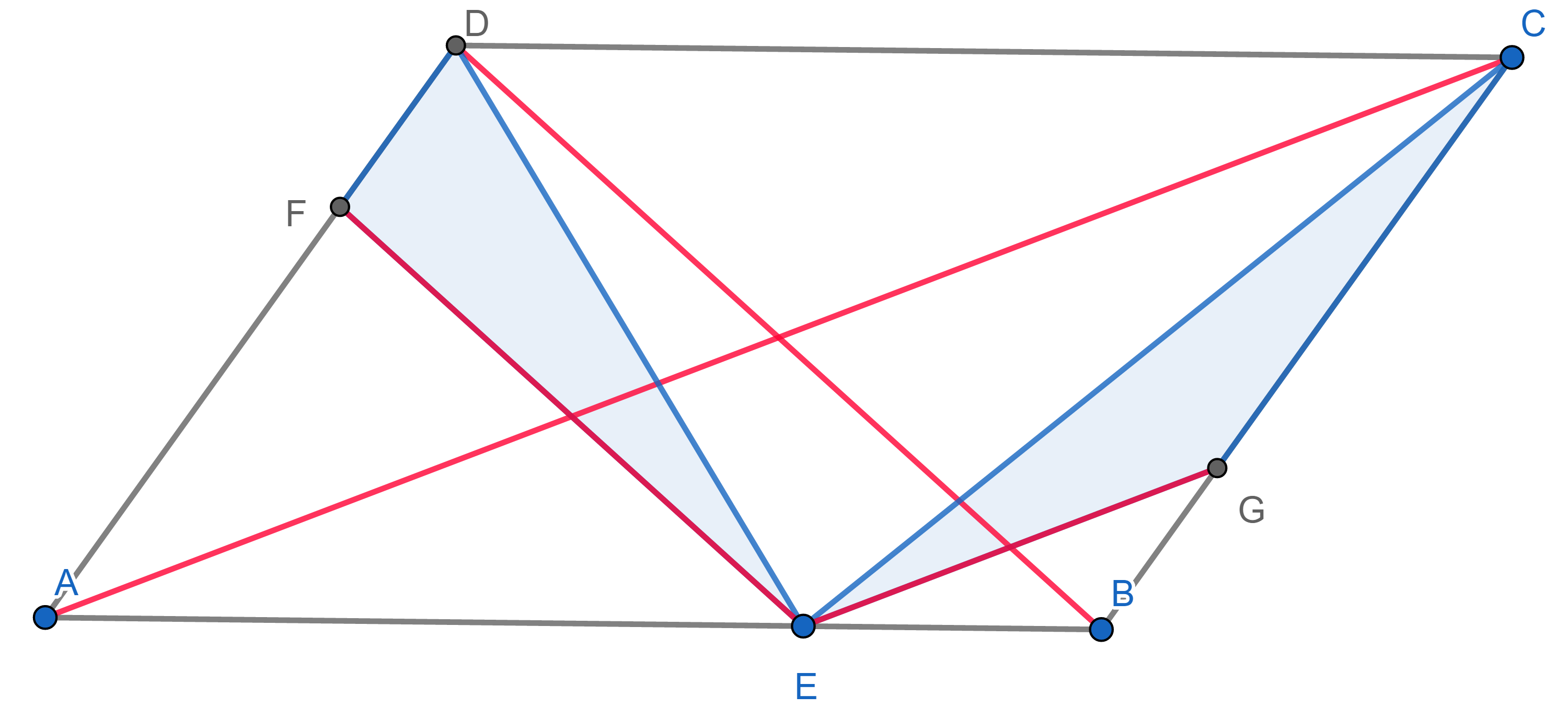
Let \(ABCD\) be a parallelogram. The segment \(EF\) is parallel to the diagonal \(BD\), and the segment \(EG\) is parallel to the diagonal \(AC\). Show that the areas of the triangles \(\triangle EFD\) and \(\triangle EGC\) are equal.
In a trapezium \(ABCD\), the side \(AB\) is parallel to the side \(CD\). Prove that the areas of triangles \(\triangle ABC\) and \(\triangle ABD\) are equal.

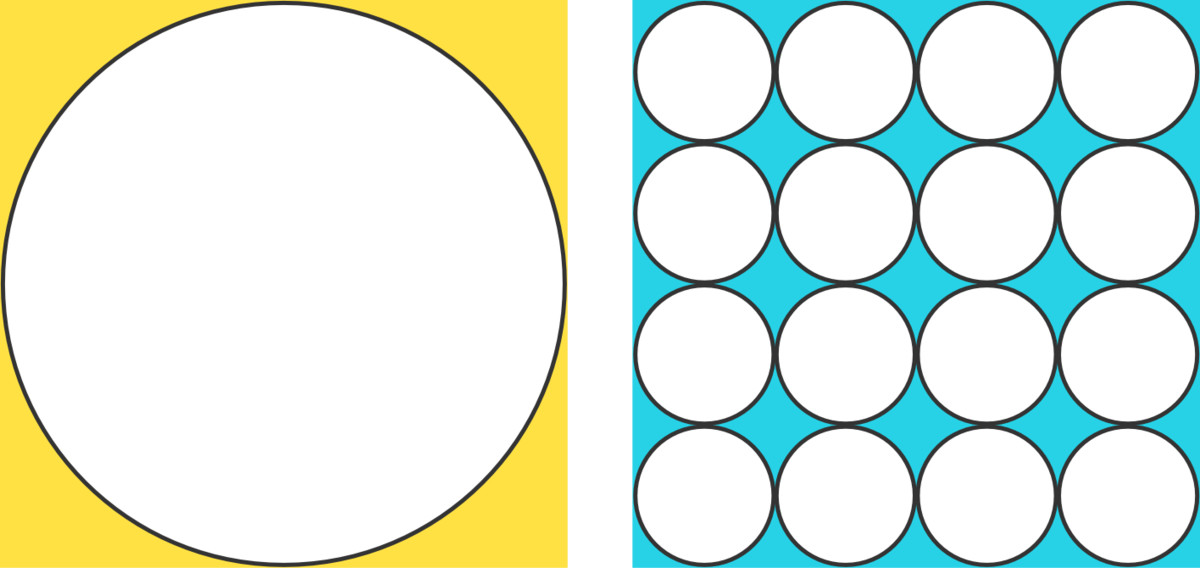
On the left there is a circle inscribed in a square with side \(1\). On the right there are \(16\) smaller, identical circles, which all together fit inside a square of side \(1\). Which area is greater, the yellow or the blue one?
A circle is inscribed in a square, and another square is inscribed in the circle. Which area is larger, the blue or the orange one?

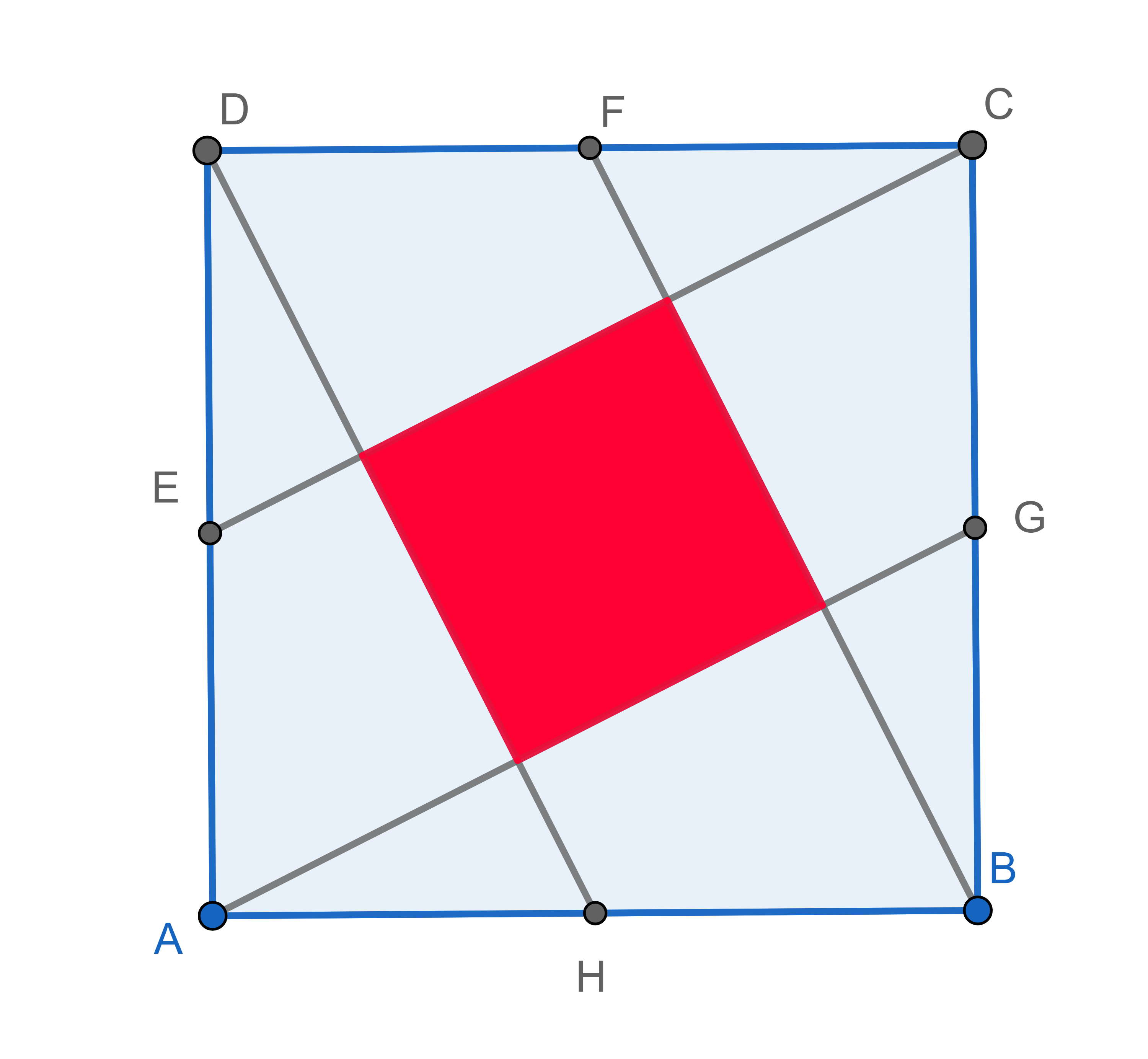
In a square, the midpoints of its sides were marked and connected to the vertices of the square. There is another square formed in the centre. The side length of the large square is \(10\). Find the area of the smaller square. (That is, the red one)
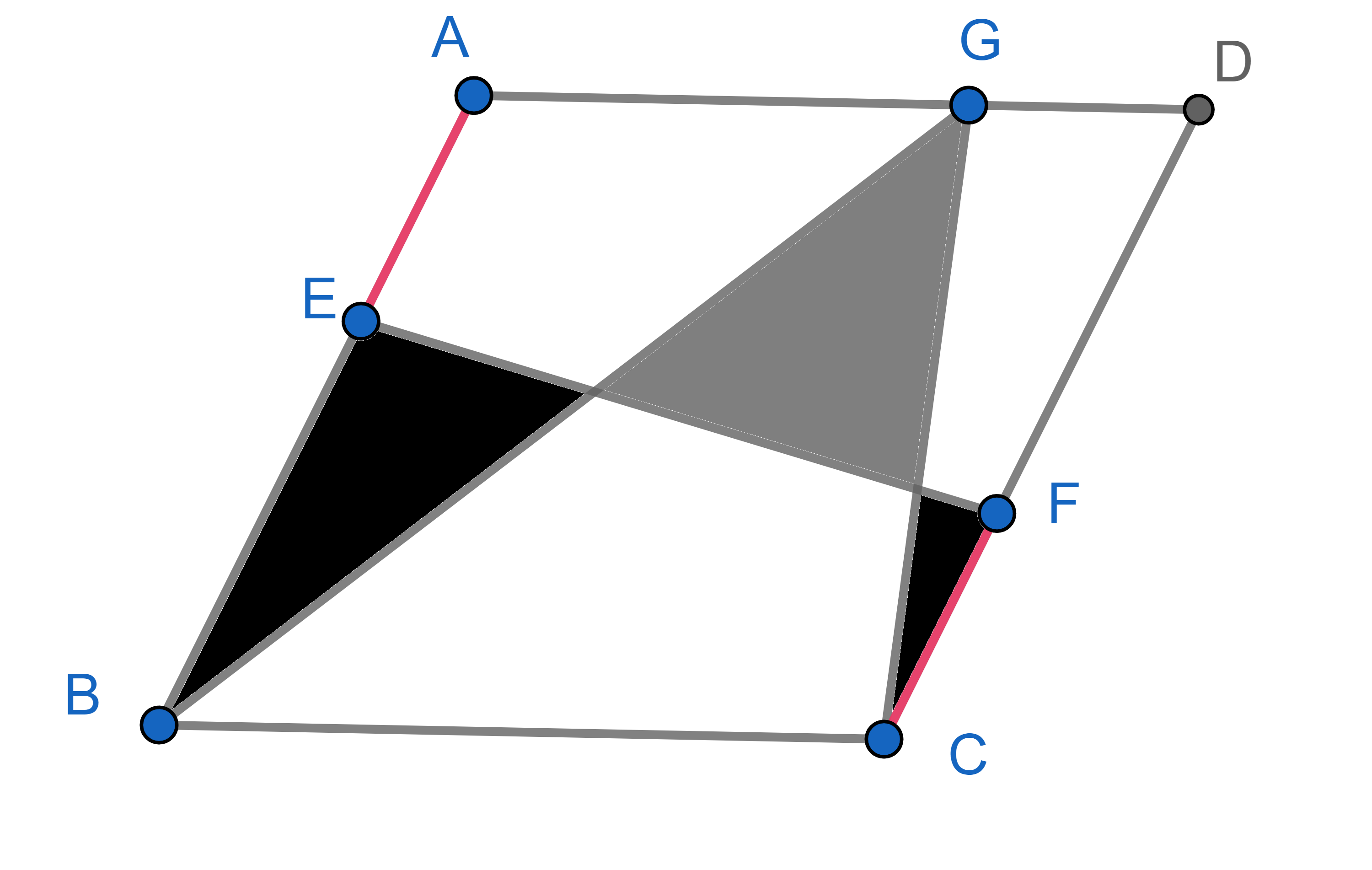
In a parallelogram \(ABCD\), point \(E\) belongs to the side \(AB\), point \(F\) belongs to the side \(CD\) and point \(G\) belongs to the side \(AD\). We know that the marked red segments \(AE\) and \(CF\) have equal lengths. Prove that the total grey area is equal to the total black area.
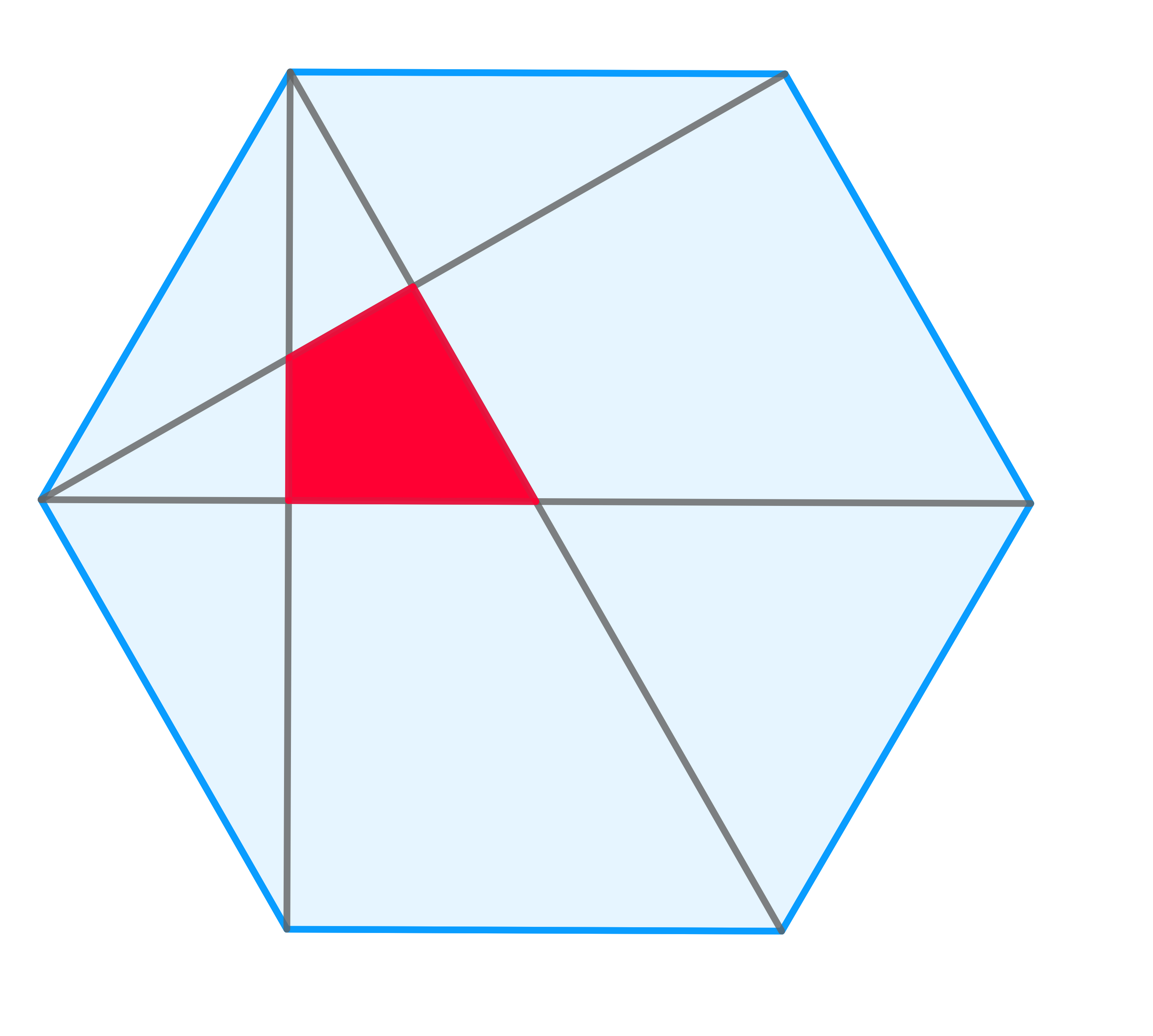
In a regular hexagon of area \(72\), some diagonals were drawn. Find the area of the red region.
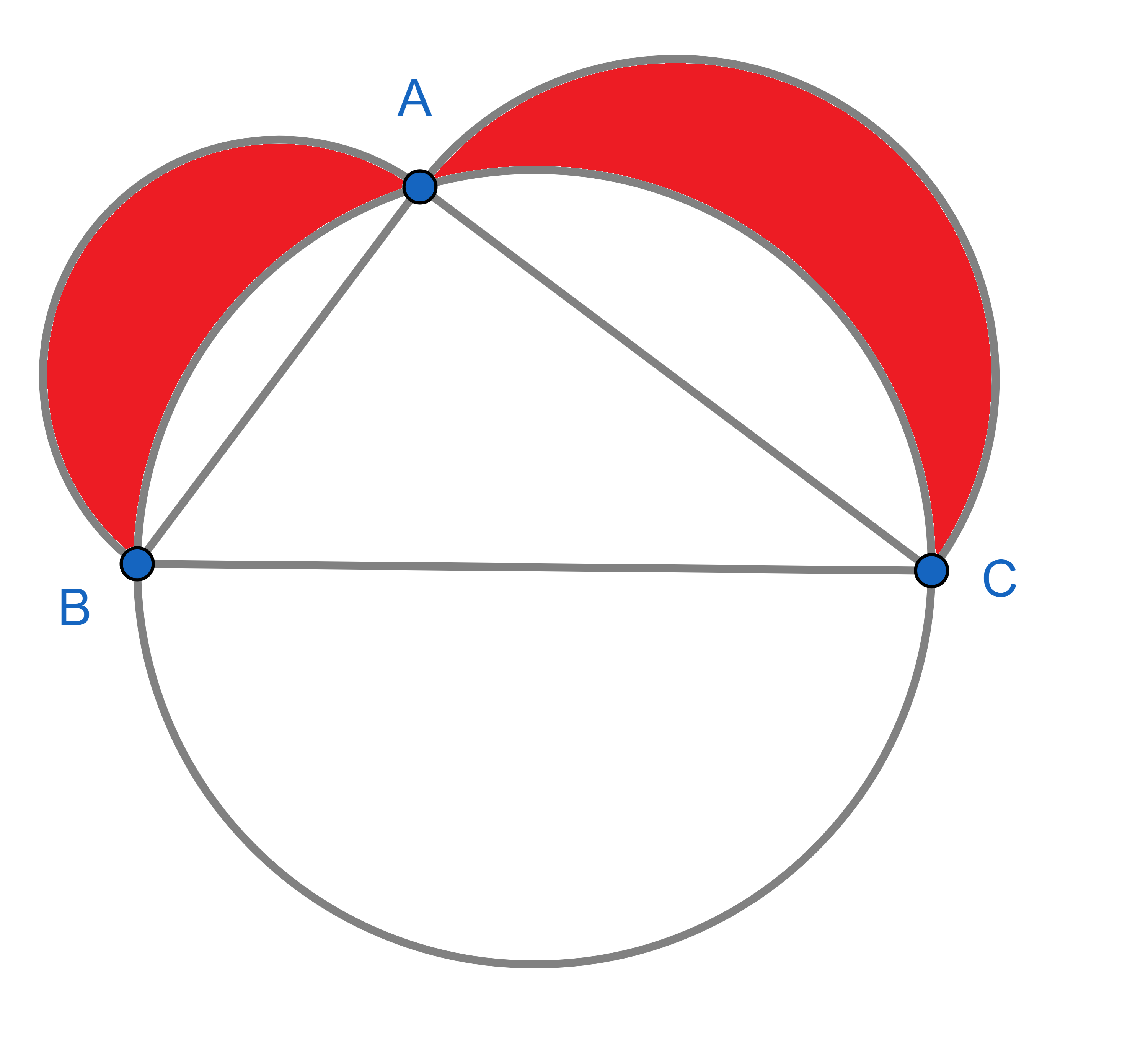
We have a triangle \(\triangle ABC\) with side lengths \(|AB|=3\), \(|AC|=4\) and angle \(\angle BAC=90^{\circ}\). A circle is drawn with side \(BC\) as diameter, and two semicircles are drawn with sides \(AB\) and \(AC\) as diameters. Find the area of the red part.